A solid metal ball has a spherical cavity. If the ball is heated, the volume of the cavity will
increase
decreases
remain uncharged
Data insufficient
The correct Answer is:A
To solve the problem, we need to analyze the effect of heating on a solid metal ball that contains a spherical cavity. Here’s a step-by-step breakdown of the solution:
Step 1: Understand the Structure
We have a solid metal ball with a spherical cavity inside it. Let’s denote the radius of the metal ball as r2 and the radius of the cavity as r1.
Step 2: Heating the Metal Ball
When the metal ball is heated, the temperature of the entire ball increases. As a result, the metal expands due to thermal expansion.
Step 3: Effect of Thermal Expansion
Thermal expansion affects all parts of the metal ball, including the cavity. The metal expands outward, which means that the radius of the metal ball r2 will increase.
Step 4: Impact on the Cavity
Since the cavity is part of the metal ball, as the metal expands, the cavity will also expand. This means that the radius of the cavity r1 will also increase.
Step 5: Volume Calculation
The volume V of a sphere is given by the formula:
V=43πr3
As the radius r1 of the cavity increases due to the expansion, the volume of the cavity will also increase.
Conclusion
Therefore, when the solid metal ball is heated, the volume of the cavity will increase.
Final Answer
The volume of the cavity will increase.
---
We have a solid metal ball with a spherical cavity inside it. Let’s denote the radius of the metal ball as r2 and the radius of the cavity as r1.
When the metal ball is heated, the temperature of the entire ball increases. As a result, the metal expands due to thermal expansion.
Thermal expansion affects all parts of the metal ball, including the cavity. The metal expands outward, which means that the radius of the metal ball r2 will increase.
Since the cavity is part of the metal ball, as the metal expands, the cavity will also expand. This means that the radius of the cavity r1 will also increase.
The volume V of a sphere is given by the formula:
V=43πr3
As the radius r1 of the cavity increases due to the expansion, the volume of the cavity will also increase.
Therefore, when the solid metal ball is heated, the volume of the cavity will increase.
The volume of the cavity will increase.
Topper's Solved these Questions
- THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESBook:DC PANDEY ENGLISHChapter:THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESExercise:Check point 14.3
Explore 20 Videos
- THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESBook:DC PANDEY ENGLISHChapter:THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESExercise:Check point 14.4
Explore 25 Videos
- THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESBook:DC PANDEY ENGLISHChapter:THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESExercise:Check point 14.1
Explore 10 Videos
- SUPERPOSITION OF WAVESBook:DC PANDEY ENGLISHChapter:SUPERPOSITION OF WAVESExercise:Level 2 Subjective
Explore 8 Videos
- THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASESBook:DC PANDEY ENGLISHChapter:THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASESExercise:Level 2 Subjective
Explore 9 Videos
Similar Questions
A solid ball of metal has a concentric spherical cavity within it. If the ball is heated, the volume of the cavity will
View SolutionA solid uniform ball of volume V floats on the interface of two immiscible liquids [The specific gravity of the upper liquid is γ/2 and that of the lower liquid is 2γ, where γ is th especific gracity of the solid ball.] The fraction of the volume of the ball that will be in upper liquid is
View SolutionA solid sphere of radius R has a spherical cavity of radius r as shown in the figure. If the volume charge density σ is uniformly distributed over the whole volume of the sphere, then electric field strength at the centre of the sphere will be
View SolutionA solid sphere of mass M and radius R has a spherical cavity of radius R/2 such that the centre of cavity is at a distance R/2 from the centre of the sphere. A point mass m is placed inside the cavity at a distanace R/4 from the centre of sphere. The gravitational force on mass m is
View SolutionA solid sphere of mass M and radius R has a spherical cavity of radius R/2 such that the centre of cavity is at a distance R/2 from the centre of the sphere. A point mass m is placed inside the cavity at a distance R/4 from the centre of sphere. The gravitational force on mass m is
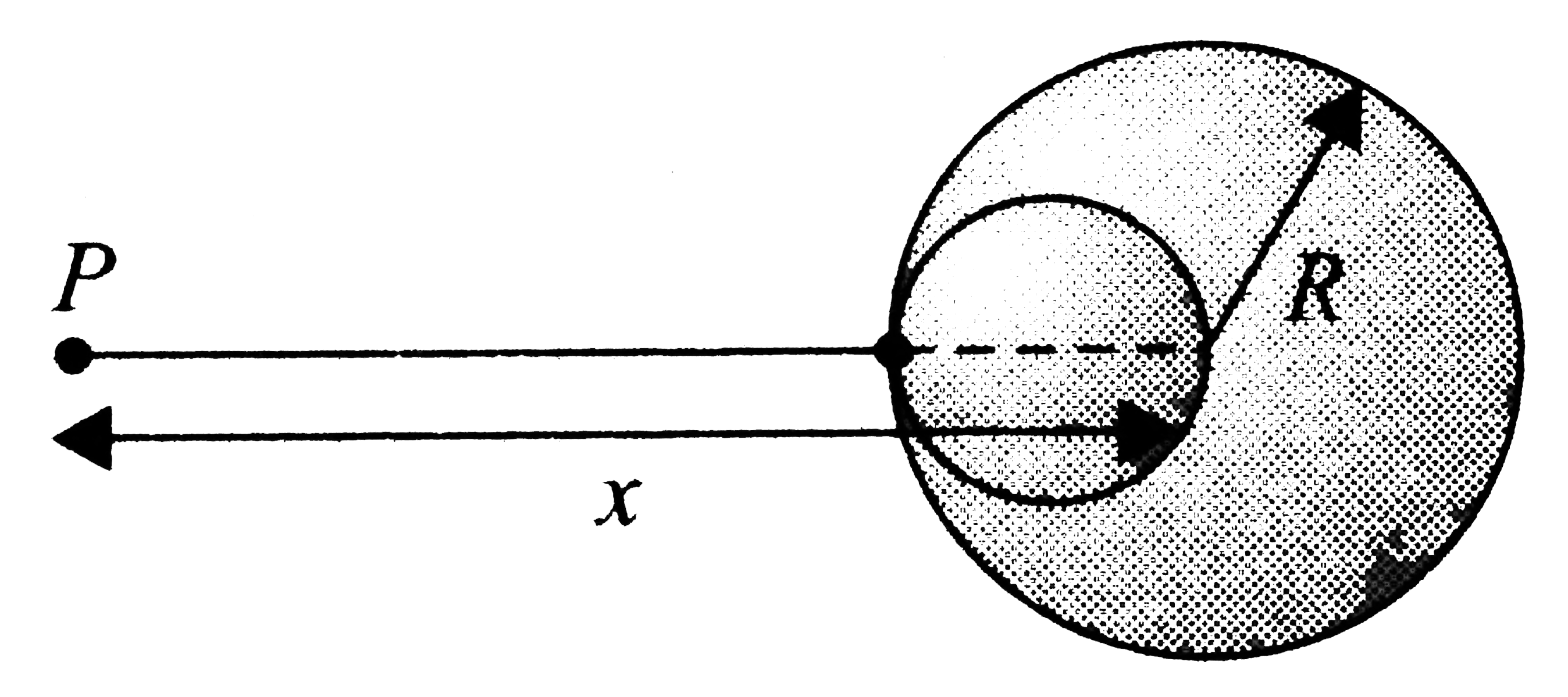
View SolutionA solid spherical region, having a spherical cavity whose diameter R is equal to the radius of the spherical region, has a total charge Q. Find the electric field at a point P as shown in figure
 .
.
View SolutionState True or False and justify your answer
A solid ball is exactly fitted inside the cubical box of side a. The volume of the ball is 43πa3.
View SolutionA sphere of radius R has a uniform volume density ρ. A spherical cavity of radius b, whose center lies at →a, is removed from the sphere.
i. Find the electric field at any point inside the spherical cavity.
ii. Find the electric field outside the cavity
(a) at points inside the large sphere but outside the cavity and
(b) at points outside the large sphere.

View SolutionAssertion :− The anatomical setup of lungs in thorax is such that any change in the volume of the thoracic cavity will be reflected in the lung cavity. (Pulmonary cavity )
Reason :− Lungs have no muscles so we cannot directly alter the pulmonary volume
View SolutionA cylindrical rod whose height is 8 times of its radius is melted and recast into spherical balls of same radius. The number of the balls will be
View Solution