The velocity v and displacement x of a particle executing simple harmonic motion are related as
vdvdx=−ω2x.
Atx=0,v=v0. Find the velocity v when the displacement becomes x.
√v20+ω2x2
√v20−ω2x2
v=3√v30+ω2x3
v=v0−(ω3x3ex3)1/3
The correct Answer is:B
To solve the problem, we will follow these steps:
Step 1: Start with the given equation
The relationship between velocity v and displacement x for a particle in simple harmonic motion is given by:
vdvdx=−ω2x
Step 2: Rearrange the equation
We can rearrange the equation to separate variables:
vdv=−ω2xdx
Step 3: Integrate both sides
Now, we will integrate both sides. The left side integrates with respect to v and the right side with respect to x:
∫vdv=∫−ω2xdx
Step 4: Perform the integration
The integrals yield:
v22=−ω2x22+C
where C is the constant of integration.
Step 5: Determine the constant of integration
We know that at x=0, v=v0. Plugging these values into the equation gives:
v202=C
Thus, the equation becomes:
v22=−ω2x22+v202
Step 6: Simplify the equation
Multiplying through by 2 to eliminate the fractions:
v2=v20−ω2x2
Step 7: Solve for v
Taking the square root of both sides, we find:
v=√v20−ω2x2
Final Result
The velocity v when the displacement is x is given by:
v=√v20−ω2x2
---
The relationship between velocity v and displacement x for a particle in simple harmonic motion is given by:
vdvdx=−ω2x
We can rearrange the equation to separate variables:
vdv=−ω2xdx
Now, we will integrate both sides. The left side integrates with respect to v and the right side with respect to x:
∫vdv=∫−ω2xdx
The integrals yield:
v22=−ω2x22+C
where C is the constant of integration.
We know that at x=0, v=v0. Plugging these values into the equation gives:
v202=C
Thus, the equation becomes:
v22=−ω2x22+v202
Multiplying through by 2 to eliminate the fractions:
v2=v20−ω2x2
Taking the square root of both sides, we find:
v=√v20−ω2x2
The velocity v when the displacement is x is given by:
v=√v20−ω2x2
Similar Questions
Knowledge Check
A particle executing simple harmonic motion with an amplitude 5 cm and a time period 0.2 s. the velocity and acceleration of the particle when the displacement is 5 cm is
A0.5πms−1,0ms−2B0.5ms−1,−5π2ms−2C0ms−1,−5π2ms−2D0.5πms−1,−0.5π2ms−2Which of the following relationships between the acceleration a and the displacement x of a particle executing simple harmonic motion?
Aa=2x2Ba=−2x2Ca=2xDa=−2x
The acceleration-displacement (a−X) graph of a particle executing simple harmonic motion is shown in the figure. Find the frequency of oscillation.

View SolutionObtain the formulae for displacement, velocity, acceleration and time period of a particle executing simple harmonic motion. When is the velocity of particle maximum and when zero ? Acceleration ?
View SolutionThe acceleration (a) of a particle depends on displacement (s) as a=5+s . Initially s=0,v=5, then velocity v corresponding to the displacement is given by
View SolutionIf a graph is plotted between velocity (v) and displacement (y) of a particle executing SHM from mean position, then the nature of the graph is
View SolutionFor a particle undergoing simple harmonic motion, the velocity is plotted against displacement. The curve will be
View SolutionThe velocity-displacement graph of a particle is shown in Fig . (a) Write the relation between (v) and (x).
(b) Obtain the relation between acceleration and displacement and plot it .
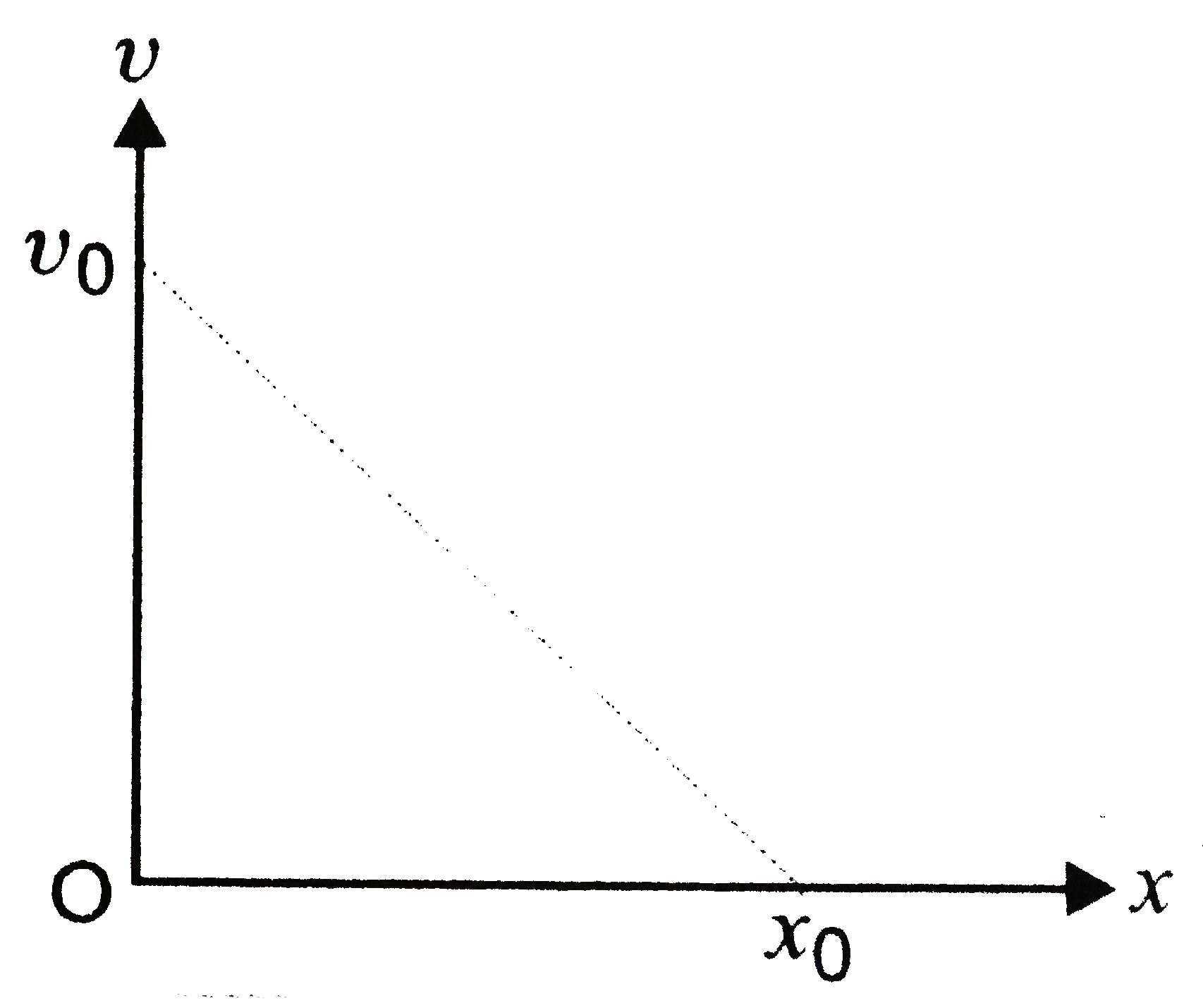 .
.
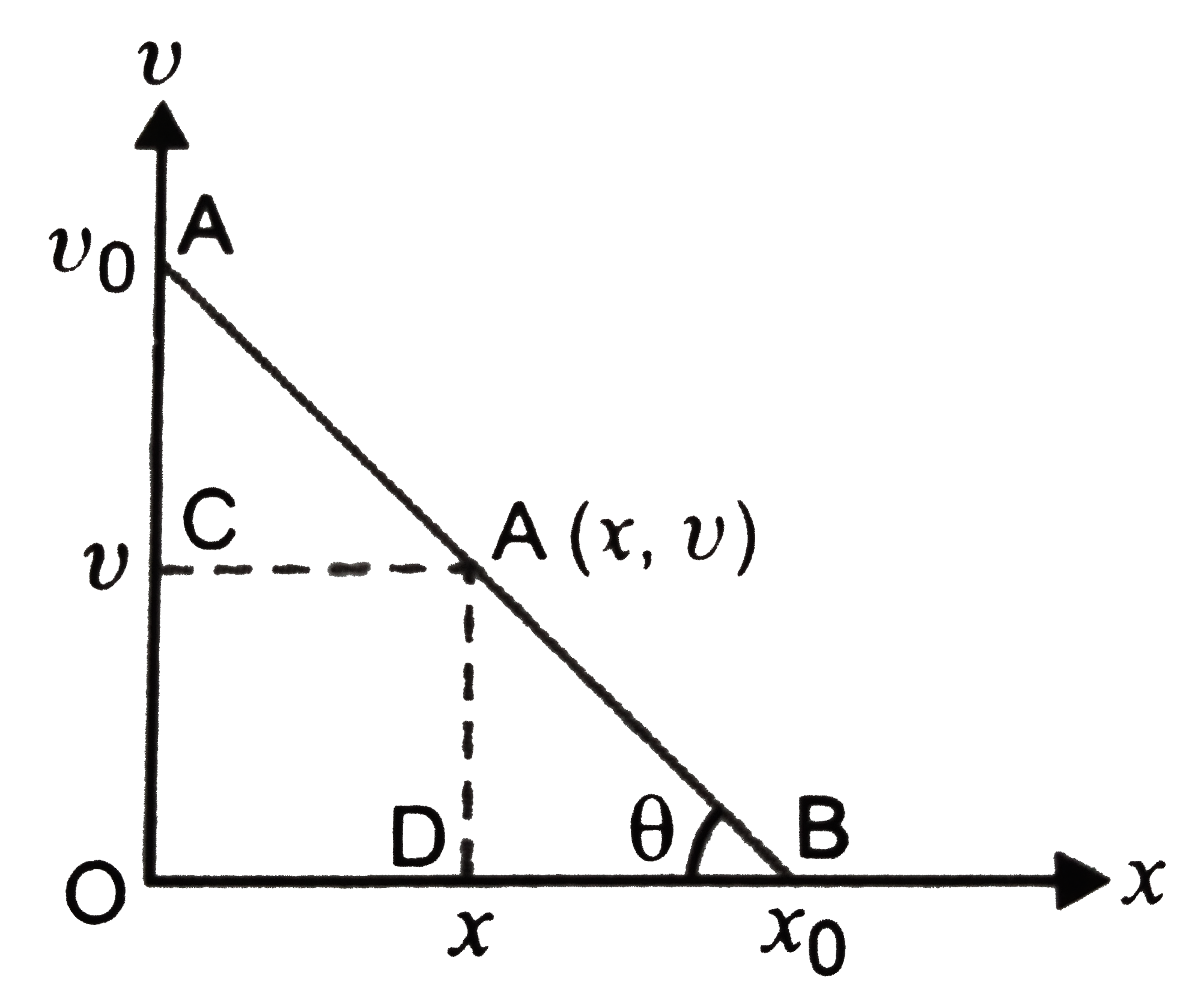 .
.
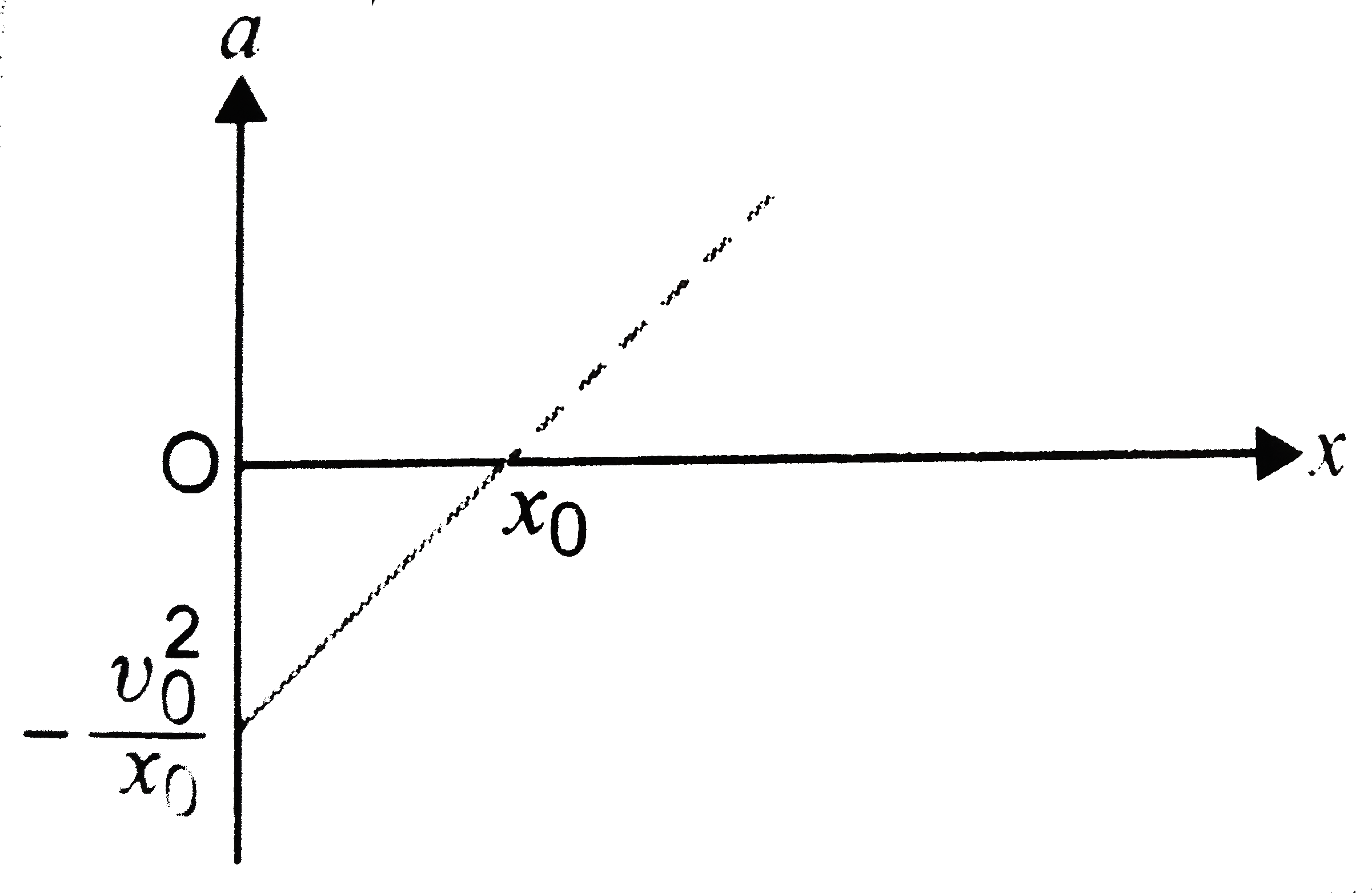 .
.
View Solution


