Assertion : x-t equation of a particle in SHM is given as :x=Acosωt
Reason : In the given equation the minimum potential energy is zero.
If both Assertion and Reason are correct and Reason is the correct explanation of Assertion.
If both Assertion and Reason are correct but Reason is not the correct explanation of Assertion.
If Assertion is true but Reason is false.
If Assertion is false but Reason is true.
The correct Answer is:C
To solve the given question, we need to analyze both the assertion and the reason provided.
1. Understanding the Assertion:
- The assertion states that the displacement-time equation of a particle in Simple Harmonic Motion (SHM) is given by x=Acos(ωt).
- This equation is indeed a standard form of the equation for SHM, where:
- x is the displacement,
- A is the amplitude (maximum displacement),
- ω is the angular frequency,
- t is the time.
- At t=0, the displacement x equals A, which means the particle starts from the maximum displacement (amplitude).
2. Understanding the Reason:
- The reason states that in the given equation, the minimum potential energy is zero.
- In SHM, potential energy (PE) is related to the displacement from the equilibrium position. The potential energy can be expressed as:
PE=12kx2
where k is the spring constant.
- The potential energy is minimum when the displacement x=0 (at the equilibrium position), and it is at this point that the potential energy is zero.
- However, the statement that "the minimum potential energy is zero" depends on the reference point chosen for potential energy. In many cases, we take the equilibrium position as the reference point where potential energy is zero. Thus, the statement can be misleading.
3. Conclusion:
- The assertion is true because x=Acos(ωt) is indeed the correct equation for SHM.
- The reason, however, is false because while the minimum potential energy can be zero, it is not universally true for all reference points.
Final Answer:
- Assertion: True
- Reason: False
- The assertion states that the displacement-time equation of a particle in Simple Harmonic Motion (SHM) is given by x=Acos(ωt).
- This equation is indeed a standard form of the equation for SHM, where:
- x is the displacement,
- A is the amplitude (maximum displacement),
- ω is the angular frequency,
- t is the time.
- At t=0, the displacement x equals A, which means the particle starts from the maximum displacement (amplitude).
- The reason states that in the given equation, the minimum potential energy is zero.
- In SHM, potential energy (PE) is related to the displacement from the equilibrium position. The potential energy can be expressed as:
PE=12kx2
where k is the spring constant.
- The potential energy is minimum when the displacement x=0 (at the equilibrium position), and it is at this point that the potential energy is zero.
- However, the statement that "the minimum potential energy is zero" depends on the reference point chosen for potential energy. In many cases, we take the equilibrium position as the reference point where potential energy is zero. Thus, the statement can be misleading.
- The assertion is true because x=Acos(ωt) is indeed the correct equation for SHM.
- The reason, however, is false because while the minimum potential energy can be zero, it is not universally true for all reference points.
- Assertion: True
- Reason: False
Similar Questions
x-t equation of a particle moving along x-axis is given as
x=A+A(1−cosωt)
View SolutionThe motion of a particle is given by x=Asinωt+Bcosωt. The motion of the particle is
View Solutionx-t equation of a particle in SHM is given as x=1.0sin(12πt) in SI units. Potential energy at mean position is zero. Mass of particle is 14 kg. Match the following table (SI units).
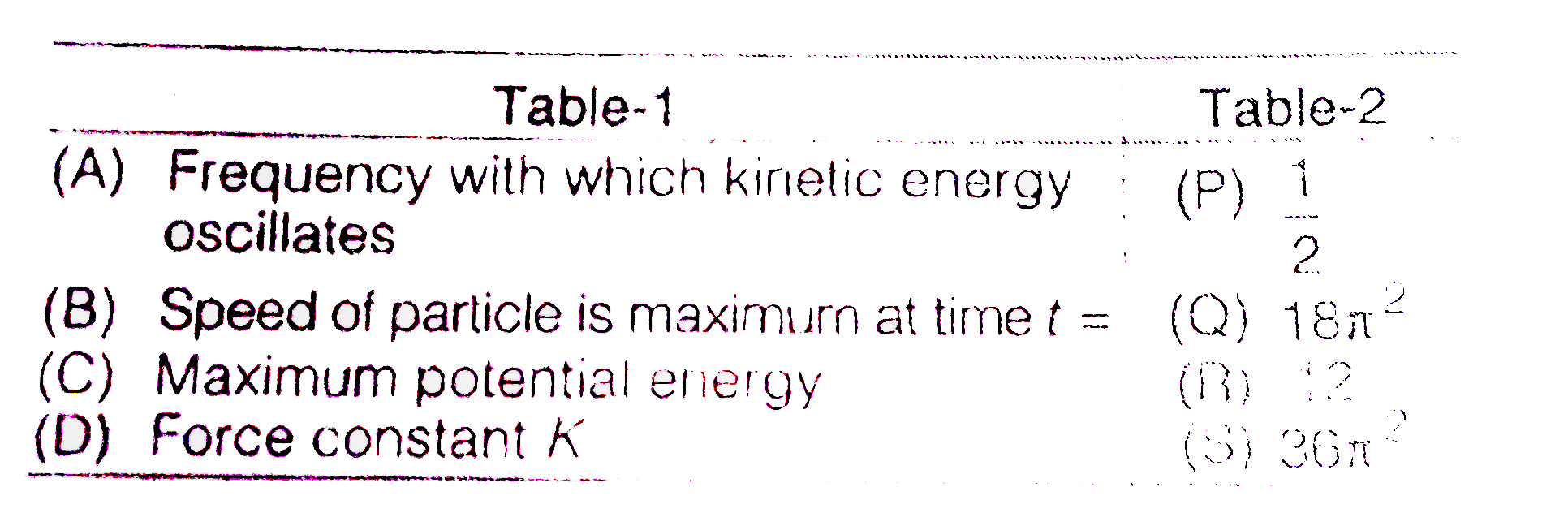
View SolutionThe position of a particle moving along x- axis is given by x=x0cos2(ωt) . Its when it is at mean position is
View SolutionAssertion : Time period of a spring-block equation of a particle moving along X-axis is x=4+6sinωt . Under this situation, motion of particle is not simple harmonic.
Reason : d2xdt2 for the given equation is proportional to -x.
View SolutionFor a particle executing SHM, the displacement x is given by x=Acosωt. Identify the graph which represents the variation of potential energy (PE) as a function of time t and displacement x.

(a) I,III
(b) II,IV (c ) II,III
(d) I,IV
View SolutionAssertion : We know the relation a=v.dvds . Therefore, if velocity of a particle is zero, then acceleration is also zero.
Reason : In the above equation, a is the instantaneous acceleration.
View Solutionx−t equation of a particle executing SHM is
x=Acos(ωt−45∘)
Find the point from where particle starts its journey and the direction of its initial velocity.
View SolutionAssertion :Mean positon of SHM is the stable equlibrium position.
Reason: In stable equilibrium , position potential energy is minimum.
View SolutionAssertion : In x=Acosωt, x is the displacement measured from extreme position.
Reason : In the above equation x=A at time t=0.
View Solution
