The energy at the mean position of a pendulum will be
zero
partial PE and partial KE
totally KE
totally PE
The correct Answer is:C
To determine the energy at the mean position of a pendulum, we can analyze the kinetic and potential energy involved in simple harmonic motion (SHM). Here’s a step-by-step solution:
Step 1: Understand the Energy Forms in SHM
In simple harmonic motion, the total mechanical energy (E) of the system is the sum of kinetic energy (KE) and potential energy (PE). The formulas for these energies are:
- Kinetic Energy (KE) = 12mω2(A2−x2)
- Potential Energy (PE) = 12mω2x2
Where:
- m = mass of the pendulum bob
- ω = angular frequency
- A = amplitude of the motion
- x = displacement from the mean position
Step 2: Identify the Mean Position
The mean position of the pendulum corresponds to the point where the displacement x=0.
Step 3: Calculate Kinetic Energy at Mean Position
Substituting x=0 into the kinetic energy formula:
KE=12mω2(A2−02)=12mω2A2
Step 4: Calculate Potential Energy at Mean Position
Substituting x=0 into the potential energy formula:
PE=12mω2(02)=0
Step 5: Determine Total Energy at Mean Position
The total energy at the mean position is entirely kinetic since the potential energy is zero:
E=KE+PE=12mω2A2+0=12mω2A2
Conclusion
At the mean position of a pendulum, the energy is entirely kinetic. Therefore, the answer to the question is that the energy at the mean position of a pendulum will be totally kinetic energy.
Final Answer
The energy at the mean position of a pendulum will be totally kinetic energy.
---
In simple harmonic motion, the total mechanical energy (E) of the system is the sum of kinetic energy (KE) and potential energy (PE). The formulas for these energies are:
- Kinetic Energy (KE) = 12mω2(A2−x2)
- Potential Energy (PE) = 12mω2x2
- m = mass of the pendulum bob
- ω = angular frequency
- A = amplitude of the motion
- x = displacement from the mean position
The mean position of the pendulum corresponds to the point where the displacement x=0.
Substituting x=0 into the kinetic energy formula:
KE=12mω2(A2−02)=12mω2A2
Substituting x=0 into the potential energy formula:
PE=12mω2(02)=0
The total energy at the mean position is entirely kinetic since the potential energy is zero:
E=KE+PE=12mω2A2+0=12mω2A2
At the mean position of a pendulum, the energy is entirely kinetic. Therefore, the answer to the question is that the energy at the mean position of a pendulum will be totally kinetic energy.
The energy at the mean position of a pendulum will be totally kinetic energy.
Similar Questions
A simple pendulum is oscillating in a trolley moving on a horizontal straight road with constant acceleration a. If direction of motion of trolley is taken as positive x direction and vertical upward direction as positive y direction then the mean position of pendulum makes an angle
View SolutionRatio of kinetic energy at mean position to potential energy at A/2 of a particle performing SHM
View SolutionExplain the conversion of energy in the oscillation of a simple pendulum using a diagram.
View SolutionA particle executes shm of period.8 s. After what time of its passing through the mean position the energy will be half kinetic and half potential.
View SolutionA linear harmonic oscillator has a total mechanical energy of 200J. Potential energy of it at mean position is 50J. Find
(i) the maximum kinetic energy,
(ii)the minimum potential energy,
(iii) the potential energy at extreme positions.
View SolutionA linear harmonic oscillator has a total mechanical energy of 200J. Potential energy of it at mean position is 50J. Find
(i) the maximum kinetic energy,
(ii)the minimum potential energy,
(iii) the potential energy at extreme positions.
View SolutionA particle is executing simple harmonic motion in a conservative force field. The total energy of simple harmonic motion is given by E=ax2+bv2where ‘x’ is the displacement from mean position x = 0 and v is the velocity of the particle at x then choose the INCORRECT statements.{Potential energy at mean position is assumed to be zero}
View SolutionThe total energy of a particle in SHM is E. Its kinetic energy at half the amplitude from mean position will be
View SolutionStatement-1: kinetic energy of SHM at mean position is equal to potential energy at ends for a particle moving in SHM.
Statement-2: Total energy in SHM is conserved.
View SolutionPotential energy of a particle at mean position is 4 J and at extreme position is 20 J. Given that amplitude of oscillation is A. Match the following two columns
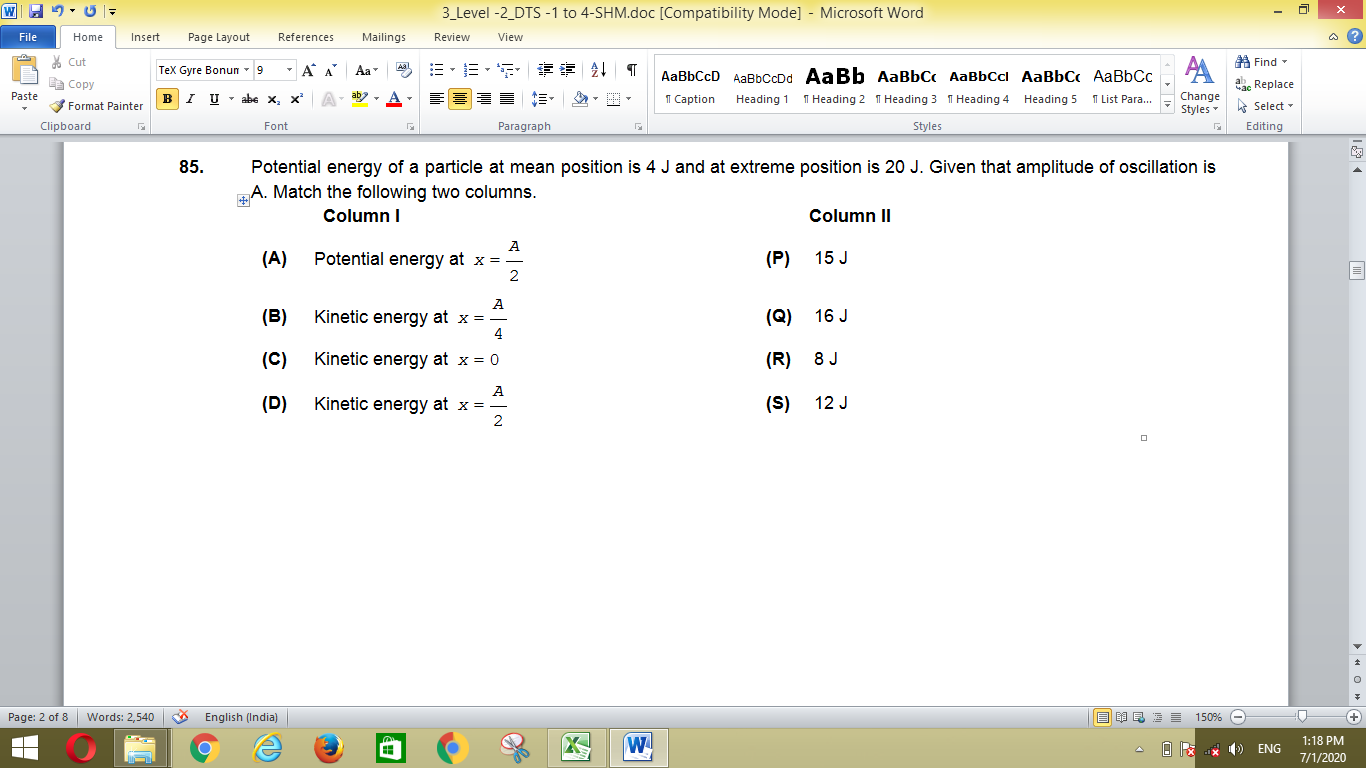
View Solution
