Assertion (A+B).(A-B) is always positve.
Reason this is positive If |A|>|B|
If both Assetion and Reason are correct but Reason is the correct explanation of Assertion.
If both Assetion and Reason are correct but Reason is not the correct explanation of Assertion.
If Asserion is true but Reason is false
If Asserion is false but Reason is true
The correct Answer is:D
To solve the question, we need to analyze both the assertion and the reason provided.
Step 1: Understanding the Assertion
The assertion states that (A+B)⋅(A−B) is always positive. We need to evaluate this expression.
Step 2: Expanding the Dot Product
Using the distributive property of the dot product, we can expand the expression:
(A+B)⋅(A−B)=A⋅A−A⋅B+B⋅A−B⋅B
Since the dot product is commutative (A⋅B=B⋅A), we can simplify this to:
A⋅A−B⋅B=|A|2−|B|2
Step 3: Analyzing the Result
The expression |A|2−|B|2 can be positive, negative, or zero depending on the magnitudes of A and B:
- If |A|>|B|, then |A|2−|B|2>0.
- If |A|<|B|, then |A|2−|B|2<0.
- If |A|=|B|, then |A|2−|B|2=0.
Thus, the assertion that (A+B)⋅(A−B) is always positive is false.
Step 4: Understanding the Reason
The reason states that the expression is positive if |A|>|B|. We have already established that if |A|>|B|, then (A+B)⋅(A−B)=|A|2−|B|2>0. This is indeed true.
Conclusion
- The assertion is false because (A+B)⋅(A−B) is not always positive.
- The reason is true because the expression is positive when |A|>|B|.
Final Answer
The answer is: Assertion is false, but reason is true.
---
The assertion states that (A+B)⋅(A−B) is always positive. We need to evaluate this expression.
Using the distributive property of the dot product, we can expand the expression:
(A+B)⋅(A−B)=A⋅A−A⋅B+B⋅A−B⋅B
Since the dot product is commutative (A⋅B=B⋅A), we can simplify this to:
A⋅A−B⋅B=|A|2−|B|2
The expression |A|2−|B|2 can be positive, negative, or zero depending on the magnitudes of A and B:
- If |A|>|B|, then |A|2−|B|2>0.
- If |A|<|B|, then |A|2−|B|2<0.
- If |A|=|B|, then |A|2−|B|2=0.
The reason states that the expression is positive if |A|>|B|. We have already established that if |A|>|B|, then (A+B)⋅(A−B)=|A|2−|B|2>0. This is indeed true.
- The assertion is false because (A+B)⋅(A−B) is not always positive.
- The reason is true because the expression is positive when |A|>|B|.
The answer is: Assertion is false, but reason is true.
Topper's Solved these Questions
Similar Questions
In a triangle ABC √a+√b−√c is (A) always positive (B) always negative (C) positive only when c is smallest (D) none of these
View SolutionAssertion (A) Glycine must be taken through diet.
Reason (R) It is an essential amino acid.
(a)Assertion and reason both are correct statements and reason explains the assertion.
(b)Both assertion and reason are wrong statements
(c)Assertion is correct statement and reason is wrong statement.
(d)Assertion is wrong statement and reason is correct statement.
View Solution
Knowledge Check
Assertion : If A and B are square matrices of order 2, then AB = BA is not always true.
Reason : Matrix multiplication is associative.ABoth assertion and reason are correct and reason is the correct explanation of assertion.BBoth assertion and reason are correct but reason is not the correct explanation of assertion.CAssertion is correct but reason is notcorrect.DAssertion is incorrect but reason is correct.Assertion: Force on a body A by body B is equal and opposite to the force on the body B by A .
Reason: Force in nature always occur between pairs of bodies.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true and reason is the not correct explanation of assertion.CIf assertion is true but reason is falseDIf both assertion and reason are false.Assertion: Drosophila melanogaster is widely used in genetic research.
Reason: Drosophila melanogaster is a readily available insect. a) If both Assertion and Reason are true and the Reason is the correct explanation of the Assertion. b) If both Assertion and Reason are true and the Reason is not the correct explanation of the Assertion. c) If Assertion is true but the Reason is false. d) If both Assertion and Reason are false.AIf both Assertion and Reason are true and the Reason is the correct explanation of the Assertion.BIf both Assertion and Reason are true and the Reason is not the correct explanation of the Assertion.CIf Assertion is true but the Reason is false.DIf both Assertion and Reason are false.
Assertion (A) D(+)− Glucose is dextrorotatory in nature.
Reason (R) 'D' represents its dextrorotatory nature.
(a)Assertion and reason both are correct statements and reason explains the assertion.
(b)Both assertion and reason are wrong statements
(c)Assertion is correct statement and reason is wrong statement
(d)Assertion is wrong statement and reason is correct statement.
View Solutionp : if two integers a and b are such that a>b then a - b is always a positive integer.
q : If two integers a and b are such that a - b is always a positive integer, then a>b
Which of the following is true regarding statements p and q?
View Solution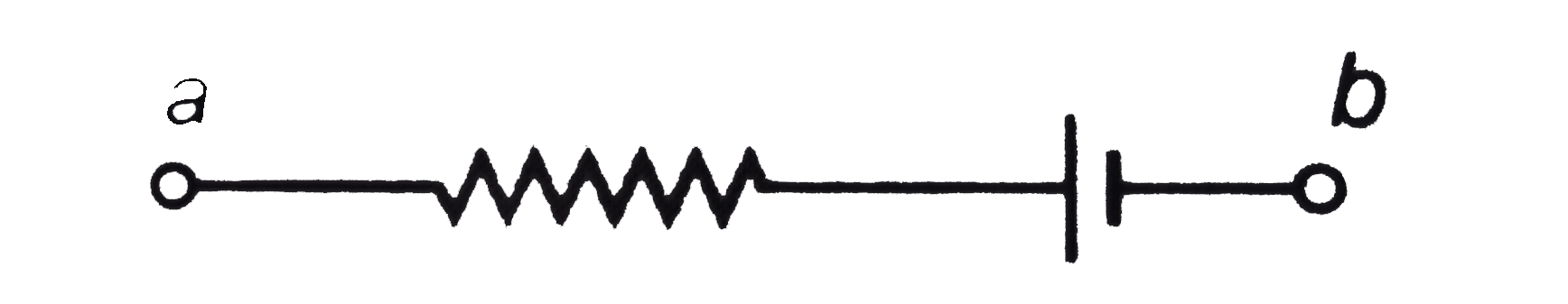
Assertion:In the part of a circuit shown in figure, given that Vb>Va. The current should flow from b to a.
Reason: Direction of current inside a battry is always from negative terminal to positive terminal.
View SolutionAssertion: all monosaccharides are reducing sugar
Reason : monosaccharides contain free aldehyde or free ketonic group
(a) If both assertion & Reason are true & the Reason is a correct explanation of the Assertion
(b) If both Assertion & Reason are True but Reason is not a correct explanation of the Assertion
(c) If Assertion is True but the Reason is False
(d) If both Assertion & Reason are false
View SolutionAssertion : All diploic bones of body are haemopoietic.
Reason : Red bone marrow is present in the diploic bone.
(a) If both Assertion & Reason are True & the Reason is a correct explanation of the Assertion
(b) If both Assertion & Reason are true but Reason is not a correct explanation of the Assertion
(c) If Assertion is True but the Reason is false
(d) If both Assertion & Reason are false
View Solution
