The correct Answer is:
[A]=
Now,
Topper's Solved these Questions
- UNITS, DIMENSIONS & ERROR ANALYSIS Book:DC PANDEY ENGLISHChapter:UNITS, DIMENSIONS & ERROR ANALYSIS Exercise:Medical entrances gallery
Explore 32 Videos
- UNITS, DIMENSIONS & ERROR ANALYSIS Book:DC PANDEY ENGLISHChapter:UNITS, DIMENSIONS & ERROR ANALYSIS Exercise:Assertion and reason
Explore 15 Videos
- UNIT AND DIMENSIONSBook:DC PANDEY ENGLISHChapter:UNIT AND DIMENSIONSExercise:Assertion And Reason
Explore 2 Videos
- VECTORSBook:DC PANDEY ENGLISHChapter:VECTORSExercise:Medical enrances gallery
Explore 9 Videos
Similar Questions
Knowledge Check
Column A1<p<3ColumnBp22p
AIf column A is largerBIf column B is largerCIf the columns are equalDIf there is not enough information to decide
Match the following columns.
Column IColumn IIA.Teslap[ML2A−2T−2]B.Weberq[MLA−2T−1]C.Weber m−2r.[MA−1T−2]D.Henrys.None
View SolutionMatch the following two columns.
Column IColumn IIA.Electrical resistance1.[ML3T−3A−2]B.Electrical potential2.[ML2T−3A−2]C.Specific resistance3.[ML2T−3A−1]D.Specific conductance4.None
View SolutionColumn IColumn II(A)Moment of inertia(p)newton/metre2(B)Surface tension(q)kg/(metre-sec)(C)Angular acceleration(r)kg-metre2(D)Coefficient of viscosity(s)newton/metre(E)Modulus of elasticity(t)radian/sec2
View SolutionMatch the following
Column-IColumn-II(A)Even number(p)227(B)Rational number(q)π(C)Irrational number(r)0(D)Real number(s)√2(t)1.¯¯¯¯¯¯234
View SolutionMatch column I with column II and select the correct option from the given codes
Column IColumn IIA.Autopolyploidy(i)2n+1B.Trisomy(ii)AAAAC.Allopolyploidy(iii)AABBD.Nullisomy(iv)2n-2
View SolutionFour rods of equal length l and mass m each forms a square as shown in figure
Moment of inertia about three axes 1,2 and 3 are say I1,I2andI3. Then, match the following columns
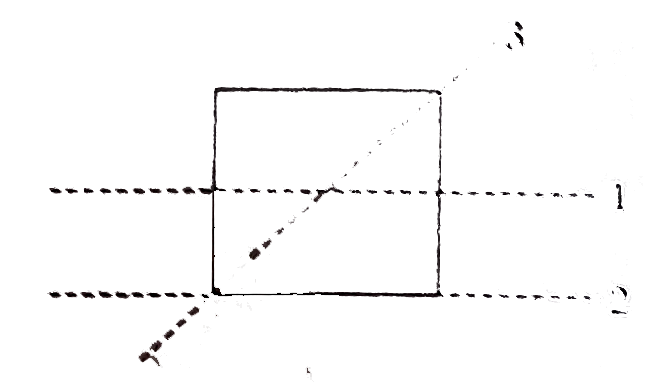
Column-IColumn-II(A)I1(p)43ml2(B)I2(q)23ml2(C)I3(r)12ml2(s)None
View SolutionIn the s-t equation (s=10+20t−5t2) match the following columns.
∣∣ ∣ ∣ ∣ ∣ ∣∣Column IColumn II(A)Distancec travelled in 3s(p)−20 units(B)Displacement 1 s(q)15 units(C)Initial acceleration(r)25 units(D)Velocity at 4 s(s)−10 units∣∣ ∣ ∣ ∣ ∣ ∣∣
View Solution