Two satellites P and Q ratio of masses 3:1 are in circular orbits of radii rand8r. Then ratio of total mechanical energy of A to B is
1:3
3:1
3:4
24:1
The correct Answer is:D
To solve the problem, we need to find the ratio of the total mechanical energy of two satellites P and Q, given their masses and the radii of their orbits.
1. Identify the given data:
- Mass of satellite P, mP=3m
- Mass of satellite Q, mQ=m
- Radius of orbit of satellite P, rP=r
- Radius of orbit of satellite Q, rQ=8r
2. Recall the formula for total mechanical energy (E) of a satellite in orbit:
The total mechanical energy E of a satellite in a circular orbit is given by:
E=−GMm2r
where G is the gravitational constant, M is the mass of the central body (e.g., Earth), m is the mass of the satellite, and r is the radius of the orbit.
3. Calculate the total mechanical energy of satellite P:
Using the formula:
EP=−GMmP2rP=−GM(3m)2r=−3GMm2r
4. Calculate the total mechanical energy of satellite Q:
Using the formula:
EQ=−GMmQ2rQ=−GMm2(8r)=−GMm16r
5. Find the ratio of total mechanical energies EPEQ:
EPEQ=−3GMm2r−GMm16r=3GMm2r×16rGMm
Simplifying this gives:
EPEQ=3×162=482=24
6. Conclusion:
The ratio of the total mechanical energy of satellite P to satellite Q is:
EPEQ=24:1
Final Answer:
The ratio of total mechanical energy of satellite P to satellite Q is 24:1.
- Mass of satellite P, mP=3m
- Mass of satellite Q, mQ=m
- Radius of orbit of satellite P, rP=r
- Radius of orbit of satellite Q, rQ=8r
The total mechanical energy E of a satellite in a circular orbit is given by:
E=−GMm2r
where G is the gravitational constant, M is the mass of the central body (e.g., Earth), m is the mass of the satellite, and r is the radius of the orbit.
Using the formula:
EP=−GMmP2rP=−GM(3m)2r=−3GMm2r
Using the formula:
EQ=−GMmQ2rQ=−GMm2(8r)=−GMm16r
EPEQ=−3GMm2r−GMm16r=3GMm2r×16rGMm
Simplifying this gives:
EPEQ=3×162=482=24
The ratio of the total mechanical energy of satellite P to satellite Q is:
EPEQ=24:1
The ratio of total mechanical energy of satellite P to satellite Q is 24:1.
Topper's Solved these Questions
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (A) Chapter Exercises
68 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (B) Chapter Exercises
31 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - Check Point 10.5
20 Videos
- GENERAL PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE
10 Videos
Similar Questions
Knowledge Check
A satellite is orbiting the earth in a circular orbit of radius r. Its
AKnetic enegy varies as rBangular momentum varies as 1√rClinear momentum varies as 1rDfrequency of revolution varies as 1r3/2For a satellite moving in a circular orbit around the earth, the ratio of its potential energy to kinetic energy is
A1B−1C2D−2
For a satellite moving in a circular orbit around the earth, the ratio of its total mechanical energy to kinetic energy
View SolutionTwo satellites P and Q go round a planet in circular orbits of radii 9R and R respectively. If the speed of the satellite P is 4V, then speed of satellite Q will be
View SolutionTwo satellites A and B go around a planet in circular orbits of radii 4 R and R respectively. If the speed of the satellite A is 3 V, then the speed of the satellite B will be
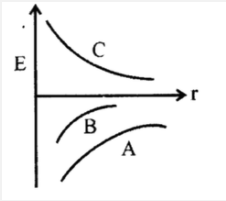
View SolutionAn artificial satellite is made to move in circular orbits of different radii around the earth. The variations of its K.E., P.E. and total energy (E) in different orbits is shown in the figure by different curves. Then for the satellites
View SolutionTwo satellites, A and B, have masses m and 2m respectively. A is in a circular orbit of radius R, and B is in a circular orbit of radius 2R around the earth. The ratio of their kinetic energies, TA/TB, is :
View SolutionTwo satellites , A and B , have masses m and 2m respectively . A is in a circular orbit of radius R , and B is in a circular orbit of radius 2R around the earth . The ratio of their energies , KAKB is :
View Solution
