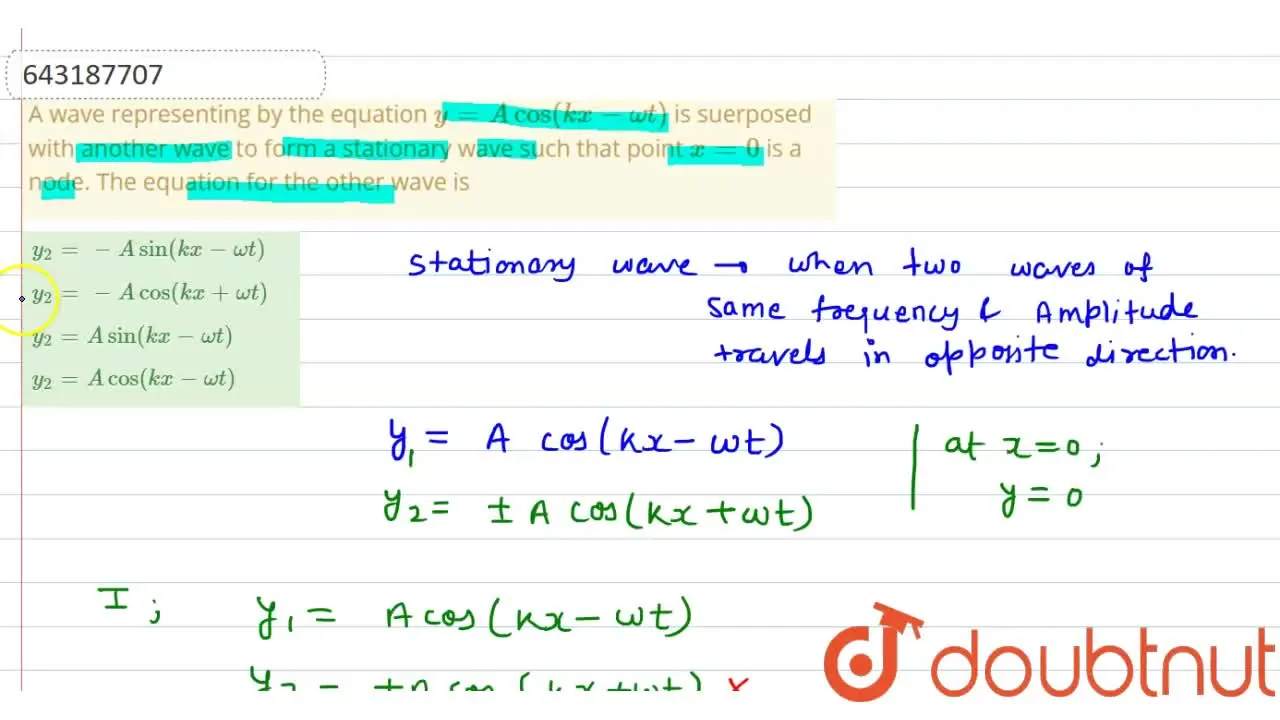
A wave representing by the equation y=Acos(kx−ωt) is suerposed with another wave to form a stationary wave such that point x=0 is a node. The equation for the other wave is
y2=−Asin(kx−ωt)
y2=−Acos(kx+ωt)
y2=Asin(kx−ωt)
y2=Acos(kx−ωt)
The correct Answer is:B
Step by step video & image solution for A wave representing by the equation y = A cos(kx - omegat) is suerposed with another wave to form a stationary wave such that point x = 0 is a node. The equation for the other wave is by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
- BOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - ONLY ONE OPTION IS CORRECT62videos
WAVE MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - More Than One Option is Correct23videos
WAVE MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Subjective Questions2videos
WAVE MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - VECTORSEXERCISE - Medical enrances gallery9videos
VECTORS
- BOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based2videos
WORK, ENERGY & POWER
Similar Questions
A wave represented by the equation y=acos(kx−ωt) is superposed with another wave to form a stationary wave such that the point x=0 is a node . The equation for the other wave is
View SolutionA wave represented by the equation y=acos(kx−ωt) is superposed with another wave to form stationary wave such that the point x=0 is a node. The equation for the other wave is:
View SolutionA wave is represented by the equation y=asin(kx−ωt) is superimposed with another wave to form a stationary wave such that the point x = 0 is a node. Then the equation of other wave is :-
View SolutionA wave represented by a given equation [y(x,t)=asin(ωt−kx)] superimposes on another wave giving a stationary wave having antinode at x = 0 then the equation of the another wave is
View SolutionIn a standing wave, node is a point of
View SolutionThe equation y=Asin2(kx−ωt) represents a wave motion with
View SolutionTwo wave are represented by the equations y1=asinωt ad y2=acosωt the first wave
View SolutionIn a wave motion y=asin(kx−ωt), y can represent
View SolutionA wave of length 2m is superposed on its reflected wave to form a stationary wave. A node is located at x = 3m. The next node will be located at x=
View SolutionIn a wave motion y=asin(kx−ωt),y can represent :-
View Solution