A particle of mass m, kinetic energy K and momentum p collides head on elastically with another particle of mass 2m at rest. After collision, :
Column IColumn II(A)Momentum of first particle(p)3/4p(B)Momentum of second particle(q)−K/9(C)Kinetic energy of first particle(r)−p/3(D)Kinetic energy of second particle(s)8K9(t)None
The correct Answer is:A → r, B →t, D → s
To solve the problem, we will analyze the elastic collision between two particles: one with mass m, kinetic energy K, and momentum p, and another with mass 2m at rest. We will use the principles of conservation of momentum and conservation of kinetic energy.
Step 1: Conservation of Momentum
The initial momentum of the system is given by the momentum of the first particle since the second particle is at rest:
pinitial=mv+0=mv
After the collision, let the velocities of the first and second particles be v1 and v2 respectively. The final momentum is:
pfinal=mv1+2mv2
By conservation of momentum:
mv=mv1+2mv2
Dividing through by m:
v=v1+2v2(1)
Step 2: Conservation of Kinetic Energy
For an elastic collision, the total kinetic energy before and after the collision is conserved. The initial kinetic energy is:
Kinitial=12mv2
The final kinetic energy is:
Kfinal=12mv21+12(2m)v22=12mv21+mv22
By conservation of kinetic energy:
12mv2=12mv21+mv22
Dividing through by m:
12v2=12v21+v22(2)
Step 3: Solve the Equations
From equation (1), we can express v1 in terms of v2:
v1=v−2v2
Substituting this into equation (2):
12v2=12(v−2v2)2+v22
Expanding the square:
12v2=12(v2−4vv2+4v22)+v22
Combining terms:
12v2=12v2−2vv2+2v22
Cancelling 12v2 from both sides:
0=−2vv2+2v22
Factoring out 2v2:
0=2v2(v2−v)
This gives us two solutions:
1. v2=0 (not possible since the second particle is at rest)
2. v2=v
Step 4: Find Velocities
Substituting v2=v back into equation (1):
v=v1+2v⟹v1=v−2v=−v
Step 5: Calculate Momentum and Kinetic Energy
Now we can find the momentum and kinetic energy for both particles after the collision:
- Momentum of first particle:
p1=mv1=m(−v)=−mv=−p
- Momentum of second particle:
p2=2mv2=2m(v)=2mv=2p
- Kinetic energy of first particle:
K1=12m(v21)=12m(v2)=12mv2=K
- Kinetic energy of second particle:
K2=12(2m)(v22)=mv2=2K
Final Results
- Momentum of first particle: −p
- Momentum of second particle: 2p
- Kinetic energy of first particle: K
- Kinetic energy of second particle: 2K
Matching with Options
- (A) Momentum of first particle: −p (not in options)
- (B) Momentum of second particle: 2p (not in options)
- (C) Kinetic energy of first particle: K (not in options)
- (D) Kinetic energy of second particle: 2K (not in options)
The initial momentum of the system is given by the momentum of the first particle since the second particle is at rest:
pinitial=mv+0=mv
After the collision, let the velocities of the first and second particles be v1 and v2 respectively. The final momentum is:
pfinal=mv1+2mv2
By conservation of momentum:
mv=mv1+2mv2
Dividing through by m:
v=v1+2v2(1)
For an elastic collision, the total kinetic energy before and after the collision is conserved. The initial kinetic energy is:
Kinitial=12mv2
The final kinetic energy is:
Kfinal=12mv21+12(2m)v22=12mv21+mv22
By conservation of kinetic energy:
12mv2=12mv21+mv22
Dividing through by m:
12v2=12v21+v22(2)
From equation (1), we can express v1 in terms of v2:
v1=v−2v2
Substituting this into equation (2):
12v2=12(v−2v2)2+v22
Expanding the square:
12v2=12(v2−4vv2+4v22)+v22
Combining terms:
12v2=12v2−2vv2+2v22
Cancelling 12v2 from both sides:
0=−2vv2+2v22
Factoring out 2v2:
0=2v2(v2−v)
This gives us two solutions:
1. v2=0 (not possible since the second particle is at rest)
2. v2=v
Substituting v2=v back into equation (1):
v=v1+2v⟹v1=v−2v=−v
Now we can find the momentum and kinetic energy for both particles after the collision:
- Momentum of first particle:
p1=mv1=m(−v)=−mv=−p
- Momentum of second particle:
p2=2mv2=2m(v)=2mv=2p
K1=12m(v21)=12m(v2)=12mv2=K
- Kinetic energy of second particle:
K2=12(2m)(v22)=mv2=2K
- Momentum of first particle: −p
- Momentum of second particle: 2p
- Kinetic energy of first particle: K
- Kinetic energy of second particle: 2K
- (A) Momentum of first particle: −p (not in options)
- (B) Momentum of second particle: 2p (not in options)
- (C) Kinetic energy of first particle: K (not in options)
- (D) Kinetic energy of second particle: 2K (not in options)
Topper's Solved these Questions
- CENTRE OF MASSBook:DC PANDEY ENGLISHChapter:CENTRE OF MASSExercise:Medical entrances gallery
Explore 27 Videos
- CENTRE OF MASSBook:DC PANDEY ENGLISHChapter:CENTRE OF MASSExercise:Assertion and reason
Explore 21 Videos
- CALORIMETRY AND HEAT TRANSFERBook:DC PANDEY ENGLISHChapter:CALORIMETRY AND HEAT TRANSFERExercise:Medical entrance s gallery
Explore 38 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBook:DC PANDEY ENGLISHChapter:CENTRE OF MASS, IMPULSE AND MOMENTUMExercise:Comprehension type questions
Explore 15 Videos
Similar Questions
A particle of mass m moving with speed u collides perfectly inelastically with another particle of mass 3 m at rest. Loss of KE of system in the collision is
View SolutionA particle of mass m moving with speed V collides elastically with another particle of mass 2m. Find speed of smaller mass after head on collision
View SolutionA particle of mass m moving with speed u collides perfectly inelastically with another particle of mass 2m at rest. Find loss of kinetic energy of system in the collision.
View SolutionA particle of mass m moving with speed u collides perfectly inelastically with another particle of mass 2m at rest. Find loss of kinetic energy of system in the collision.
View SolutionA particle of mass m has momentum p. Its kinetic energy will be
View SolutionA particle moving with kinetic energy E makes a head on elastic collision with an identical particle at rest. During the collision
View SolutionMatch the following: (P = momentum of particle, K = kinetic energy of particle)
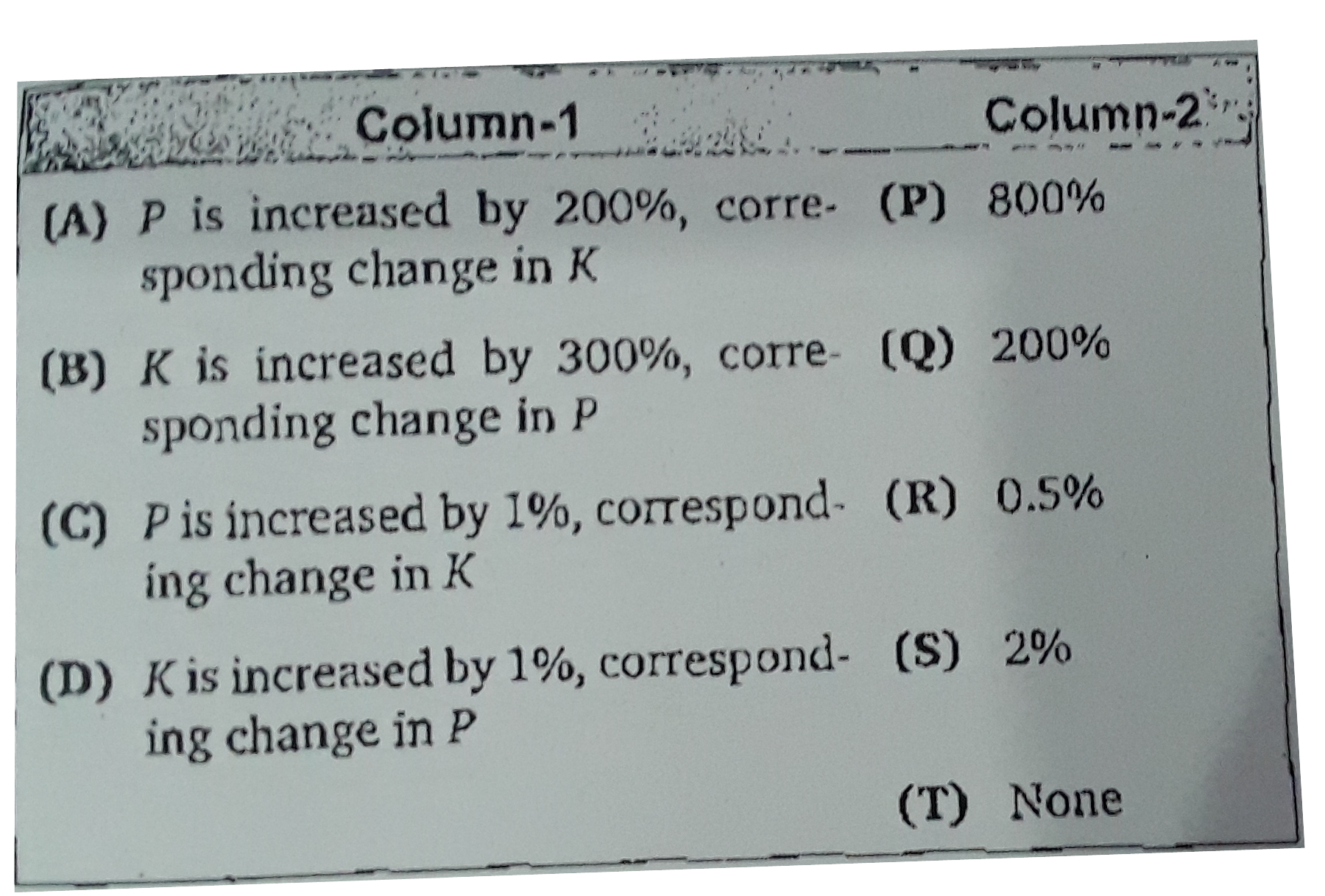
View SolutionMatch the following: (P = momentum of particle, K = kinetic energy of particle)

View SolutionA particle of mass m travelling with velocity v and kinetic energy E collides elastically to another particle of mass nm, at rest. What is the fraction of total energy retained by the particle of mass m?
View SolutionA particle of mass m1 experiences a perfectlly elastic collision with a stationary particle of mass m2. Match the entries in column I to all the entries in column II.
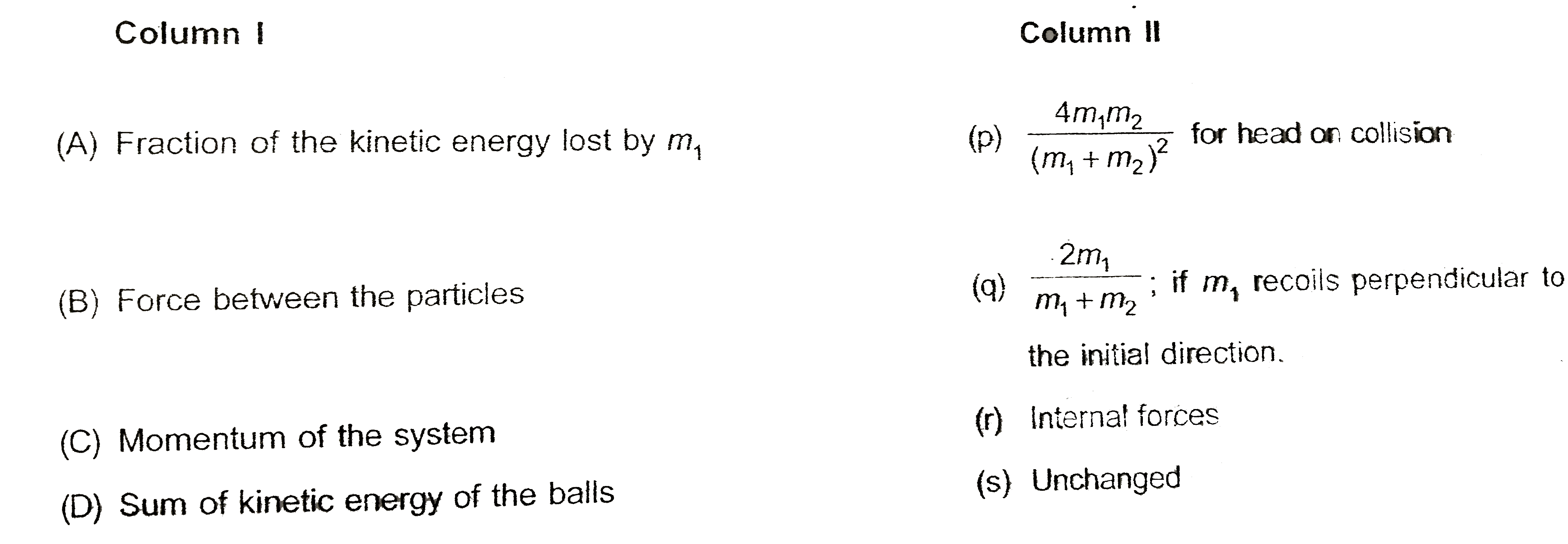
View Solution