Conservation of momentum in a collision beween particles can be understood form
Conservation of energy
Newton's first law
Newton's second law
Both Newton's second and third law
The correct Answer is:D
To solve the question regarding the conservation of momentum in a collision between particles, we can follow these steps:
1. Understanding the Concept of Momentum:
Momentum (p) is defined as the product of mass (m) and velocity (v) of an object, given by the equation:
p=mv
2. Newton's Second Law:
According to Newton's second law, the net external force (F) acting on a system is equal to the rate of change of momentum:
Fexternal=dpdt
If there are no external forces acting on the system (i.e., Fexternal=0), then:
dpdt=0
This implies that the momentum of the system is constant, meaning momentum is conserved.
3. Analyzing a Collision:
In a collision between two particles (e.g., two balls), they exert equal and opposite forces on each other due to Newton's third law. However, if we consider the two balls as a single system, the internal forces they exert on each other do not affect the total momentum of the system.
4. Applying Newton's Third Law:
Newton's third law states that for every action, there is an equal and opposite reaction. During the collision, the forces that the two particles exert on each other are equal in magnitude and opposite in direction. This means that while they may change their individual momenta, the total momentum of the system remains unchanged.
5. Conclusion:
Thus, we conclude that the conservation of momentum in a collision can be understood from both Newton's second law (which indicates that momentum is conserved when no external forces act on the system) and Newton's third law (which describes the internal forces during the collision). Therefore, the correct answer is:
Option D: Both Newton's second and third law
Momentum (p) is defined as the product of mass (m) and velocity (v) of an object, given by the equation:
p=mv
According to Newton's second law, the net external force (F) acting on a system is equal to the rate of change of momentum:
Fexternal=dpdt
If there are no external forces acting on the system (i.e., Fexternal=0), then:
dpdt=0
This implies that the momentum of the system is constant, meaning momentum is conserved.
In a collision between two particles (e.g., two balls), they exert equal and opposite forces on each other due to Newton's third law. However, if we consider the two balls as a single system, the internal forces they exert on each other do not affect the total momentum of the system.
Newton's third law states that for every action, there is an equal and opposite reaction. During the collision, the forces that the two particles exert on each other are equal in magnitude and opposite in direction. This means that while they may change their individual momenta, the total momentum of the system remains unchanged.
Thus, we conclude that the conservation of momentum in a collision can be understood from both Newton's second law (which indicates that momentum is conserved when no external forces act on the system) and Newton's third law (which describes the internal forces during the collision). Therefore, the correct answer is:
Option D: Both Newton's second and third law
Topper's Solved these Questions
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Assertion and reason
21 Videos
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Match the coloumns
9 Videos
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Check points
50 Videos
- CALORIMETRY AND HEAT TRANSFERBOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY AND HEAT TRANSFEREXERCISE - Medical entrance s gallery
38 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
Similar Questions
Knowledge Check
Conservation of momentum in a collision between particles can be understood from
Aconservation of energy.BNewton's first law onlCNewton's second law only.Dboth Newton's second and third law.An elastic collision conserves
Akinetic energy but not momentumBmomentum but not kinetic energyCneither momentum nor kinetic energyDboth kinetic energy and momentum
Water can be conserved by
View SolutionConsider a collision between two particles one of which is at rest and the other strikes it head on with momentum P1. Calculate the energy of reaction Q in terms of the kinetic energy of the particles before and they collide.
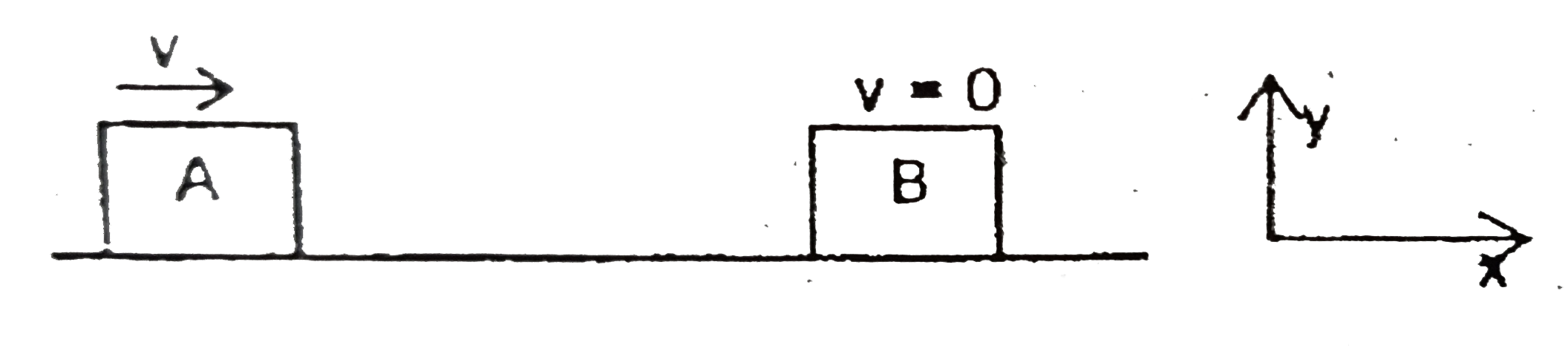
View SolutionA block B is at rest on the rough horizontal surface as shown. Another block A collides with B. Then, which of the following statement is correct?
(i) Impulsive friction acts on block B.
(ii) Conservation of momentum can not be applied on a system (A+B) along x−axis for the situation just before and just after the collision.
(iii) Conservation of momentum can be applied on system (A+B) along x−axis for the situation just before and just after the collision.
(iv) Block A always moves along positive x−axis after collision.
View SolutionThe conservation of angular momentum demands that
View SolutionThe linear momentum is conserved in
View SolutionAssertion : Even though net external force on a body is zero, momentum need not be conserved.
Reason : The internal interaction between particles of a body cancels out momentum of each other
View Solution
