Two perfectly elastic particles A and B of equal masses travelling along a line joining them with velocities 15m/s and 10m/s respectively collide. Their velocities after the elastic collision will be (in m/s) respectively
10ms−1,10ms−1
15ms−1,15ms−1
10ms−1,15ms−1
15ms−1,10ms−1
The correct Answer is:C
To solve the problem of two perfectly elastic particles A and B of equal masses colliding, we can follow these steps:
Step 1: Define the Variables
Let the mass of both particles be m.
- The initial velocity of particle A, uA=15m/s (moving in the positive direction).
- The initial velocity of particle B, uB=−10m/s (moving in the negative direction).
Step 2: Apply Conservation of Momentum
In an elastic collision, momentum is conserved. The equation for conservation of momentum can be written as:
muA+muB=mvA+mvB
Where vA and vB are the final velocities of particles A and B respectively. Since the masses are equal, we can simplify the equation:
uA+uB=vA+vB
Substituting the values:
15+(−10)=vA+vB
5=vA+vB(Equation 1)
Step 3: Apply Conservation of Kinetic Energy
In an elastic collision, kinetic energy is also conserved. The equation for conservation of kinetic energy can be written as:
12mu2A+12mu2B=12mv2A+12mv2B
Again, since the masses are equal, we can simplify:
u2A+u2B=v2A+v2B
Substituting the values:
152+(−10)2=v2A+v2B
225+100=v2A+v2B
325=v2A+v2B(Equation 2)
Step 4: Solve the Equations
Now we have two equations:
1. vA+vB=5
2. v2A+v2B=325
From Equation 1, we can express vB in terms of vA:
vB=5−vA
Substituting this into Equation 2:
v2A+(5−vA)2=325
Expanding the equation:
v2A+(25−10vA+v2A)=325
2v2A−10vA+25=325
2v2A−10vA−300=0
Dividing the entire equation by 2:
v2A−5vA−150=0
Step 5: Use the Quadratic Formula
Using the quadratic formula v=−b±√b2−4ac2a:
Here, a=1,b=−5,c=−150:
vA=−(−5)±√(−5)2−4⋅1⋅(−150)2⋅1
vA=5±√25+6002
vA=5±√6252
vA=5±252
Calculating the two possible values:
1. vA=302=15m/s
2. vA=−202=−10m/s
Step 6: Find vB
Using vB=5−vA:
1. If vA=15:
vB=5−15=−10m/s
2. If vA=−10:
vB=5−(−10)=15m/s
Conclusion
The final velocities after the collision are:
- vA=−10m/s (particle A moves in the opposite direction)
- vB=15m/s (particle B moves in the original direction of A)
Thus, the final velocities of particles A and B after the collision are −10m/s and 15m/s respectively.
Let the mass of both particles be m.
- The initial velocity of particle A, uA=15m/s (moving in the positive direction).
- The initial velocity of particle B, uB=−10m/s (moving in the negative direction).
In an elastic collision, momentum is conserved. The equation for conservation of momentum can be written as:
muA+muB=mvA+mvB
Where vA and vB are the final velocities of particles A and B respectively. Since the masses are equal, we can simplify the equation:
uA+uB=vA+vB
Substituting the values:
15+(−10)=vA+vB
5=vA+vB(Equation 1)
In an elastic collision, kinetic energy is also conserved. The equation for conservation of kinetic energy can be written as:
12mu2A+12mu2B=12mv2A+12mv2B
Again, since the masses are equal, we can simplify:
u2A+u2B=v2A+v2B
Substituting the values:
152+(−10)2=v2A+v2B
225+100=v2A+v2B
325=v2A+v2B(Equation 2)
Now we have two equations:
1. vA+vB=5
2. v2A+v2B=325
vB=5−vA
v2A+(5−vA)2=325
Expanding the equation:
v2A+(25−10vA+v2A)=325
2v2A−10vA+25=325
2v2A−10vA−300=0
Dividing the entire equation by 2:
v2A−5vA−150=0
Using the quadratic formula v=−b±√b2−4ac2a:
Here, a=1,b=−5,c=−150:
vA=−(−5)±√(−5)2−4⋅1⋅(−150)2⋅1
vA=5±√25+6002
vA=5±√6252
vA=5±252
Calculating the two possible values:
1. vA=302=15m/s
2. vA=−202=−10m/s
Using vB=5−vA:
1. If vA=15:
vB=5−15=−10m/s
2. If vA=−10:
vB=5−(−10)=15m/s
The final velocities after the collision are:
- vA=−10m/s (particle A moves in the opposite direction)
- vB=15m/s (particle B moves in the original direction of A)
Topper's Solved these Questions
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Taking it together
73 Videos
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Assertion and reason
21 Videos
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Medical entrances gallery
27 Videos
- CALORIMETRY AND HEAT TRANSFERBOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY AND HEAT TRANSFEREXERCISE - Medical entrance s gallery
38 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
Similar Questions
Two elastic bodies P and Q having equal mases are moving along the same line with velocities of 16 m/s and 10 m/s respectively. Their velocities after the elastic collision will be in m/s :
View SolutionTwo equal masses m1 and m2 moving along the same straight line with velocites +3m/s and −5m/s respectively collide elastically. Their velocities after the collision will be respectively.
View Solution
Knowledge Check
Two identical balls A and B moving with velocities + 0.5 m/s and −0.3.m/s respectively collide head-on elastically. The velocities of the ball A and B after collision will be respectively
A+0.5m/sand+0.3m/sB−0.3m/sand+0.5m/sC + 0.3 m//s and + 0.5 m//sD−0.5m/sand+0.3m/s
Two equal masses m1 and m2 moving along the same straight line with velocites +3m/s and −5m/s respectively collide elastically. Their velocities after the collision will be respectively.
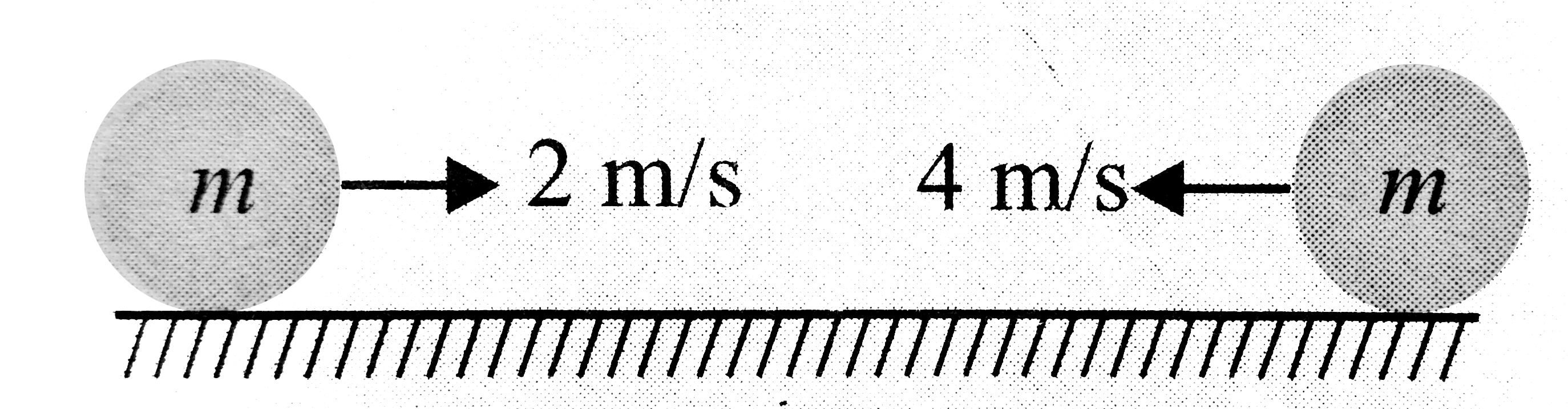
View SolutionTwo identical balls are approaching towards each other on a straight line with velocity 2m/s and 4m/s, respectiely. Find the final velocities after elastic collision between them.
View SolutionTwo identical balls AandB having velocity of 0.5m/sand−0.3m/s respectively collide elastically in one dimension. The velocities of BandA after the collision respectively will be
View SolutionTwo identiacal balls A and B having velocities of 0.5 m/s and 0.3 m/s respectively collide elastically in one dimension. The velocities of B and A after the collision respectively will be
View SolutionTwo particles of masses m and 2m moving in opposite directions collide elastically with velocity 2v and v, respectiely. Find their velocities after collision.

View SolutionTwo objects of masses 100g and 200g are moving along the same line in the same direction with velocities of 2m/s and 1m/s, respectively. They collide and after the collison, the first object moves at a velocity of 1.67m/s in the same direction. Determine the velocity of the second object.
View SolutionTwo balls of masses m each are moving at right angle to each other with velocities 6 m/s and 8 m/s respectively. If collision between them is perfectly inelastic, the velocity of combined mass is
View Solution

