Two blocks of masses 5kgand2kg are placed on a frictionless surface and connected by a spring. An external kick gives a velocity of 14m/s to the heavier block in the direction of lighter one. The magnitudes of velocities of two blocks in the centre of mass frame after the kick are respectively :
To solve the problem step by step, we will follow these steps:
Step 1: Identify the masses and initial conditions
- Let the mass of the first block (m1) be 5 kg and the mass of the second block (m2) be 2 kg.
- Initially, both blocks are at rest, so their initial velocities (u1 and u2) are both 0 m/s.
Step 2: Determine the final velocity of the first block after the kick
- The first block (5 kg) receives an external kick, giving it a velocity (v1) of 14 m/s towards the second block.
- The second block (2 kg) remains at rest immediately after the kick, so its velocity (v2) is 0 m/s.
Step 3: Calculate the velocity of the center of mass (Vcm)
- The velocity of the center of mass (Vcm) can be calculated using the formula:
Vcm=m1v1+m2v2m1+m2
- Substituting the known values:
Vcm=(5kg×14m/s)+(2kg×0m/s)5kg+2kg=70kg m/s7kg=10m/s
Step 4: Calculate the velocities of the blocks in the center of mass frame
- The velocity of the first block (m1) in the center of mass frame is:
v1,cm=v1−Vcm=14m/s−10m/s=4m/s
- The velocity of the second block (m2) in the center of mass frame is:
v2,cm=v2−Vcm=0m/s−10m/s=−10m/s
(The negative sign indicates that the second block is moving in the opposite direction relative to the center of mass.)
Step 5: Present the magnitudes of the velocities
- The magnitudes of the velocities of the two blocks in the center of mass frame are:
- For block 1 (5 kg): |v1,cm|=4m/s
- For block 2 (2 kg): |v2,cm|=10m/s
Final Answer
The magnitudes of the velocities of the two blocks in the center of mass frame after the kick are:
- Block 1 (5 kg): 4 m/s
- Block 2 (2 kg): 10 m/s
---
- Let the mass of the first block (m1) be 5 kg and the mass of the second block (m2) be 2 kg.
- Initially, both blocks are at rest, so their initial velocities (u1 and u2) are both 0 m/s.
- The first block (5 kg) receives an external kick, giving it a velocity (v1) of 14 m/s towards the second block.
- The second block (2 kg) remains at rest immediately after the kick, so its velocity (v2) is 0 m/s.
- The velocity of the center of mass (Vcm) can be calculated using the formula:
Vcm=m1v1+m2v2m1+m2
- Substituting the known values:
Vcm=(5kg×14m/s)+(2kg×0m/s)5kg+2kg=70kg m/s7kg=10m/s
- The velocity of the first block (m1) in the center of mass frame is:
v1,cm=v1−Vcm=14m/s−10m/s=4m/s
- The velocity of the second block (m2) in the center of mass frame is:
v2,cm=v2−Vcm=0m/s−10m/s=−10m/s
(The negative sign indicates that the second block is moving in the opposite direction relative to the center of mass.)
- The magnitudes of the velocities of the two blocks in the center of mass frame are:
- For block 1 (5 kg): |v1,cm|=4m/s
- For block 2 (2 kg): |v2,cm|=10m/s
The magnitudes of the velocities of the two blocks in the center of mass frame after the kick are:
- Block 1 (5 kg): 4 m/s
- Block 2 (2 kg): 10 m/s
Topper's Solved these Questions
- CENTRE OF MASSBook:DC PANDEY ENGLISHChapter:CENTRE OF MASSExercise:Check points
Explore 50 Videos
- CENTRE OF MASSBook:DC PANDEY ENGLISHChapter:CENTRE OF MASSExercise:Taking it together
Explore 73 Videos
- CALORIMETRY AND HEAT TRANSFERBook:DC PANDEY ENGLISHChapter:CALORIMETRY AND HEAT TRANSFERExercise:Medical entrance s gallery
Explore 38 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBook:DC PANDEY ENGLISHChapter:CENTRE OF MASS, IMPULSE AND MOMENTUMExercise:Comprehension type questions
Explore 15 Videos
Similar Questions
Figure shows two blocks of masses 5kg and 2kg placed on a frictionless surface and connected with a spring. An external kick gives a velocity of 14m/s to the heavier block in the direction of lighter one. Deduce (a) velocity gained by the centre of mass and (b) the separate velocities of the two blocks in the centre of mass coordinates just after the kick.
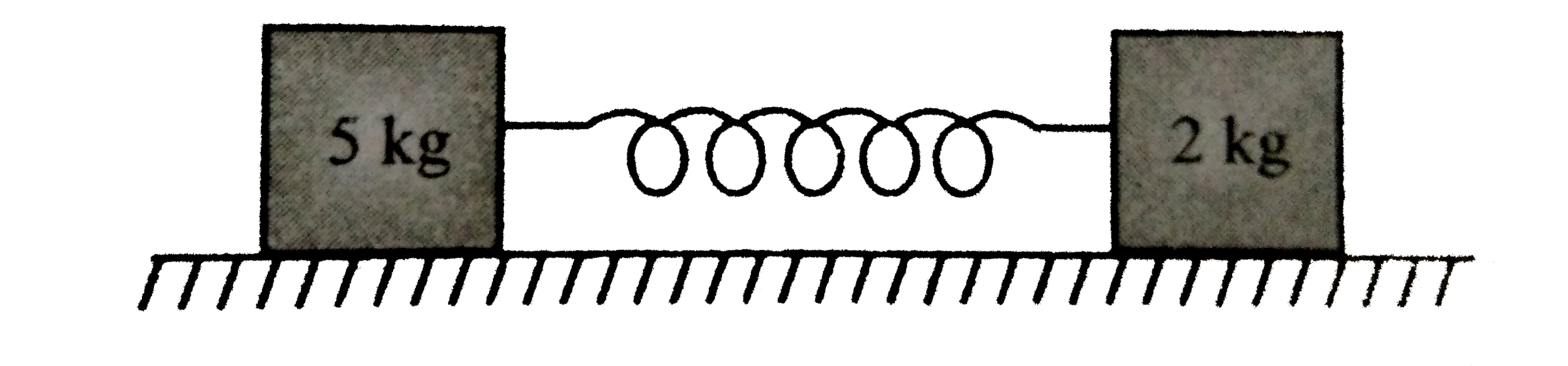
View SolutionTwo blocks of masses 10 kg and 4 kg are connected by a spring of negligible mass and placed on a frictionless horizontal surface. An impulse gives a velocity of 14m/s to the heavier block in the direction of the lighter block. The velocity of the centre of mass is
View Solution
Knowledge Check
Two blocks of masses of 40 kg and 30 kg are connected by a weightless string passing over a frictionless pulley as shown in the figure.
 A0.7ms−2B0.8ms−2C0.6ms−2D0.5ms−2
A0.7ms−2B0.8ms−2C0.6ms−2D0.5ms−2
Two blocks of masses 5kg and 2kg are connected by a spring of negligible mass and placed on a frictionless horizontal surface. An impulse provides a velocity of 7m/s to the heavier block in the direction of the lighter block. The velocity of the centre of mass is :-
View SolutionTwo blocks of masses 5kg and 2kg are connected by a spring of negligible mass and placed on a frictionless horizontal surface. An impulse provides a velocity of 7m/s to the heavier block in the direction of the lighter block. The velocity of the centre of mass is :-
View SolutionTwo blocks of masses 5kg and 2kg are connected by a spring of negligible mass and placed on a frictionless horizontal surface. An impulse provides a velocity of 7m/s to the heavier block in the direction of the lighter block. The velocity of the centre of mass is :-
View SolutionTwo blocks of masses 4kg and 6kg are kept on a rough horizontal surface as shown. If the acceleration of 6kg block is 5n, then n is :

View SolutionTwo blocks of masses 1 kg and 2 kg are placed in contact on a smooth horizontal surface as shown in fig. A horizontal force of 6N is applied d(a) on a 1-kg block. Find the force of interaction of the blocks.

 .
.
View SolutionTwo blocks of masses 5 kg and 2 kg are kept in contact with each other on a frictionless horizontal surface. If a force of 14 N is applied on the larger block what is the acceleration of the system ? What is the contact force between the two blocks ?
View SolutionTwo blocks A and B of mass 16kg and 64kg are placed on a smooth horizontal surface. Blocks are connected by a spring of spring constant 100N/m. Find the ratio of maximum speed of block A to maximum speed of block B.
View Solution
