Nuclei of a radioactive element A are being produced at a constant rate α. The element has a decay constant λ. At time t=0, there are N0 nuclei of the element.
(a) Calculate the number N of nuclei of A at time t.
(b) If α=2N0λ, calculate the number of nuclei of A after one half-life of A, and also the limiting value of N as t→∞.
The correct Answer is:A, B, C, D
To solve the problem step by step, we will break it down into parts (a) and (b) as specified in the question.
Part (a): Calculate the number N of nuclei of A at time t.
1. Understanding the Problem:
We have a radioactive element A that is being produced at a constant rate α and decaying at a rate characterized by the decay constant λ. At time t=0, the number of nuclei is N0.
2. Setting Up the Differential Equation:
The rate of change of the number of nuclei N can be expressed as:
dNdt=α−λN
Here, α is the production rate and λN is the decay rate.
3. Rearranging the Equation:
Rearranging gives:
dNα−λN=dt
4. Integrating Both Sides:
We will integrate both sides. The left side will be integrated from N0 to N and the right side from 0 to t:
∫NN0dNα−λN=∫t0dt
5. Calculating the Left Integral:
The integral on the left can be solved using the natural logarithm:
−1λln|α−λN|∣∣∣NN0=t
This leads to:
−1λ(ln|α−λN|−ln|α−λN0|)=t
6. Simplifying the Equation:
This simplifies to:
ln(α−λN0α−λN)=−λt
7. Exponentiating Both Sides:
Exponentiating gives:
α−λNα−λN0=e−λt
8. Solving for N:
Rearranging this equation leads to:
α−λN=(α−λN0)e−λt
Thus,
λN=α−(α−λN0)e−λt
Finally, we have:
N=αλ−α−λN0λe−λt
Part (b): If α=2N0λ, calculate the number of nuclei of A after one half-life of A, and also the limiting value of N as t→∞.
1. Substituting α:
Given α=2N0λ, we substitute this into the equation derived in part (a):
N=2N0λλ−2N0λ−λN0λe−λt
Simplifying gives:
N=2N0−(2N0−N0)e−λt=2N0−N0e−λt
2. Calculating After One Half-Life:
The half-life t1/2 is given by:
t1/2=ln2λ
Substituting t=t1/2:
N=2N0−N0e−ln2=2N0−N0⋅12=2N0−N02=4N02−N02=3N02
3. Finding the Limiting Value as t→∞:
As t→∞, e−λt→0:
N=2N0−N0⋅0=2N0
Final Answers:
- (a) The number of nuclei of A at time t is:
N=2N0λ−(2N0−N0)e−λtλ
- (b) After one half-life, N=3N02 and as t→∞, N=2N0.
We have a radioactive element A that is being produced at a constant rate α and decaying at a rate characterized by the decay constant λ. At time t=0, the number of nuclei is N0.
The rate of change of the number of nuclei N can be expressed as:
dNdt=α−λN
Here, α is the production rate and λN is the decay rate.
Rearranging gives:
dNα−λN=dt
We will integrate both sides. The left side will be integrated from N0 to N and the right side from 0 to t:
∫NN0dNα−λN=∫t0dt
The integral on the left can be solved using the natural logarithm:
−1λln|α−λN|∣∣∣NN0=t
This leads to:
−1λ(ln|α−λN|−ln|α−λN0|)=t
This simplifies to:
ln(α−λN0α−λN)=−λt
Exponentiating gives:
α−λNα−λN0=e−λt
Rearranging this equation leads to:
α−λN=(α−λN0)e−λt
Thus,
λN=α−(α−λN0)e−λt
Finally, we have:
N=αλ−α−λN0λe−λt
Given α=2N0λ, we substitute this into the equation derived in part (a):
N=2N0λλ−2N0λ−λN0λe−λt
Simplifying gives:
N=2N0−(2N0−N0)e−λt=2N0−N0e−λt
The half-life t1/2 is given by:
t1/2=ln2λ
Substituting t=t1/2:
N=2N0−N0e−ln2=2N0−N0⋅12=2N0−N02=4N02−N02=3N02
As t→∞, e−λt→0:
N=2N0−N0⋅0=2N0
- (a) The number of nuclei of A at time t is:
N=2N0λ−(2N0−N0)e−λtλ
- (b) After one half-life, N=3N02 and as t→∞, N=2N0.
Topper's Solved these Questions
- MODERN PHYSICS - 2BOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICS - 2EXERCISE - Level 2 Comprehension Based
3 Videos
- MODERN PHYSICS - 1BOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICS - 1EXERCISE - Level 2 Subjective
23 Videos
- NUCLEIBOOK - DC PANDEY ENGLISHCHAPTER - NUCLEIEXERCISE - C MADICAL ENTRANCES GALLERY
46 Videos
Similar Questions
Nuclei of a radioactive element X are being produced at a constant rate K and this element decays to a stable nucleus Y with a decay constant λ and half-life T1/3. At the time t=0, there are N0nuclei of the element X.
The number NY of nuclei of Y at time t is .
View SolutionNuclei of a radioactive element X are being produced at a constant rate K and this element decays to a stable nucleus Y with a decay constant λ and half-life T1/2. At the time t=0, there are N0nuclei of the element X.
The number NX of nuclei of X at time t=T1/2 is .
View Solution
Knowledge Check
Nuclei of a radioactive element A are being produced at a constant rate α. The element has a decay constant λ. At time t=0, there are N0 nuclei of the element.
(a) Calculate the number N of nuclei of A at time t.
(b) If α=2N0λ, calculate the number of nuclei of A after one half-life of A, and also the limiting value of N as t→∞.A1λ[α+(α−N0λ)e−λt]B1λ[α−(α−N0λ)e−λt]Cλ[α−(α−N0λ)e−λt]D[α−(N0λ−α)e−λt]
A rodiactive nucleus is being produced at a constant rate α per second Its decay constant is λ If N0 are the number of nuclie at tieme t=O then, maximum number of nuclei possible are
View SolutionA radioacitve nucleus is being produced at a constant rate α per second. Its decay constant is λ. If N0 are the number of nuclei at time t=0, then maximum number of nuceli possible are .
View SolutionNuclei of radioactive element A are produced at rate t2 (where t is time) at any time t. The element A has decay constant λ. Let N be the number of nuclei of element A at any time t. At time t=t0,dN/dt is minimum. The number of nuclei of element A at time t=t0 is
View SolutionA radioactive substance (A) is produced at a constant rate which decays with a decay constant λ to form stable substance (B).If production of A starts at t=0 . Find (i)The number of nuclei of A and (ii)Number of nuclei of B at any time t.
View SolutionA radioactive sample undergoes decay as per the following gragp. At timet=0, the number of undecayed nuclei is N0. Calculate the number of nuclei left after 1h.
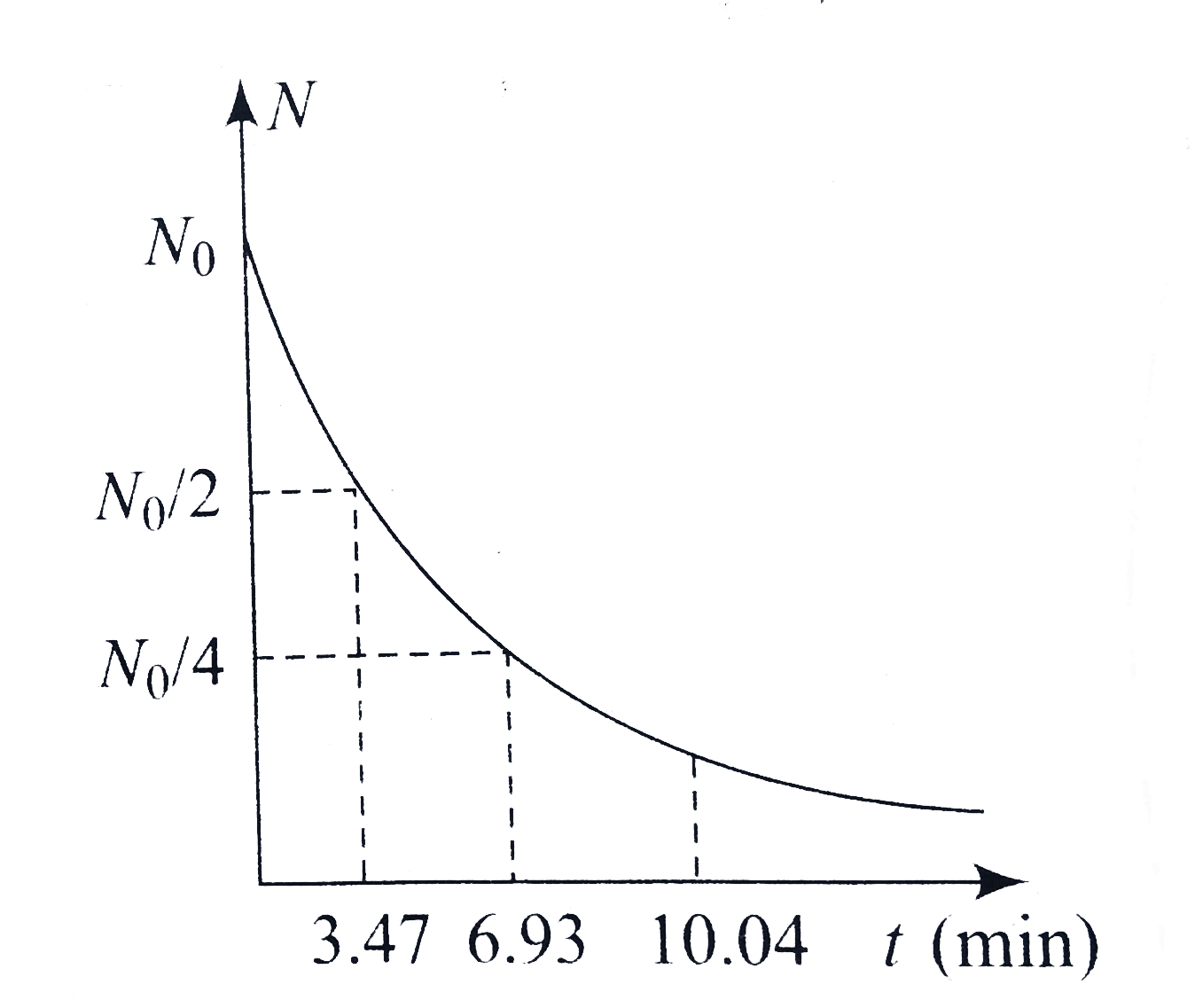 .
.
View SolutionA radioactive sample undergoes decay as per the following gragp. At timet=0, the number of undecayed nuclei is N0. Calculate the number of nuclei left after 1h.
 .
.
View SolutionA radio nuclide X is produced at constant rate α. At time t=0, number of nuclei of X are zero. Find
(a) the maximum number of nuclei of X.
the number of nuclei at time t.
Decay constant of X is λ.
View Solution
