Magnitude of focal length of a spherical mirror is f and magnitude of linear magnification is 12
If image is inverted, it is a concave mirror
If image is erect, it is a convex mirror
Object distance from the mirror may be 3f
Object distance from the mirror may be f.
The correct Answer is:A, B, C, D
To solve the problem, we need to analyze the situation for both types of spherical mirrors: concave and convex. We will use the mirror formula and the magnification formula to derive the object distance and determine the type of mirror based on the given conditions.
1. Understanding the Given Information:
- Magnitude of focal length of a spherical mirror: f
- Magnitude of linear magnification: 12
2. Determine the Type of Mirror:
- If the image is inverted, it indicates a concave mirror.
- If the image is erect, it indicates a convex mirror.
3. Case 1: Convex Mirror
- For a convex mirror, the object distance u is negative, so we can denote it as u=−x.
- The magnification m for a convex mirror is given by:
m=h′h=vu
where v is the image distance. Given m=12, we have:
v−x=12⟹v=−x2
- The focal length f for a convex mirror is positive.
4. Using the Mirror Formula:
- The mirror formula is:
1f=1v+1u
- Substituting the values:
1f=1−x2+1−x
- Simplifying:
1f=−2x−1x=−3x
- Therefore, we get:
f=−x3
- Since f is positive for a convex mirror, this case is valid if x=3f.
5. Case 2: Concave Mirror
- For a concave mirror, the object distance u is again negative, so we denote it as u=−x.
- The image distance v for a concave mirror, given the image is inverted (real), is:
v=−x2
- The focal length f for a concave mirror is negative.
6. Using the Mirror Formula Again:
- Substituting into the mirror formula:
1−f=1−x2+1−x
- Simplifying:
−1f=−2x−1x=−3x
- Therefore:
f=x3
- Since f is negative for a concave mirror, this case is valid if x=3f.
Conclusion:
- If the image is erect, it is a convex mirror with object distance x=f.
- If the image is inverted, it is a concave mirror with object distance x=3f.
Final Answer:
- The statements are correct for both types of mirrors:
- For a convex mirror: Object distance may be f.
- For a concave mirror: Object distance may be 3f.
- Magnitude of focal length of a spherical mirror: f
- Magnitude of linear magnification: 12
- If the image is inverted, it indicates a concave mirror.
- If the image is erect, it indicates a convex mirror.
- For a convex mirror, the object distance u is negative, so we can denote it as u=−x.
- The magnification m for a convex mirror is given by:
m=h′h=vu
where v is the image distance. Given m=12, we have:
v−x=12⟹v=−x2
- The focal length f for a convex mirror is positive.
- The mirror formula is:
1f=1v+1u
- Substituting the values:
1f=1−x2+1−x
- Simplifying:
1f=−2x−1x=−3x
- Therefore, we get:
f=−x3
- Since f is positive for a convex mirror, this case is valid if x=3f.
- For a concave mirror, the object distance u is again negative, so we denote it as u=−x.
- The image distance v for a concave mirror, given the image is inverted (real), is:
v=−x2
- The focal length f for a concave mirror is negative.
- Substituting into the mirror formula:
1−f=1−x2+1−x
- Simplifying:
−1f=−2x−1x=−3x
- Therefore:
f=x3
- Since f is negative for a concave mirror, this case is valid if x=3f.
- If the image is erect, it is a convex mirror with object distance x=f.
- If the image is inverted, it is a concave mirror with object distance x=3f.
- The statements are correct for both types of mirrors:
- For a convex mirror: Object distance may be f.
- For a concave mirror: Object distance may be 3f.
Topper's Solved these Questions
- REFLECTION OF LIGHTBook:DC PANDEY ENGLISHChapter:REFLECTION OF LIGHTExercise:Level 2 Comprehensive Based
Explore 4 Videos
- REFLECTION OF LIGHTBook:DC PANDEY ENGLISHChapter:REFLECTION OF LIGHTExercise:Subjective
Explore 9 Videos
- REFLECTION OF LIGHTBook:DC PANDEY ENGLISHChapter:REFLECTION OF LIGHTExercise:Level 2 Single Correct
Explore 16 Videos
- RAY OPTICSBook:DC PANDEY ENGLISHChapter:RAY OPTICSExercise:Medical entrance gallary
Explore 76 Videos
- REFRACTION OF LIGHTBook:DC PANDEY ENGLISHChapter:REFRACTION OF LIGHTExercise:Level 2 Subjective
Explore 21 Videos
Similar Questions
Focal length of a concave mirror is
View SolutionFocal length of a convex mirror is 10 cm
View SolutionHow is the focal length of a spherical mirror related to its radius of curvature?
View SolutionA small object is placed at distance of 3.6 cm from a magnifier of focal length 4.0 cm. a. find the position of theimage. B. find the linear magnification c. Find the angular magnification.
View SolutionFor a plane mirror what is the focal length? The magnification?
View SolutionThe given ray diagram illustrates the experimental set up for the determination of the focal length of a converging lens using a plane mirror.
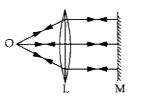
state the magnification of the image formed .
View SolutionCalculate focal length of a spherical mirror from the following observations : object distance, u=(50.1±0.5)cm and image distance , υ=(20.1±0.2)cm.
View SolutionAn object is moving away from concave mirror of focal length f starting from focus. The distance of an object from pole of mirror is x. The correct graph of magnitude of magnification(m) verses distance x is:
View SolutionAn object is 30.0 cm from a spherical mirror along the central axis. The absolute value of lateral magnification is 12. The image produced is inverted. What is the focal length of the mirror?
View SolutionAn object is 30.0 cm form a spherical mirror, along the central axis.
The absolute value of lateral magnification is 12, The image produced is inverted .
What is the focal length of the mirror?
View Solution