A boy of height 1.5m with his eye level at 1.4m stands before a plane mirror of length 0.75 m fixed on the wall. The height of the lower edge of the mirror above the floor is 0.8 m. Then ,
the boy will see his full image
the boy cannot see his hair
the boy cannot see his feet
the boy can see neither his hair nor his feet.
The correct Answer is:C
To solve the problem, we need to determine whether the boy can see his head and feet in the mirror based on the given dimensions.
1. Identify the Given Data:
- Height of the boy (H_boy) = 1.5 m
- Eye level of the boy (H_eye) = 1.4 m
- Length of the mirror (L_mirror) = 0.75 m
- Height of the lower edge of the mirror from the floor (H_lower_mirror) = 0.8 m
2. Determine the Height of the Upper Edge of the Mirror:
- The height of the upper edge of the mirror (H_upper_mirror) can be calculated as:
Hupper_mirror=Hlower_mirror+Lmirror=0.8m+0.75m=1.55m
3. Check if the Boy Can See His Head:
- The boy's head is at a height of 1.5 m.
- The upper edge of the mirror is at 1.55 m, which is higher than the height of the boy's head.
- Since the line of sight from the top of the boy's head to the mirror will reflect back to his eyes, he can see his head.
4. Check if the Boy Can See His Feet:
- The boy's feet are at a height of 0 m (floor level).
- The lower edge of the mirror is at 0.8 m.
- We need to see if the reflected ray from the feet can reach the boy's eyes.
- The incident ray from the feet to the mirror will reflect and must meet the boy's eye level (1.4 m).
5. Using Similar Triangles:
- The height from the floor to the lower edge of the mirror is 0.8 m.
- The distance from the lower edge of the mirror to the boy's eye level is:
Heye−Hlower_mirror=1.4m−0.8m=0.6m
- The distance from the feet to the lower edge of the mirror is 0.8 m.
- Using similar triangles, we can set up the ratio:
HeyeDistancefeet=Hlower_mirrorDistanceeye_level
0.60.8=Hlower_mirrorDistanceeye_level
- Solving this gives us:
Hlower_mirror=0.6×0.80.8=0.6m
- Since the height of the boy's eyes (1.4 m) is lower than the height where the reflected ray would meet, he cannot see his feet.
6. Conclusion:
- The boy can see his head but cannot see his feet in the mirror.
Final Answer:
The boy cannot see his feet in the mirror.
- Height of the boy (H_boy) = 1.5 m
- Eye level of the boy (H_eye) = 1.4 m
- Length of the mirror (L_mirror) = 0.75 m
- Height of the lower edge of the mirror from the floor (H_lower_mirror) = 0.8 m
- The height of the upper edge of the mirror (H_upper_mirror) can be calculated as:
Hupper_mirror=Hlower_mirror+Lmirror=0.8m+0.75m=1.55m
- The boy's head is at a height of 1.5 m.
- The upper edge of the mirror is at 1.55 m, which is higher than the height of the boy's head.
- Since the line of sight from the top of the boy's head to the mirror will reflect back to his eyes, he can see his head.
- The boy's feet are at a height of 0 m (floor level).
- The lower edge of the mirror is at 0.8 m.
- We need to see if the reflected ray from the feet can reach the boy's eyes.
- The incident ray from the feet to the mirror will reflect and must meet the boy's eye level (1.4 m).
- The height from the floor to the lower edge of the mirror is 0.8 m.
- The distance from the lower edge of the mirror to the boy's eye level is:
Heye−Hlower_mirror=1.4m−0.8m=0.6m
- The distance from the feet to the lower edge of the mirror is 0.8 m.
- Using similar triangles, we can set up the ratio:
HeyeDistancefeet=Hlower_mirrorDistanceeye_level
0.60.8=Hlower_mirrorDistanceeye_level
- Solving this gives us:
Hlower_mirror=0.6×0.80.8=0.6m
- Since the height of the boy's eyes (1.4 m) is lower than the height where the reflected ray would meet, he cannot see his feet.
- The boy can see his head but cannot see his feet in the mirror.
The boy cannot see his feet in the mirror.
Topper's Solved these Questions
- REFLECTION OF LIGHTBook:DC PANDEY ENGLISHChapter:REFLECTION OF LIGHTExercise:Exercise Level 1 Subjective
Explore 17 Videos
- REFLECTION OF LIGHTBook:DC PANDEY ENGLISHChapter:REFLECTION OF LIGHTExercise:Level 2 Single Correct
Explore 16 Videos
- REFLECTION OF LIGHTBook:DC PANDEY ENGLISHChapter:REFLECTION OF LIGHTExercise:Exercise Level 1 Assertion And Reason
Explore 10 Videos
- RAY OPTICSBook:DC PANDEY ENGLISHChapter:RAY OPTICSExercise:Medical entrance gallary
Explore 76 Videos
- REFRACTION OF LIGHTBook:DC PANDEY ENGLISHChapter:REFRACTION OF LIGHTExercise:Level 2 Subjective
Explore 21 Videos
Similar Questions
A person wants to see AB part of his image. His eye level is at 1.8m above the ground. If he uses minimum size of mirror required for this, find the height of the lowest point of mirror above the ground.
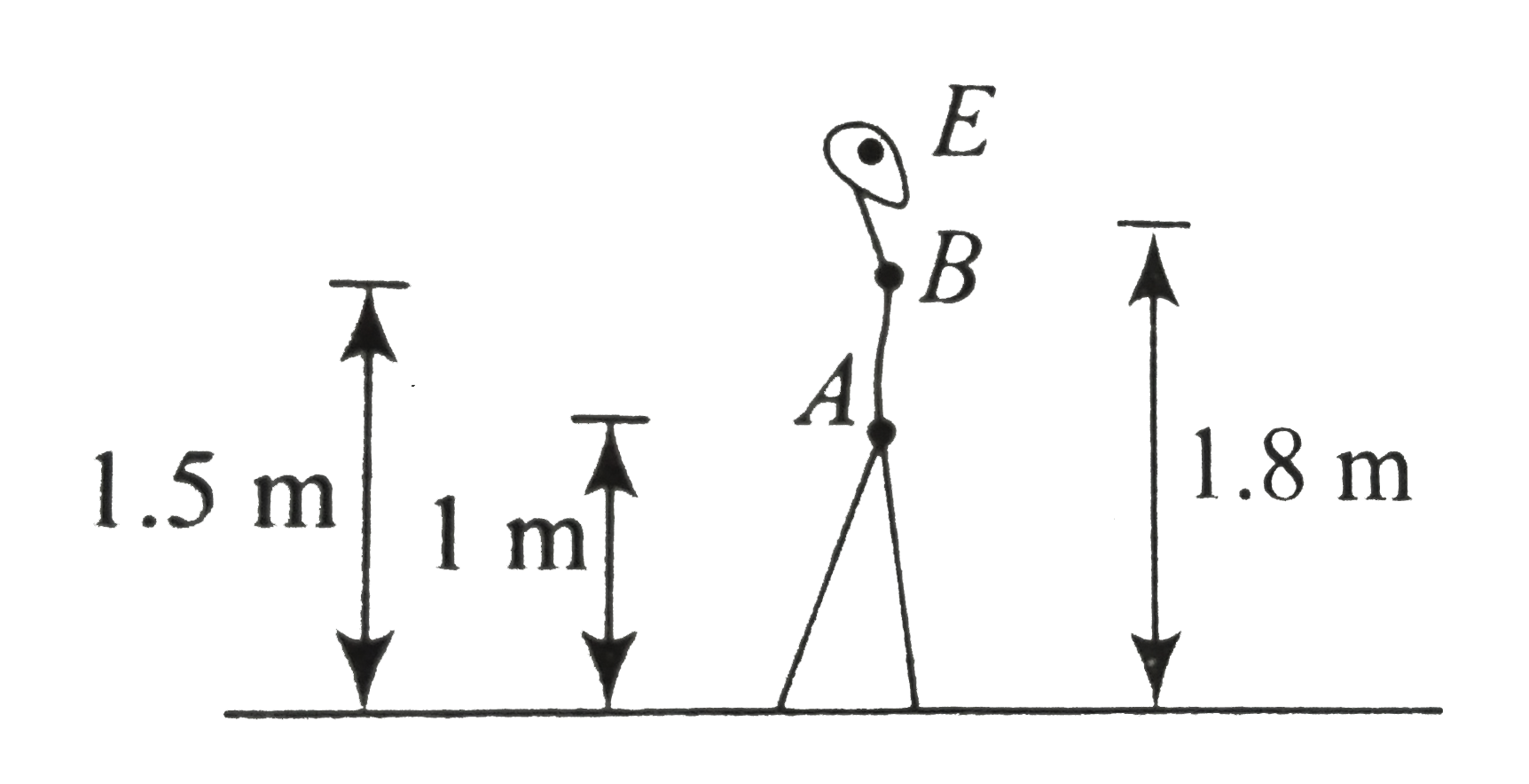
View SolutionA pole of height 4 m is kept in front of a vertical plane mirror
of length 2m. The lower end of the mirror is at a height of 6m form the grown
.the horizontal distance betweem the mirror and the pole is 2m. Upto what
minimum and maximum heights a man can see the image of top of the pole at a
horizontal distance of 4m (from the mirror O standing on the same horizontal
line which is passing through the pole and the horizontal point below the
mirror?

View Solution
Knowledge Check
A boy of height 1m stands in front of a convex mirror. His distance from the mirror is equal to its focal length. The height of his image is
A0.25mB0.33mC0.5mD0.67m
A person's eye is at a height of 1.5 m . He stands infront of a 0.3 m long plane mirror whose lower end is 0.8m above the ground. The length of the image he sees of himself is
View SolutionA tree grows at a rate of 0.5 m/yr . What will be the height of a board fixed at 1.5 m above the base, five years ago
View SolutionA plane mirror 50cm long, is hung on a vetical wall of a room, with its lower edge 50cm above the ground. A man stands of the mirror at a distance 2m away from the mirror. If his eyes are at a height 1.8m above the ground, then the length (distance between the extreme points of the visible region perpendicular to the mirror ) of the floor visible to him due to refliection from the mirror is x26m . Find the value of x
View SolutionAt what distance from a convex mirror of focal length 2.5 m should as boy stand so that his image has a height equal to half the original height?
View SolutionIn a gravity free space, man of mass M standing at a height h above the floor, throws a ball of mass m straight down with a speed u. When the ball reaches the floor, the distance of the man above the floor will be.
View SolutionA man of 50kg mass is standing in a gravity free space at a height of 10m above the floor. He throws a stone of 0.5kg mass downwards with a speed 2m/s. When the stone reaches the floor, the distance of the man above the floor will be
View SolutionA man of 50kg mass is standing in a gravity free space at a height of 10m above the floor. He throws a stone of 0.5kg mass downwards with a speed 2m/s. When the stone reaches the floor, the distance of the man above the floor will be
View Solution