A battery of emf E and of negligible internal resistance is connected in a L−R circuit as shown in figure. The inductor has a piece of soft iron inside it. When steady state is reached in the piece of soft iron is abruptly pulled out suddenly so that the inductance of the inductor decreases to nL with n<1 with battery remaining connected. Calculate.
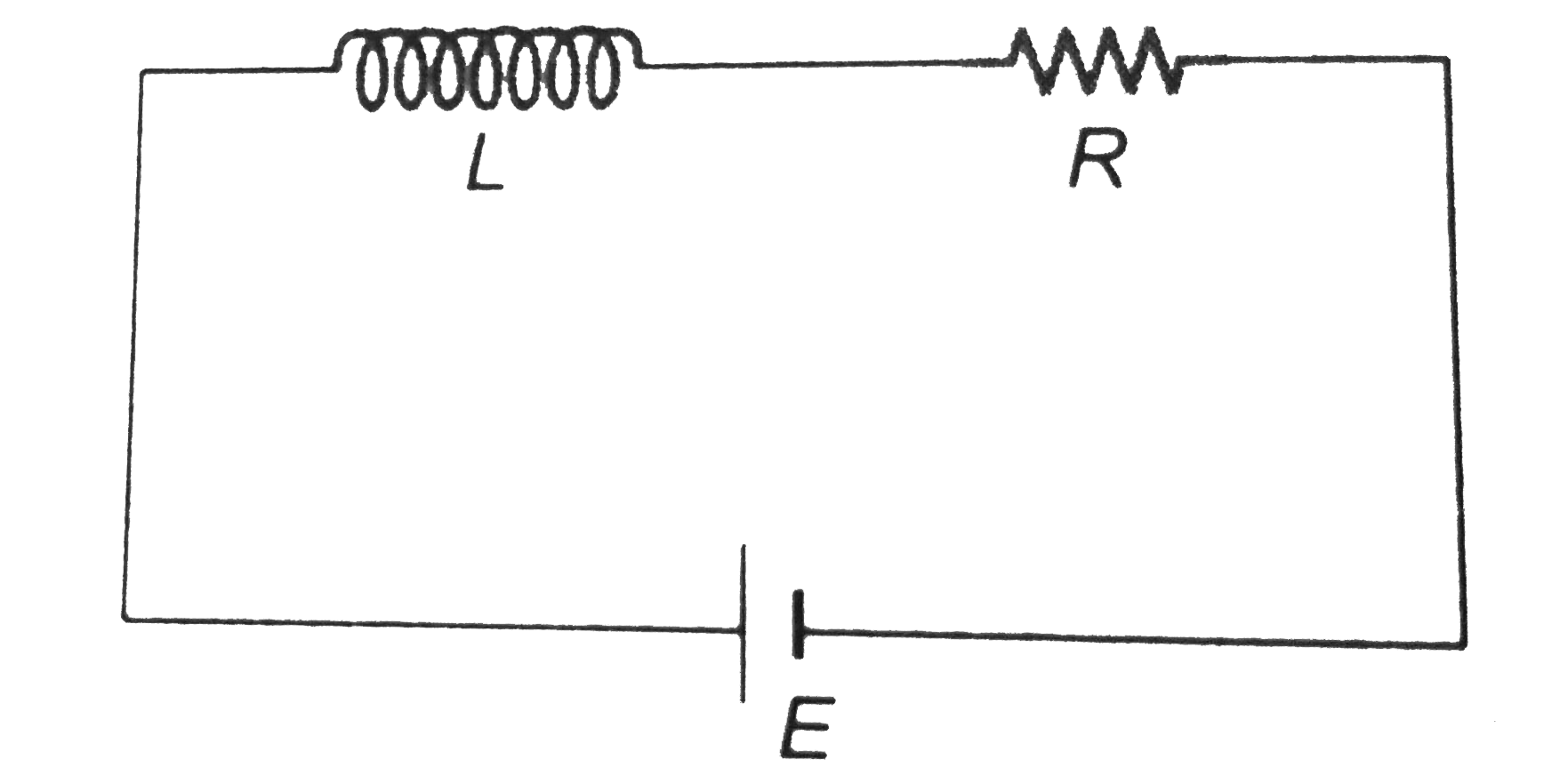
a. Current as a function of time assuming t=0 at the instant when piece is pulled.
b. the work done to pull out the piece.
c. thermal power generated in the circuit as as function of time.
d. power supplied by the battery as a function of time.
HOW TO PROCEED When the inductance of an inductor is abruptly changed, the flux passing through it remains constant.
ϕ= constant
∴Li= constant (L=ϕi)
a. At time t=0 steady state current in the circuit is i0=E/R. Suddenly ,L reduces to nL(n<1), so current in the circuit at time t=0 will increase to i0n=EnR. Let i be the current at time t.
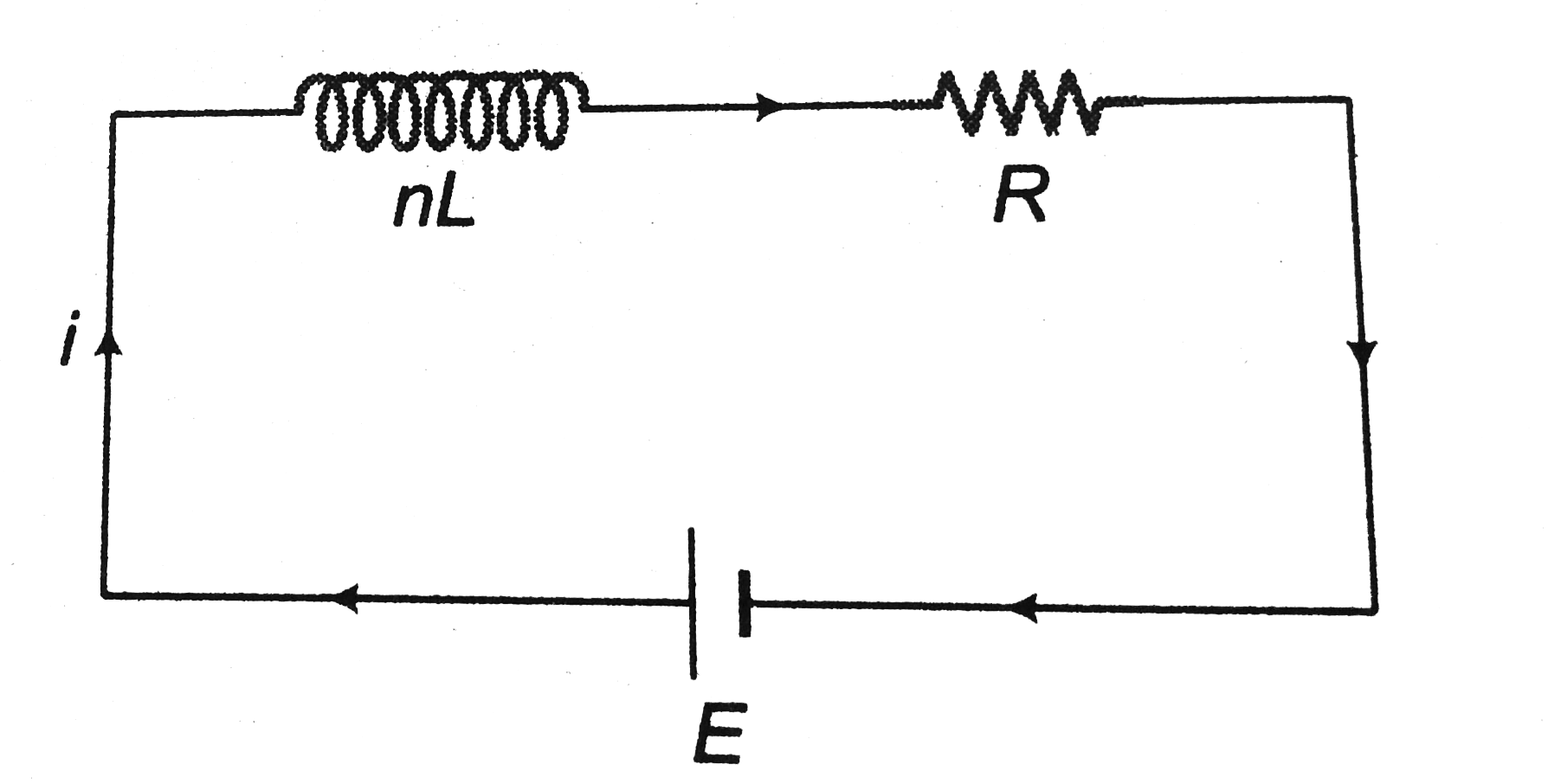
Applying Kirchoff's loop rule, we have
E−nL(didt)−iR=0
∴diE−iR=1nLdt
∴∫iio/ndiE=iR=1nL∫t0dt
solving this equation we get
i=i0−(i0−i0n)e−t/τL
Here, i0=ER
andτL=nLR
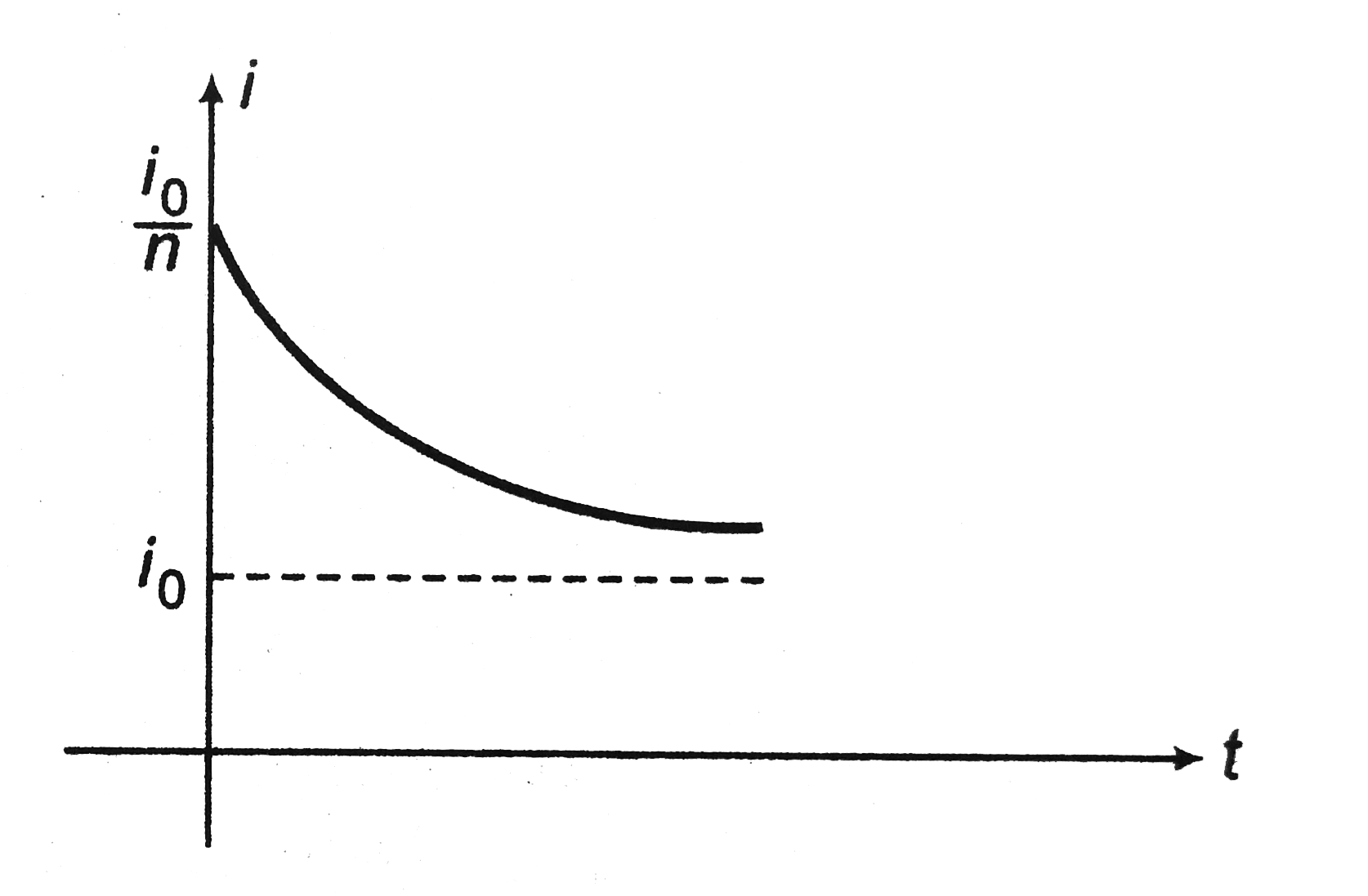
Form the i-t equation we get i=i0n att=0 and i=i0 at t=∞
The i−t graph is as known in figure.
At =0, current in the circuit is i0n. Current in the circuit in steady state will be again i0. So, it will decrease exponentially from i0n to i0. From the i−t graph, the equation can be fomed without doing any calculation.
 <span class=) <br>:. i=i_0+(i_0/n-i_0)e^(-t//tau_L)<br>b.workdo≠→pl–outtheπece<br>W=U_f-U_i=1/2L_fi_f^2-1/2L_(i)i_(i)^(2)<br>=(1)/(2)(nL) ((E)/(nR))^(2) -(1)/(2)(L) ((E)/(R))^(2)<br>=1/2L(E/R)^2(1/n-1)<br>=1/2L(E/R)^2((1-n)/n)<br>c.Thermalpower≥≠rated∈the∘uitasafunctionoftimeis<br>P_1=i^2R<br>Hereisthecurrentcalca––ted∈parta.<br>d.Power⊃pliedbythebaeryasafunctionoftimeis<br>P_2=Ei
<br>:. i=i_0+(i_0/n-i_0)e^(-t//tau_L)<br>b.workdo≠→pl–outtheπece<br>W=U_f-U_i=1/2L_fi_f^2-1/2L_(i)i_(i)^(2)<br>=(1)/(2)(nL) ((E)/(nR))^(2) -(1)/(2)(L) ((E)/(R))^(2)<br>=1/2L(E/R)^2(1/n-1)<br>=1/2L(E/R)^2((1-n)/n)<br>c.Thermalpower≥≠rated∈the∘uitasafunctionoftimeis<br>P_1=i^2R<br>Hereisthecurrentcalca––ted∈parta.<br>d.Power⊃pliedbythebaeryasafunctionoftimeis<br>P_2=Ei
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercise 27.1
8 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercise 27.2
4 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Example Type 6
3 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Medical entrances gallery
97 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
Similar Questions
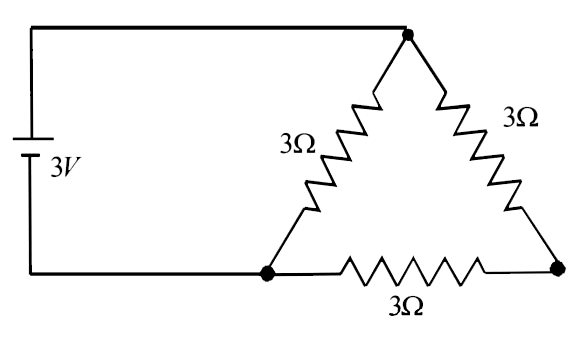
A 3 volt battery with negligible internal resistance is connected in a circuit as shown in the figure. The current I, in the circuit will be
View SolutionA battery of emf 2 V and initial resistance 1Ω is
connected across terminals A and B of the circuit shown
in figure
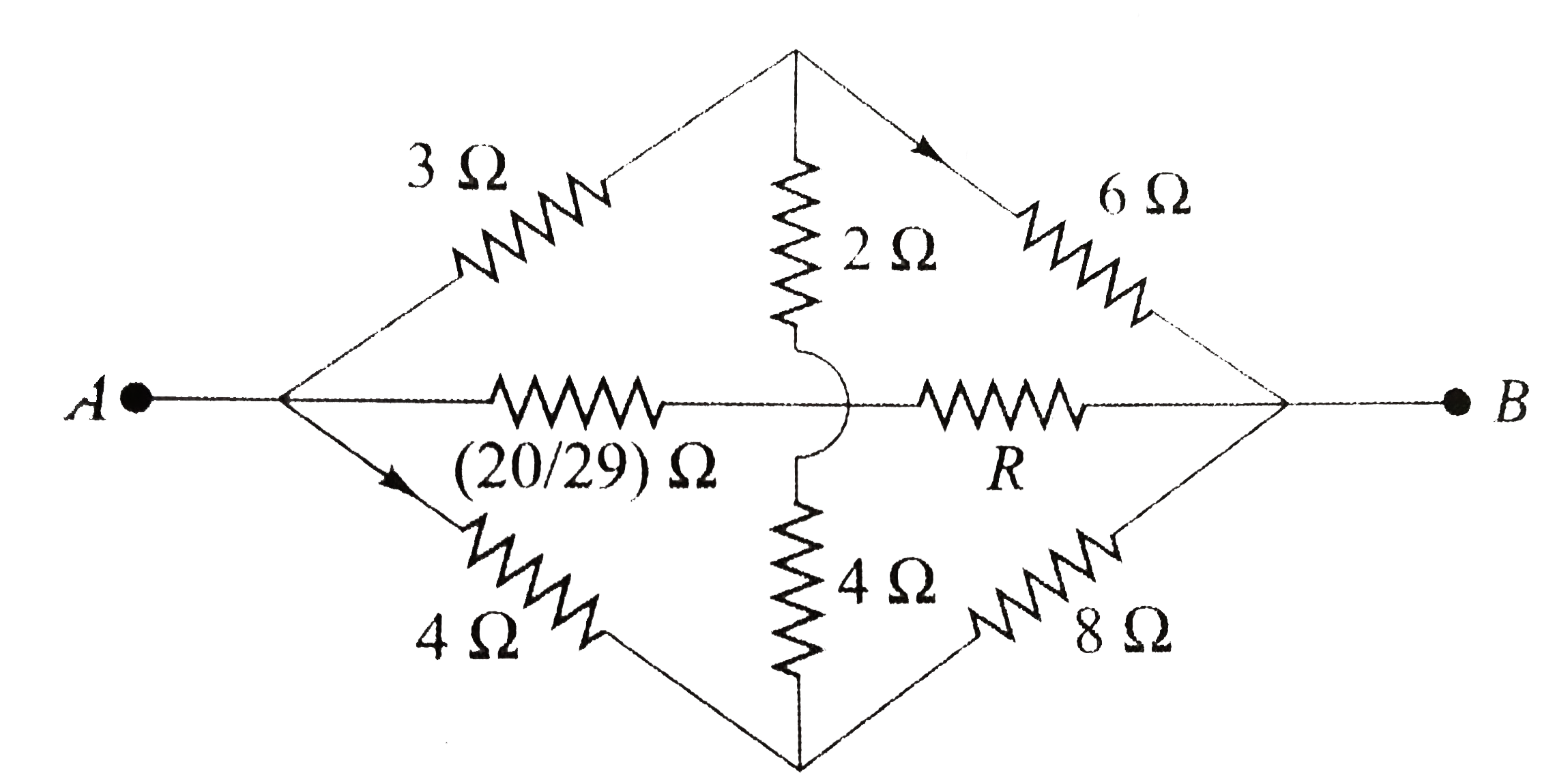 .
.
View SolutionA solenoid of resistance R and inductance L has a piece of soft iron inside it. A battery of emf E and of negligible internal resistance is connected across the solenoid as shown in Fig. At any instant, the piece of soft iron is pulled out suddenly so that inductance of the solenoid decrease to ηL(η<1) with battery remaining connected.
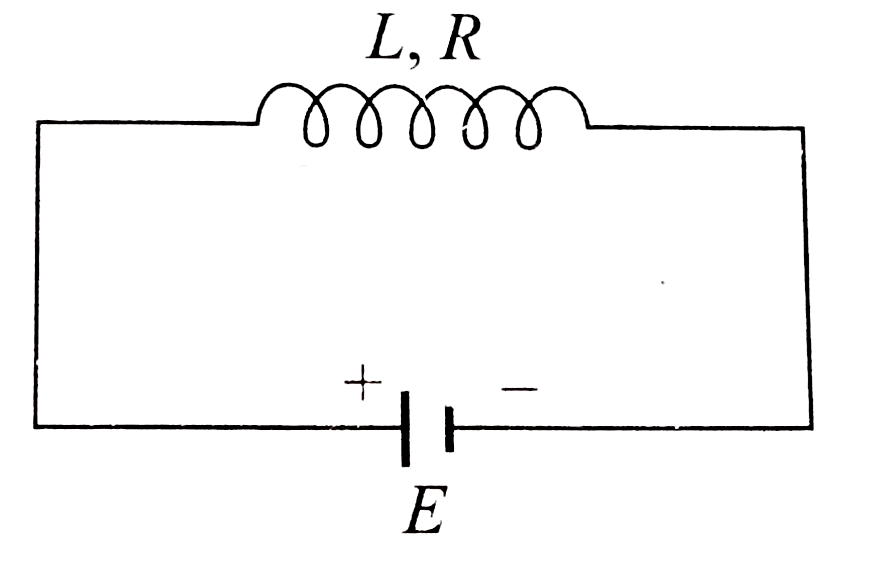
Power supplied by the battery as a function of time
View SolutionA solenoid of resistance R and inductance L has a piece of soft iron inside it. A battery of emf E and of negligible internal resistance is connected across the solenoid as shown in Fig. At any instant, the piece of soft iron is pulled out suddenly so that inductance of the solenoid decrease to ηL(η<1) with battery remaining connected.
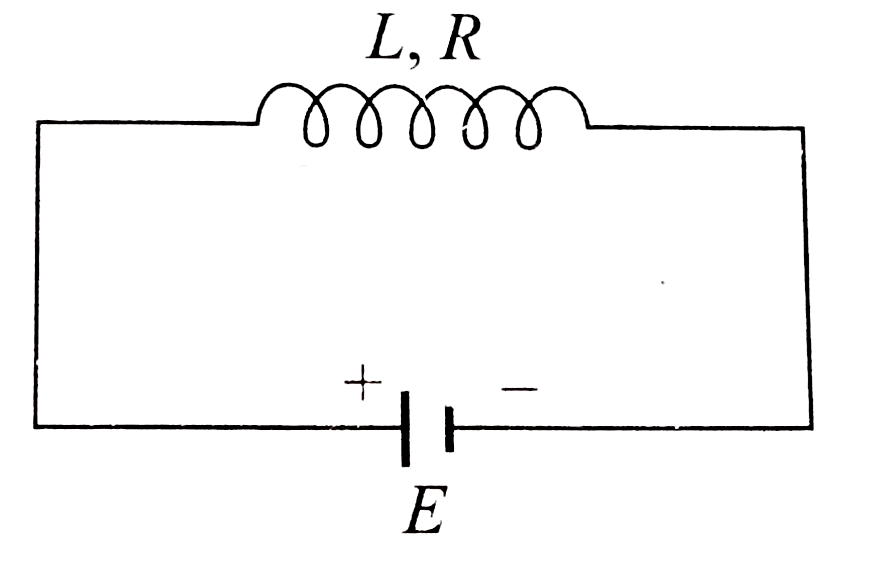
The work done to pull out the soft iron piece is
View SolutionPieces of soft iron used for the storage of magnets
View SolutionA solenoid of resistance R and inductance L has a piece of soft iron inside it. A battery of emf E and of negligible internal resistance is connected across the solenoid as shown in Fig. At any instant, the piece of soft iron is pulled out suddenly so that inductance of the solenoid decrease to ηL(η<1) with battery remaining connected.
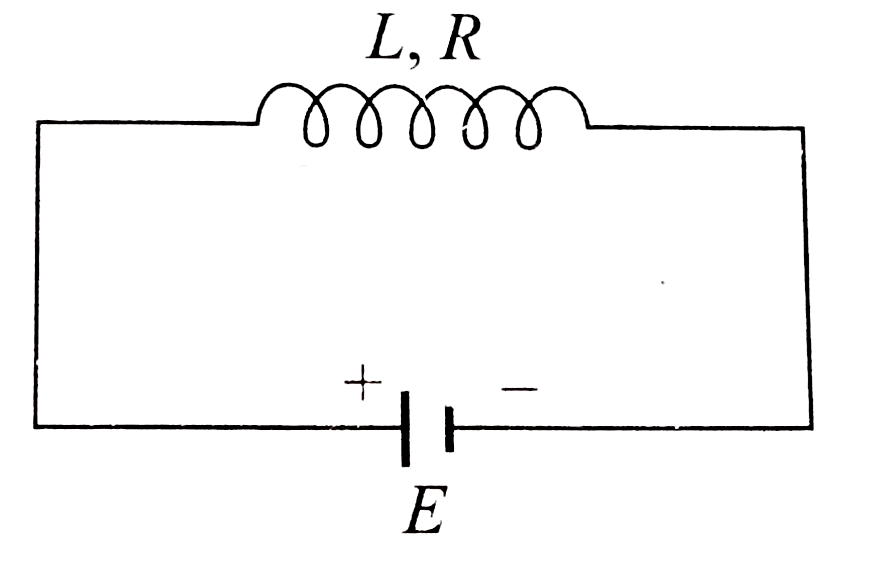
Assume t = 0 is the instant when iron piece has been pulled out, the current as a function of time after this is
View SolutionA 5 V battery of negligible internal resistance is connected across a 200 V battery and a resistance of 39Ω as shown in the figure. Find the value of the current.

View SolutionA 10 V battery of negilgible internal resistance is connected across a 200V battery and a resistance of 38Ω as shown in figure.
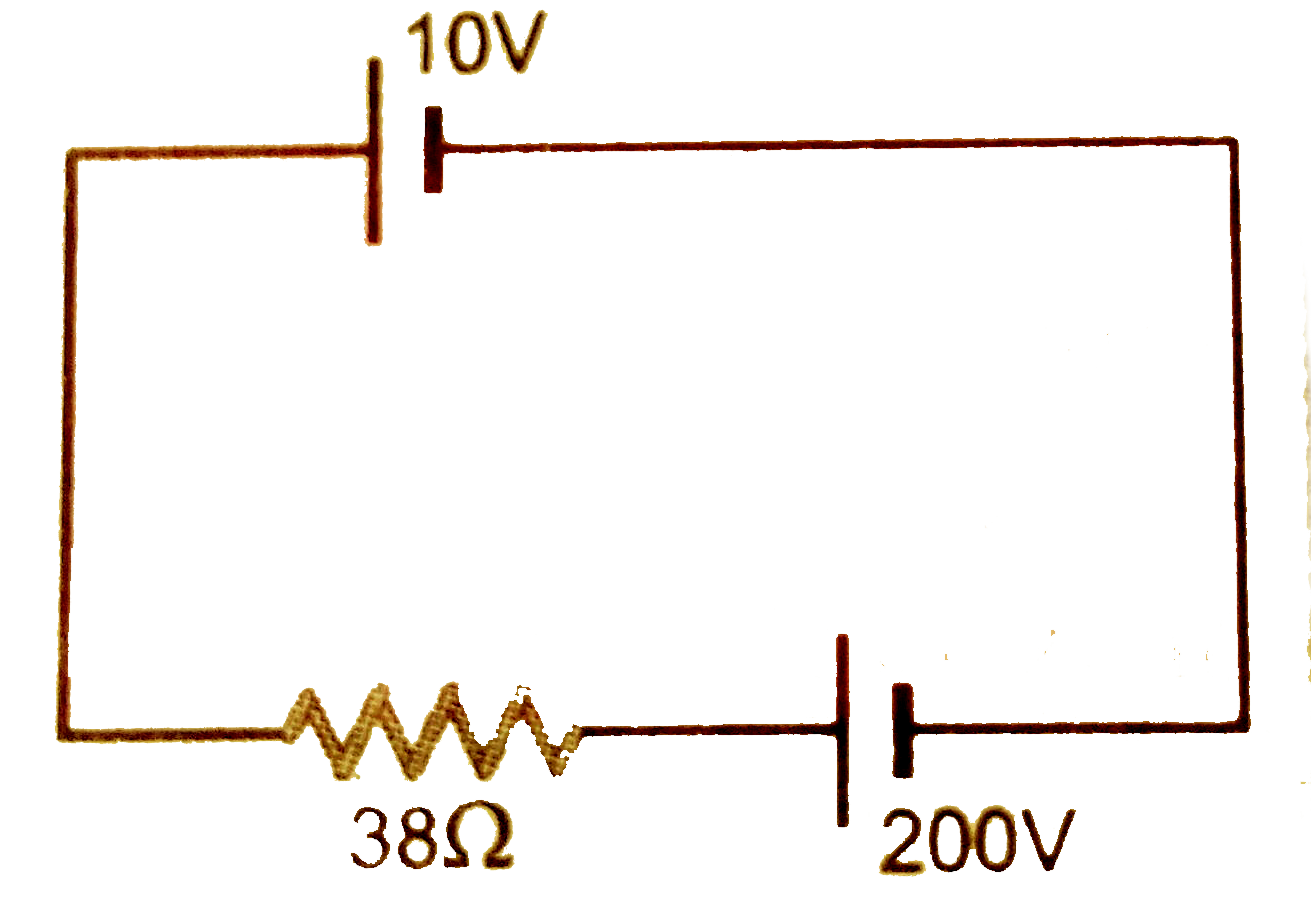 . Find the value of current in the circuit.
. Find the value of current in the circuit.
View SolutionA battery of emf 2V and internal resistance 0.5(Ω)is connected across a resistance 1.5(Ω). Find the current flow through battery ?
View SolutionTwo batteries of emf 4 V and 8 V with internal resistances 1Ω and 2Ω are connected in a circuit with a resistance of 9Ω as shown in figure. The current and potential difference between the points P and Q are
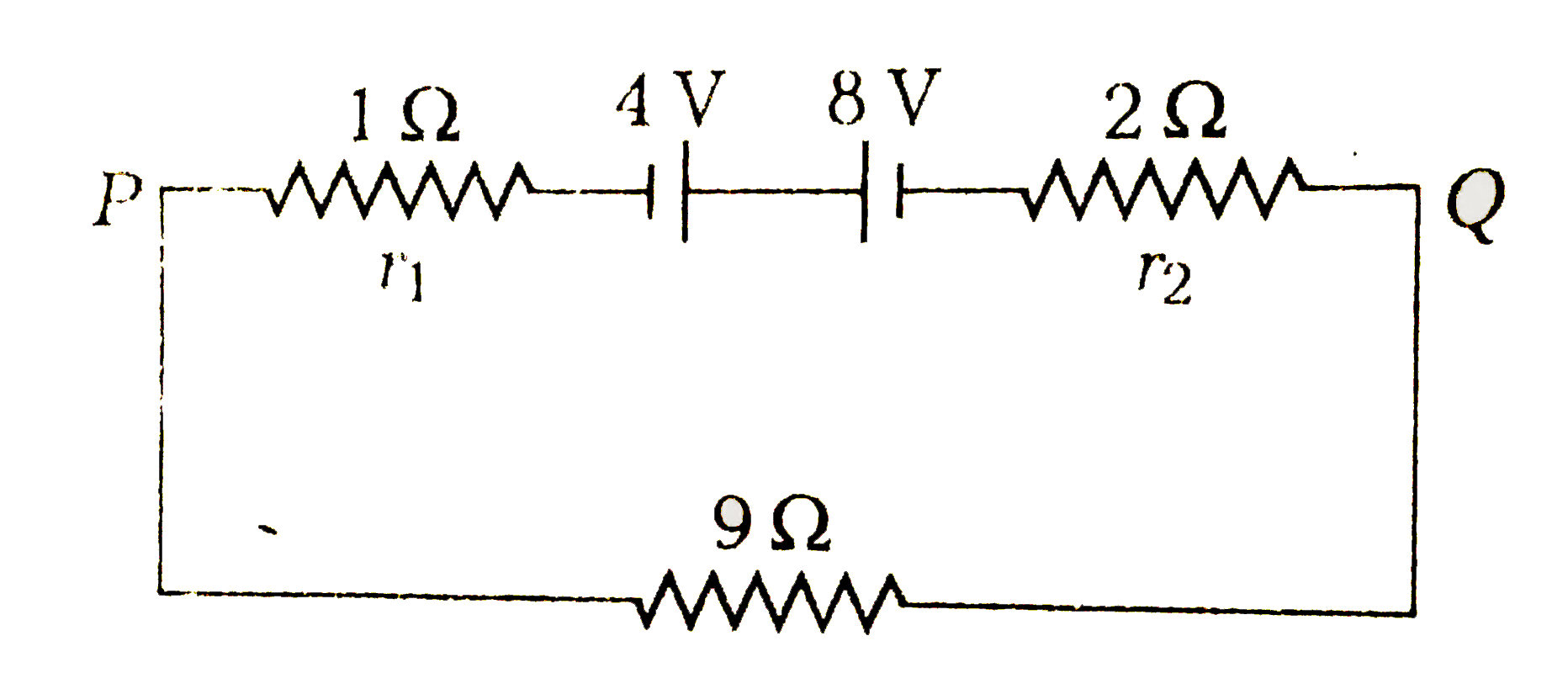
View Solution
