An electron has velocity . Magnetic field present in the region is .
(a) Find the force on electron.
(b) Repeat your calculation for a proton having the same velocity.
The correct Answer is:A, B, D
To solve the problem, we will break it down into two parts: (a) finding the force on the electron and (b) finding the force on the proton.
Part (a): Force on the Electron
1. Identify the Given Values:
- Velocity of the electron:
v=(2.0×106m/s)^i+(3.0×106m/s)^j
- Magnetic field:
B=(0.030T)^i−(0.15T)^j
- Charge of the electron:
q=−1.6×10−19C
2. Calculate the Cross Product v×B:
- Set up the determinant for the cross product:
v×B=∣∣
∣
∣∣^i^j^k2.0×1063.0×10600.030−0.150∣∣
∣
∣∣
- Calculate the determinant:
v×B=^i(3.0×106⋅0−0⋅(−0.15))−^j(2.0×106⋅0−0⋅0.030)+^k(2.0×106⋅(−0.15)−3.0×106⋅0.030)
=0^i−0^j+^k(−0.30×106−0.09×106)
=^k(−0.39×106)
3. Calculate the Force on the Electron:
- The magnetic force F on the electron is given by:
F=q(v×B)
- Substituting the values:
F=−1.6×10−19⋅(−0.39×106)^k
=6.24×10−14^kN
Part (b): Force on the Proton
1. Identify the Given Values:
- The velocity of the proton is the same as that of the electron.
- Charge of the proton:
q=+1.6×10−19C
2. Calculate the Force on the Proton:
- Using the same cross product calculated earlier:
F=q(v×B)
- Substituting the values:
F=1.6×10−19⋅(−0.39×106)^k
=−6.24×10−14^kN
Final Answers:
- (a) The force on the electron is:
Felectron=6.24×10−14^kN
- (b) The force on the proton is:
Fproton=−6.24×10−14^kN
- Velocity of the electron:
v=(2.0×106m/s)^i+(3.0×106m/s)^j
- Magnetic field:
B=(0.030T)^i−(0.15T)^j
- Charge of the electron:
q=−1.6×10−19C
- Set up the determinant for the cross product:
v×B=∣∣
∣
∣∣^i^j^k2.0×1063.0×10600.030−0.150∣∣
∣
∣∣
v×B=^i(3.0×106⋅0−0⋅(−0.15))−^j(2.0×106⋅0−0⋅0.030)+^k(2.0×106⋅(−0.15)−3.0×106⋅0.030)
=0^i−0^j+^k(−0.30×106−0.09×106)
=^k(−0.39×106)
- The magnetic force F on the electron is given by:
F=q(v×B)
- Substituting the values:
F=−1.6×10−19⋅(−0.39×106)^k
=6.24×10−14^kN
- The velocity of the proton is the same as that of the electron.
- Charge of the proton:
q=+1.6×10−19C
- Using the same cross product calculated earlier:
F=q(v×B)
- Substituting the values:
F=1.6×10−19⋅(−0.39×106)^k
=−6.24×10−14^kN
- (a) The force on the electron is:
Felectron=6.24×10−14^kN
- (b) The force on the proton is:
Fproton=−6.24×10−14^kN
Topper's Solved these Questions
- MAGNETICSBook:DC PANDEY ENGLISHChapter:MAGNETICSExercise:SCQ_TYPE
Explore 5 Videos
- MAGNETICSBook:DC PANDEY ENGLISHChapter:MAGNETICSExercise:MCQ_TYPE
Explore 1 Video
- MAGNETICSBook:DC PANDEY ENGLISHChapter:MAGNETICSExercise:SUBJECTIVE_TYPE
Explore 1 Video
- MAGNETIC FIELD AND FORCESBook:DC PANDEY ENGLISHChapter:MAGNETIC FIELD AND FORCESExercise:Medical entrance s gallery
Explore 59 Videos
- MAGNETISM AND MATTERBook:DC PANDEY ENGLISHChapter:MAGNETISM AND MATTERExercise:Medical gallery
Explore 1 Video
Similar Questions
An object has a velocity, v=(2ˆi+4ˆj)ms−1 at time t=0s. It undergoes a constant acceleration a=(ˆi−3ˆj)ms−2 for 4s. Then
(i) Find the coordinates of the object if it is at origin at t=0
(ii) Find the magnitude of its velocity at the end of 4s.
View SolutionAt some instant the velocity components of an electron moving between two charged parallel plates are vx=1.5×105m/s and vy=3.0×106m/s.Suppose that the electric field between the plates is given by E=(120N/C)ˆj
. (a) What is the acceleration of the electron?
(b) What will be the velocity of the electron after its x-coordinate has changed by 2.0 cm?
View Solution
Knowledge Check
An electron is moving with an initial velocity →v=v0ˆi and is in a magnetic field →B=B0ˆj. Then it's de-Broglie wavelength
Aremains constantBincreases with timeCdecreases with timeDincreases and decreases periodically
A particle with charge 7.80μC is moving with velocity v=−(3.80×103ms)ˆj. The magnetic force on the particle is measured to be F=+(7.60×10−3N)ˆi−(5.20×10−3N)ˆk
(a) Calculate the components of the magnetic field you can find from this information.
(b) Are the components of the magnetic field that are not determined by the measurement of the force? Explain.
(c) Calculate the scalar product B.F. What is the angle between B and F
View SolutionThe velocity of an object is given by →v=(6t3ˆi+t2ˆj)m/s) . Find the acceleration at t = 2s.
View SolutionThe velocity of an object is given by →v=(6t3ˆi+t2ˆj)m/s . Find the acceleration at t = 2s.
View SolutionAn electron is moving with an initial velocity →v=v0ˆi and is in a magnetic field →B=B0ˆj. Then it's de-Broglie wavelength
View SolutionA proton moves at a constant velocity of 50ms along the x-axis, in uniform electric and magnetic fields. The magnetic field is B=(2.0mT)ˆj. What is the electric field?
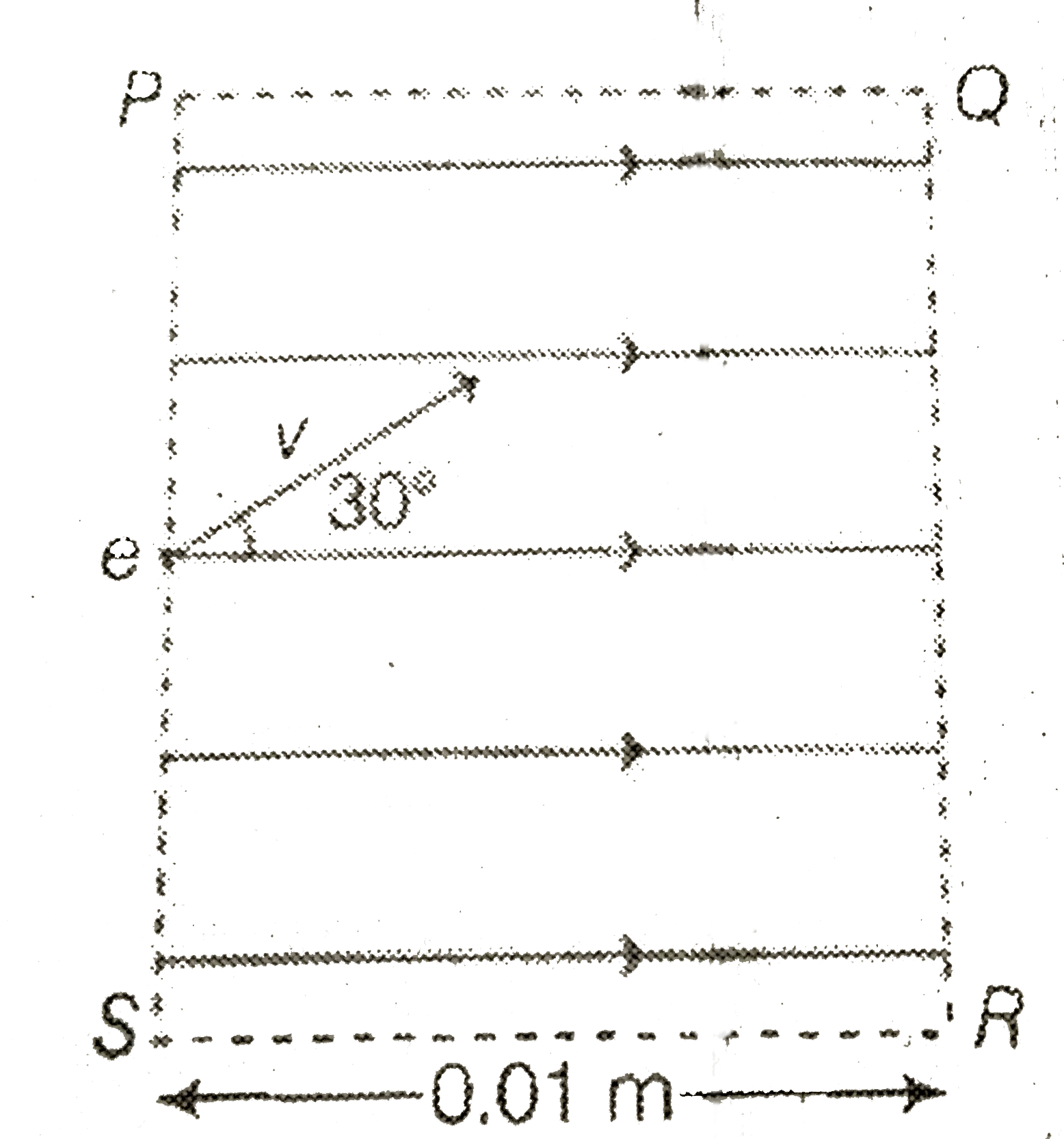
View SolutionRefer to the figure. Rectangle PQRS represents the cross section of a uniform magnetic field region of 0.20 T. An electron is projected at a speed of V=2.0×106m/s into the region at an angle of 30∘ to the direction of the mangetic field. The length of the magnetic field region is 0.01 m. Find the number of revolutions made by the electron before it leaves the magnetic field region.
View SolutionElectric field in a region is given by E=(2ˆi+3ˆj−4ˆk)V/m. Find the potential difference between points (0, 0, 0) and (1,2,3) in this region.
View Solution
