A horizontal rod long is mounted on a balnce and carries a current. At the location of the rod a uniform horizontal magnetic field has magnitude and direction perpendicular to the rod. The magnetic force on the rod is measured by the balance and is found to be . What is the current?
To solve the problem, we will use the formula for the magnetic force acting on a current-carrying conductor in a magnetic field. The formula is given by:
F=I⋅L⋅B⋅sin(θ)
Where:
- F is the magnetic force (in Newtons),
- I is the current (in Amperes),
- L is the length of the rod (in meters),
- B is the magnetic field strength (in Teslas),
- θ is the angle between the direction of the current and the magnetic field.
1. Identify the given values:
- Length of the rod, L=0.2m
- Magnetic field strength, B=0.067T
- Magnetic force, F=0.13N
- Angle θ=90∘ (since the magnetic field is perpendicular to the rod)
2. Substitute the values into the formula:
Since sin(90∘)=1, the formula simplifies to:
F=I⋅L⋅B
Thus, we can write:
0.13=I⋅0.2⋅0.067
3. Rearranging the equation to solve for current I:
I=FL⋅B
4. Plug in the known values:
I=0.130.2⋅0.067
5. Calculate the denominator:
0.2⋅0.067=0.0134
6. Now calculate the current I:
I=0.130.0134≈9.7A
Final Answer:
The current in the rod is approximately 9.7A.
---
- F is the magnetic force (in Newtons),
- I is the current (in Amperes),
- L is the length of the rod (in meters),
- B is the magnetic field strength (in Teslas),
- θ is the angle between the direction of the current and the magnetic field.
- Length of the rod, L=0.2m
- Magnetic field strength, B=0.067T
- Magnetic force, F=0.13N
- Angle θ=90∘ (since the magnetic field is perpendicular to the rod)
Since sin(90∘)=1, the formula simplifies to:
F=I⋅L⋅B
Thus, we can write:
0.13=I⋅0.2⋅0.067
I=FL⋅B
I=0.130.2⋅0.067
0.2⋅0.067=0.0134
I=0.130.0134≈9.7A
The current in the rod is approximately 9.7A.
Topper's Solved these Questions
- MAGNETICSBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETICSEXERCISE - INTRODUCTORY EXERCISE
1 Videos
- MAGNETICSBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETICSEXERCISE - SUBJECTIVE_TYPE
1 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Medical entrance s gallery
59 Videos
- MAGNETISM AND MATTERBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETISM AND MATTEREXERCISE - Medical gallery
1 Videos
Similar Questions
A semicircular wire of radius 5.0 cm carries a current of 5.0 A. A magnetic field B of magnitude 0.50 T exists along the perpendicular to the plane of the wire. Find the magnitude of the magnetic force acting on the wire.
View SolutionA 20 cm long conducting rod is set into pure translation with a uniform velocity of 10cm s ^(-1) perpendicular to its length. A uniform magnetic field of magnitude 0.10 T exists in a direction perpendicular to the plane of motion. Find emf developed in the rod.
View Solution
Knowledge Check
The magnetic force per unit length on a wire carrying a current of 10 A and making an angle of 45∘ with the direction of a uniform magnetic field of 0.20 T is
A2√2Nm−1B2√2Nm−1C√22Nm−1D4√2Nm−1
A rod of mass 0.720 kg and radius 6 cm rests on two parallel rails that are d=12cm apart and length of rails is L=49cm. The rod carried a current of I=48A Fig. and rolls along the rails without slipping. A uniform magnetic field of magnetude 0.240 T is directed perpendicular to the rod and the rails. If it starts from rest, what is the speed of the rod as it leaves the rails?

View SolutionThe moment of inertia of a uniform rod of mass 0.50 kg and length 1 m is 0.10 kg m^2 about a line perpendicular to the rod. Find the distance of this line from the middle point of the rod.
View SolutionA metal rod moves at a constant velocity in a direction perpendicular to its length. A constant, uniform magnetic field exists in space in a direction perpendicular to the rod as well as its velocity. Select the correct statements(s) from the following
View SolutionA conducting rod of mass m and length l is placed over a smooth horizontal surface. A uniform magnetic field B is acting perpendicular to the rod. Charge q is suddenly passed through the rod and it acquires an initial velocity v on the surface, then q is equal to
View SolutionA square of side 2.0m is placed in a uniform magnetic field B=2.0T in a direction perpendicular to the plane of the square inwards. Equal current i=3.0A is flowing in the directions shown in figure. Find the magnitude of magnetic force on the loop.
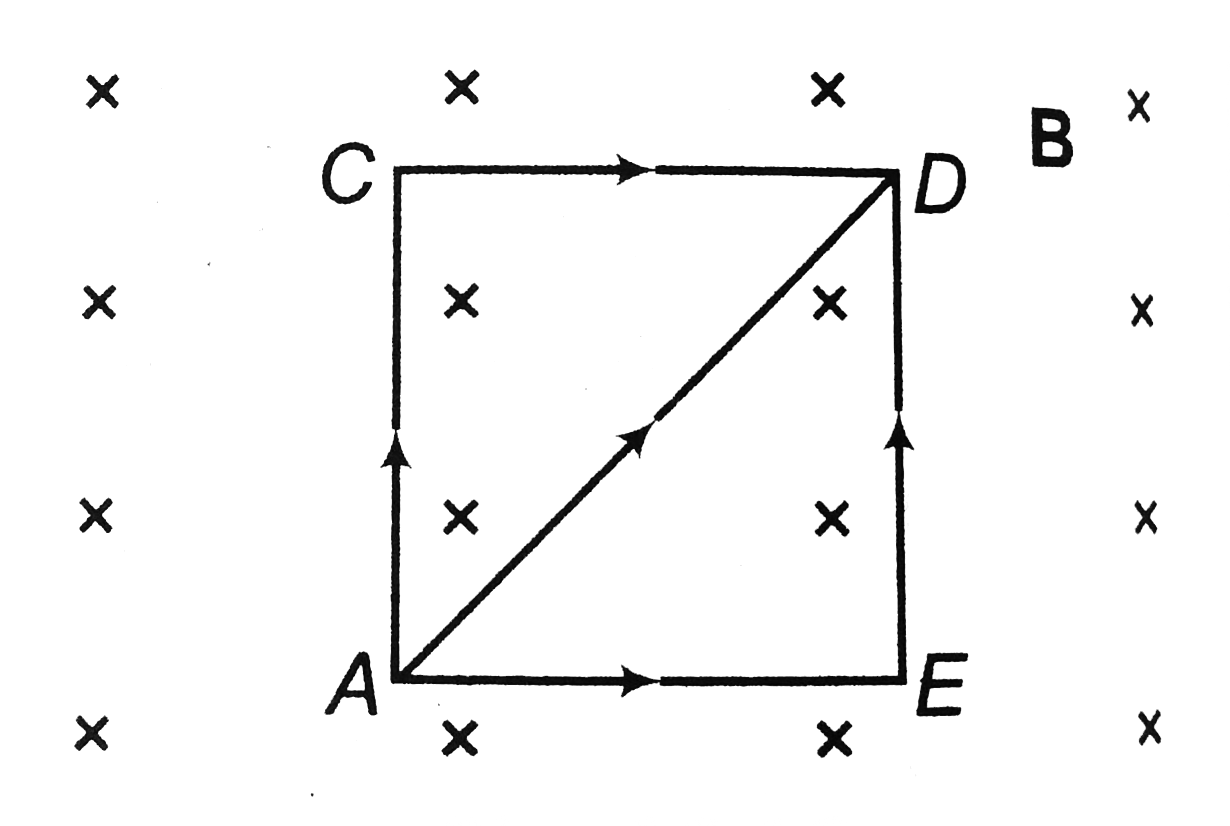
View SolutionA square of side 2.0m is placed in a uniform magnetic field B=2.0T in a direction perpendicular to the plane of the square inwards. Equal current i=3.0A is flowing in the directions shown in figure. Find the magnitude of magnetic force on the loop.

View SolutionA uniform rod of mass M and length L carrying a charge q uniformly distributed over its length is rotated with constant velocity w about its mid point perpendicular to the rod. Its magnetic moment is
View Solution
