Find Vba, if 12J of work has to be done against an electric field to take a charge of 10−2 C from a to b.
The correct Answer is:A, B, C
To find the potential difference Vba when 12J of work is done against an electric field to move a charge of 10−2C from point A to point B, we can follow these steps:
Step 1: Understand the relationship between work, charge, and potential difference.
The work done W in moving a charge Q through a potential difference Vba is given by the formula:
W=Q⋅Vba
Where:
- W is the work done (in joules),
- Q is the charge (in coulombs),
- Vba is the potential difference between points B and A (in volts).
Step 2: Rearrange the formula to find Vba.
We can rearrange the formula to solve for Vba:
Vba=WQ
Step 3: Substitute the known values into the equation.
We know:
- W=12J
- Q=10−2C
Substituting these values into the equation gives:
Vba=12J10−2C
Step 4: Calculate Vba.
Now, perform the calculation:
Vba=1210−2=12×102=1200V
Final Answer:
Thus, the potential difference Vba is:
Vba=1200V
---
The work done W in moving a charge Q through a potential difference Vba is given by the formula:
W=Q⋅Vba
Where:
- W is the work done (in joules),
- Q is the charge (in coulombs),
- Vba is the potential difference between points B and A (in volts).
We can rearrange the formula to solve for Vba:
Vba=WQ
We know:
- W=12J
- Q=10−2C
Vba=12J10−2C
Now, perform the calculation:
Vba=1210−2=12×102=1200V
Thus, the potential difference Vba is:
Vba=1200V
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.6
2 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.7
2 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.4
4 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
Similar Questions
12 j of work has to be done against an existion electric field to take a charge of 0.01 C from A to B. How much is th potential difference VB−VA?
View Solutioncharges 2.0×10−6C and 1.0×10−6C are placed at. corners A and B of a squae of side 5.0cm as shown in. figure .how much work will be done against. the electric field in moving a charge of 1.0 xx 10^(-6) C from. C to D?.

View SolutionIf 20J of work has to be done to move an electric charge of 4C from a point, where potential is 10V to another point, where potential is V volt, find the value of V.
View SolutionFind the work done in taking a charge 100μC from C & D.
View SolutionIf 50 joule of work must be done to move an electric charge of 2 C from a point, where potential is V volt, the value of V is
View SolutionAn electric field 'E' whose direction is radially outward varies as distance from origin 'r' as shown in the graph E is taken as positive if its direction is away from the origin. Then the work done by electric field on a 2 C charge if it is taken from (1,1,0) to (3,0,0) is :
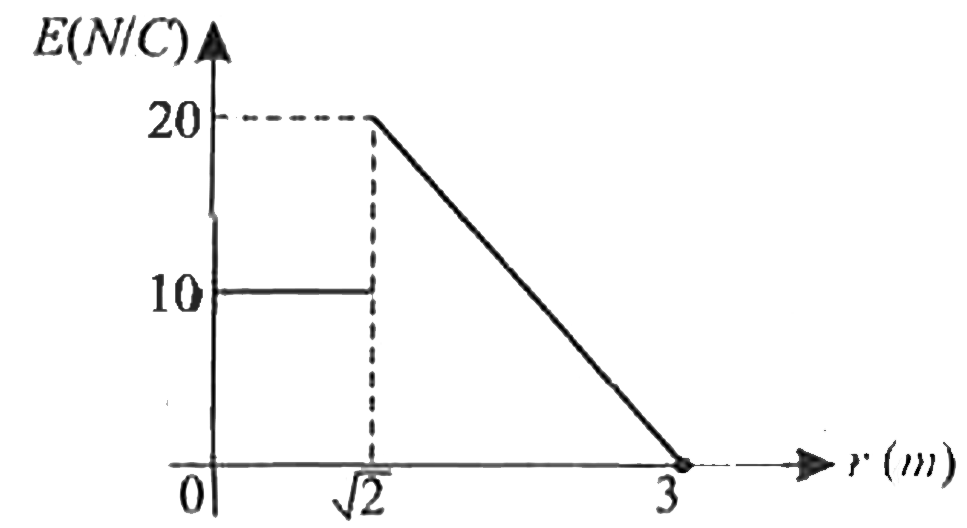
View SolutionA charge is moved in an electric field of a fixed charge distribution from point A to another point B slowly. The work done by external agent in doing so is 100J . What is the change in potential energy of the charge as it moves from A to B ? What is the work done by the electric field of the charge distribution as the charge moves from A to B ?
View SolutionA charge is moved in an electric field of a fixed charge distribution from point A to another point B slowly. The work done by external agent in doing so is 100J. What is the change in potential energy of the charge as it moves from A to B ? What is the work done by the electric field of the charge distribution as the charge moves from A to B ?
View SolutionA particle of mass 100 g is kept on the surface of a uniform sphere of mass 10 kg and radius 10 cm. Find the work to be done against the gravitational force between them to take the particle away from the sphere.
View SolutionA particle of mass 1kg is kept on the surface of a uniform sphere of mass 20kg and radius 1.0m. Find the work to be done against the gravitational force between them to take the particle away from the sphere.
View Solution

