A 2.0 V potentiometer is used to determine the internal resistance of 1.5V cell. The balance point of the cell in the circuit is 75cm. When a resistor of 10Ω is connected across cel, the balance point sifts to 60cm. The internal resistance of the cell is
1.5Ω
2.5Ω
3.5Ω
4.5Ω
The correct Answer is:B
To find the internal resistance of the 1.5 V cell using a potentiometer, we can follow these steps:
1. Understanding the Setup:
- We have a potentiometer with a total voltage of 2.0 V.
- The initial balance point with the 1.5 V cell is at 75 cm.
- When a 10 Ω resistor is connected across the cell, the balance point shifts to 60 cm.
2. Determine the EMF from the First Balance Point:
- The potential drop per unit length of the potentiometer wire can be denoted as V.
- The EMF of the cell can be expressed using the first balance point:
E=V×0.75(Equation 1)
3. Determine the Current with the Resistor Connected:
- When the 10 Ω resistor is connected, the current I flowing through the circuit can be calculated using Ohm's Law:
I = \frac{E}{r + 10} \quad \text{(where r is the internal resistance)}
4. Calculate the Potential Drop Across the Resistor:
- The potential drop across the 10 Ω resistor can be expressed as:
VAB=I×10=E×10r+10(Equation 2)
5. Relate the Second Balance Point to the Potential Drop:
- The new balance point at 60 cm gives us:
VAB=V×0.60
6. Setting Up the Equation:
- From Equation 2 and the new balance point, we have:
E×10r+10=V×0.60(Equation 3)
7. Dividing Equation 1 by Equation 3:
- We can divide Equation 1 by Equation 3 to eliminate V:
r+10r=7560=54
8. Cross Multiplying:
- Cross multiplying gives:
4(r+10)=5r
9. Solving for r:
- Expanding and rearranging:
4r+40=5r⟹5r−4r=40⟹r=40Ω
10. Final Answer:
- The internal resistance of the cell is:
r=2.5Ω
- We have a potentiometer with a total voltage of 2.0 V.
- The initial balance point with the 1.5 V cell is at 75 cm.
- When a 10 Ω resistor is connected across the cell, the balance point shifts to 60 cm.
- The potential drop per unit length of the potentiometer wire can be denoted as V.
- The EMF of the cell can be expressed using the first balance point:
E=V×0.75(Equation 1)
- When the 10 Ω resistor is connected, the current I flowing through the circuit can be calculated using Ohm's Law:
I = \frac{E}{r + 10} \quad \text{(where r is the internal resistance)}
- The potential drop across the 10 Ω resistor can be expressed as:
VAB=I×10=E×10r+10(Equation 2)
- The new balance point at 60 cm gives us:
VAB=V×0.60
- From Equation 2 and the new balance point, we have:
E×10r+10=V×0.60(Equation 3)
- We can divide Equation 1 by Equation 3 to eliminate V:
r+10r=7560=54
- Cross multiplying gives:
4(r+10)=5r
- Expanding and rearranging:
4r+40=5r⟹5r−4r=40⟹r=40Ω
- The internal resistance of the cell is:
r=2.5Ω
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - OBJECTIVE_TYPE
1 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Level 1 Subjective
53 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Level 1 Assertion And Reason
11 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Subjective
11 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Medical entrances gallery
25 Videos
Similar Questions
Figure 6.13 shows a 2.0V potentiometer used for the determination of internal resistance of a 1.5V cell. The balance point of the cell in open circuit is 76.3cm. Whan a resistor of 9.5Ω is used in the external circuit of the cell, the balance point shifts to 64.8cm, length of the potentiometer. Dentermine the internal resistance of the cell.
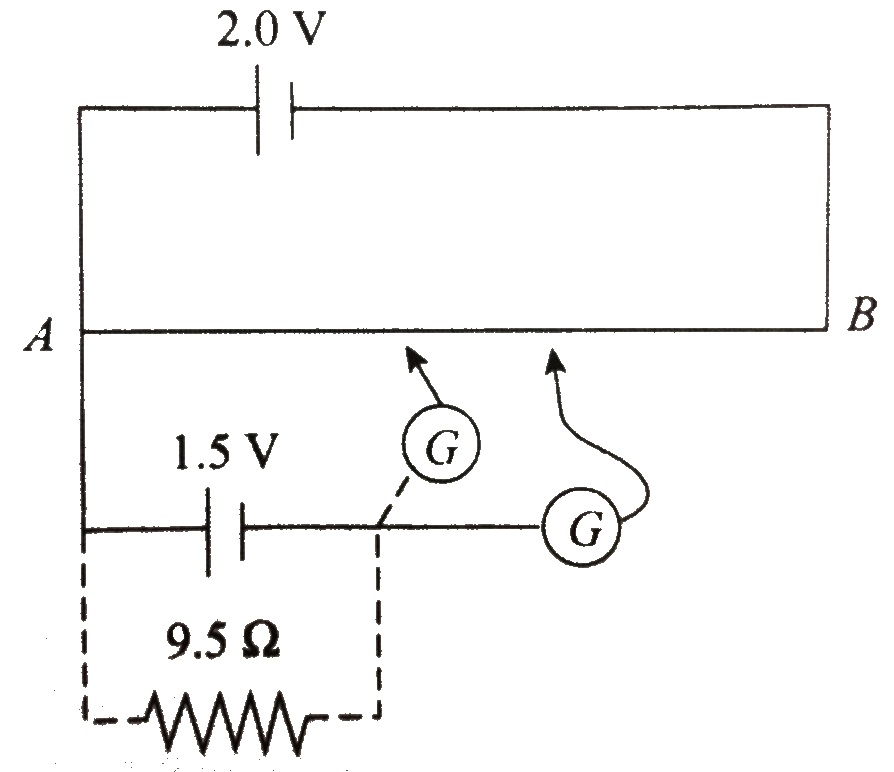
View SolutionIn a potentiometer arrangement for determining the emf of cell, the balance point of the cell in open circuit is 350 cm. When a resistance of 9Ω is used in the external circuit of cell , the balance point shifts to 300 cm. Determine the internal resistance of the cell.
View Solution
Knowledge Check
3V poteniometer used for the determination of internal resistance of a 2.4V cell. The balanced point of the cell in open circuit is 75.8cm. When a resistor of 10.2Ω is used in the external circuit of the cell the balance point shifts to 68.3cm length of the potentiometer wire. The internal resistance of the cell is
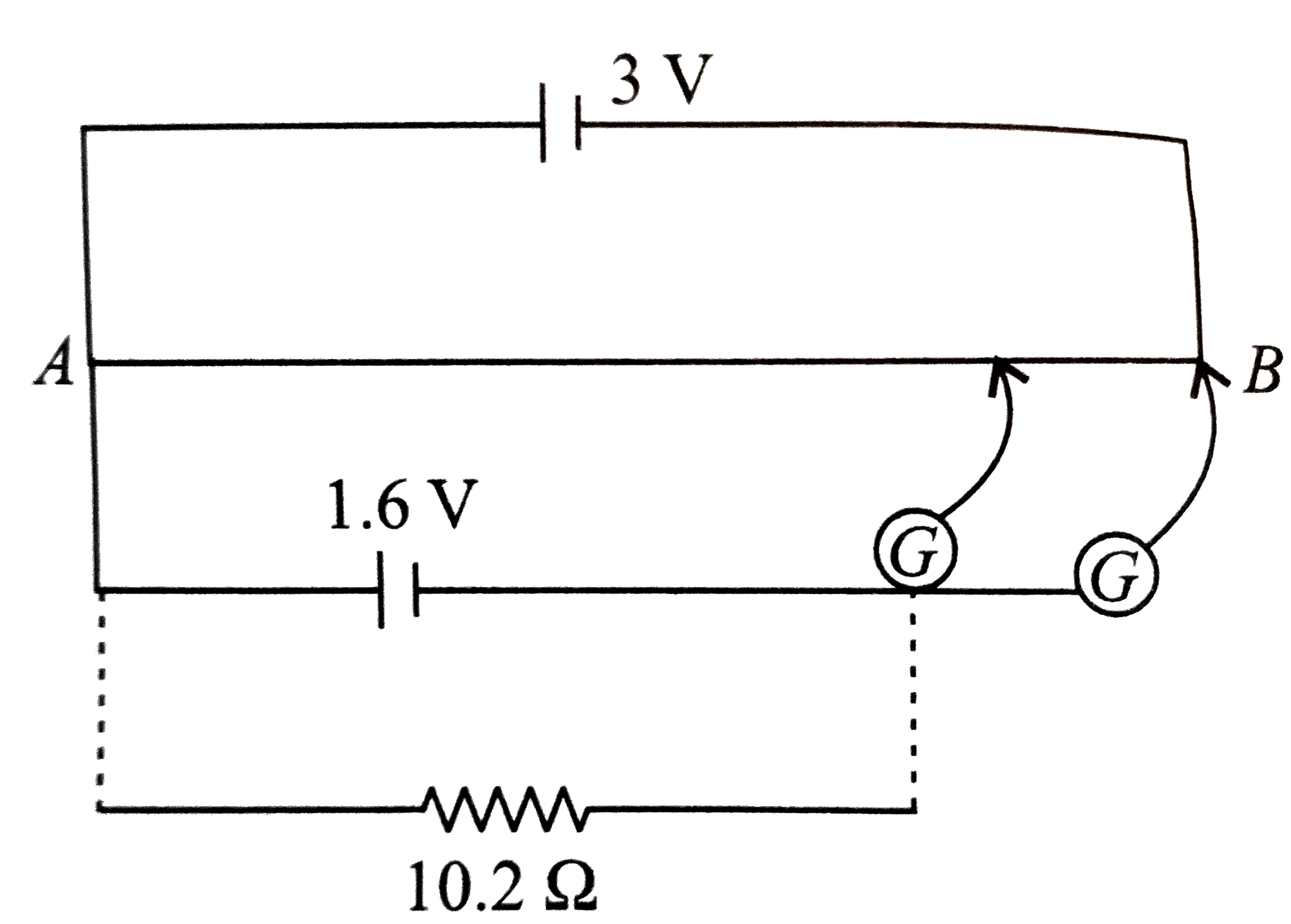 A2.5ΩB2.25ΩC1.12ΩD3.2Ω
A2.5ΩB2.25ΩC1.12ΩD3.2Ω
In an experiment to determine the internal resistance of a cell with potentiometer, the balancing length is 165 cm. When a resistance of 5 ohm is joined in parallel with the cell the balancing length is 150 cm. The internal resistance of cell is
View SolutionThe e.m.f. of a standard cell balances across 150 cm length of a wire of potentiometer. When a resistance of 2Ω is connected as a shunt with the cell, the balance point is obtained at 100cm . The internal resistance of the cell is
View SolutionIn a potentiometer experiment the balancing length with a cell is 560 cm. When an external resistance of 10Ω is connected in parallel to the cell, the balancing length changes by 60 cm. Find the internal resistance of the cell.
View SolutionA battery is connected to a potentiometer and a balance point is obtained at 84cm along the wire. When its terminals are connected by a 5Ω resistor, the balance point changes to 70cm.
Calculate the internal resistance of the cell.
View SolutionA Daniel cell is balanced on 125cm length of a potentiometer wire. Now the cells is short-circuited by a resistance 2ohm and the balance is obtained at 100cm. The internal resistance of the Dainel cell is
View SolutionIn an experiment with a potentiometer, the null point is
obtained at a distance of 60cm along the wire from the
common terminal with a leclanche cell. When a shunt
resistance of 1Ω is connected across the cell, the null
point shifts to a distance of 30cm from the common
terminal. what is the internal resistance of the cell?
View SolutionIn a potentiometer experiment the balancing with a cell is at length 240 cm. On shunting the cell with a resistance of 2Ω, the balancing length becomes 120 cm.The internal resistance of the cell is
View Solution
