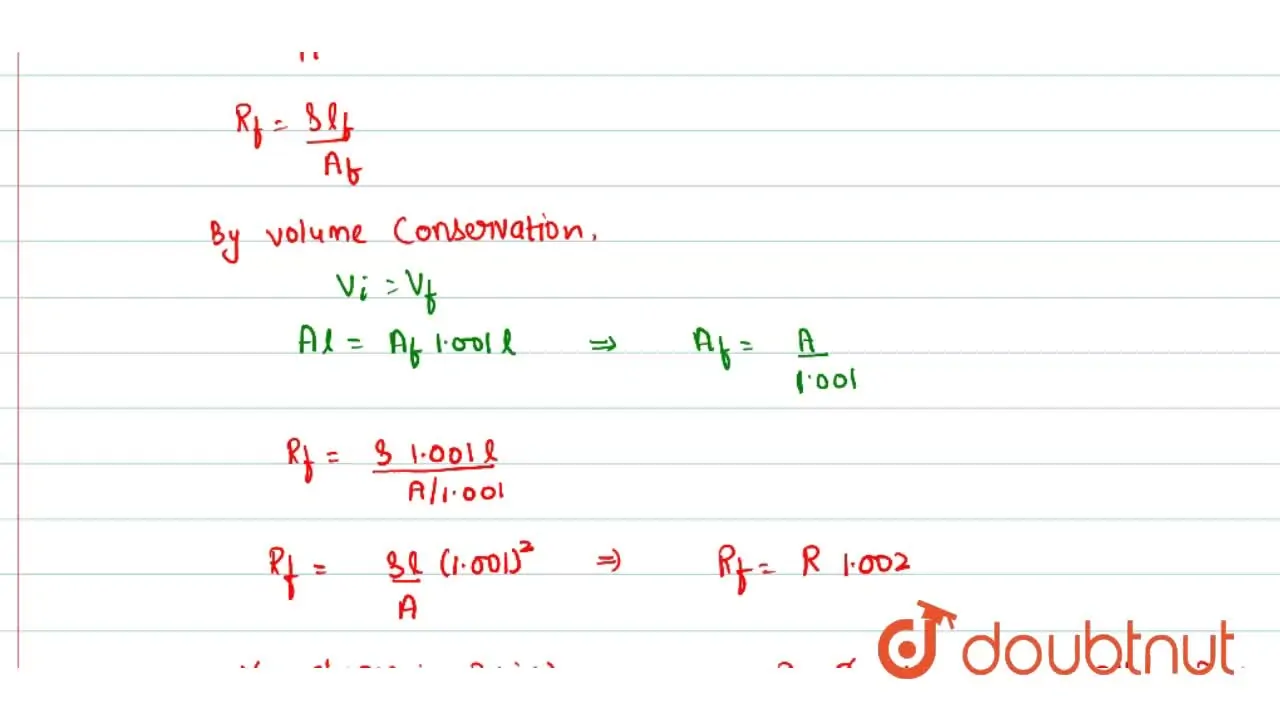
A copper wire iis stretched to make it 0.1% longer. What is the percentage change in its resistance?
R=ρ1A=ρlVl (V= volume of wire)
=ρl2V
∴R∝l2 (ρandV= constant)
For small percentage change
% change R=2(%change inl)=2(0.1%)=0.2%
Since R∝l2, with increase in the value of l, resistance will also increase.
Related Playlists
- BOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Example Type 12videos
CURRENT ELECTRICITY
- BOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Example Type 21videos
CURRENT ELECTRICITY
- BOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Subjective11videos
COMMUNICATION SYSTEM
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Medical entrances gallery25videos
ELECTROMAGNETIC INDUCTION
Similar Questions
If a copper wire is stretched to make it 0.1% longer what is the percentage change in its resistance?
View SolutionA copper wire is stretched to make it 0.5% longer. The percentage change in its electrical resistance if its volume remains unchanged is :
View SolutionA copper wire is stretched to make it 0.5% longer. The percentage change in its electrical resistance if its volume remains unchanged is :
View SolutionA copper wire is stretched to make it 0.5% longer. The percentage change in its electrical resistance if its volume remains unchanged is :
View SolutionIf a copper wire is stretched to make it 0.1% longer, then the percentage change in resistance is approximately -
View SolutionThe wire is stretched to increase the length by 1%. Find the percentage change in the resistance.
View SolutionIf a copper wire is stretched to make its radius decrease by 0.1%, then the percentage increase in resistance is approximately -
View SolutionA cylindrical wire is stretched to increase its length by 10%. Calculate the percentage increase in resistance.
View SolutionThe area of cross section of a uniform cylindrical conducting wire is decreased by 20% due to stretching it. The percentage change in its resistance is (approximately)
View SolutionA wire is stretched to n times its length. Then the resistance now will be increase by
View Solution