Question

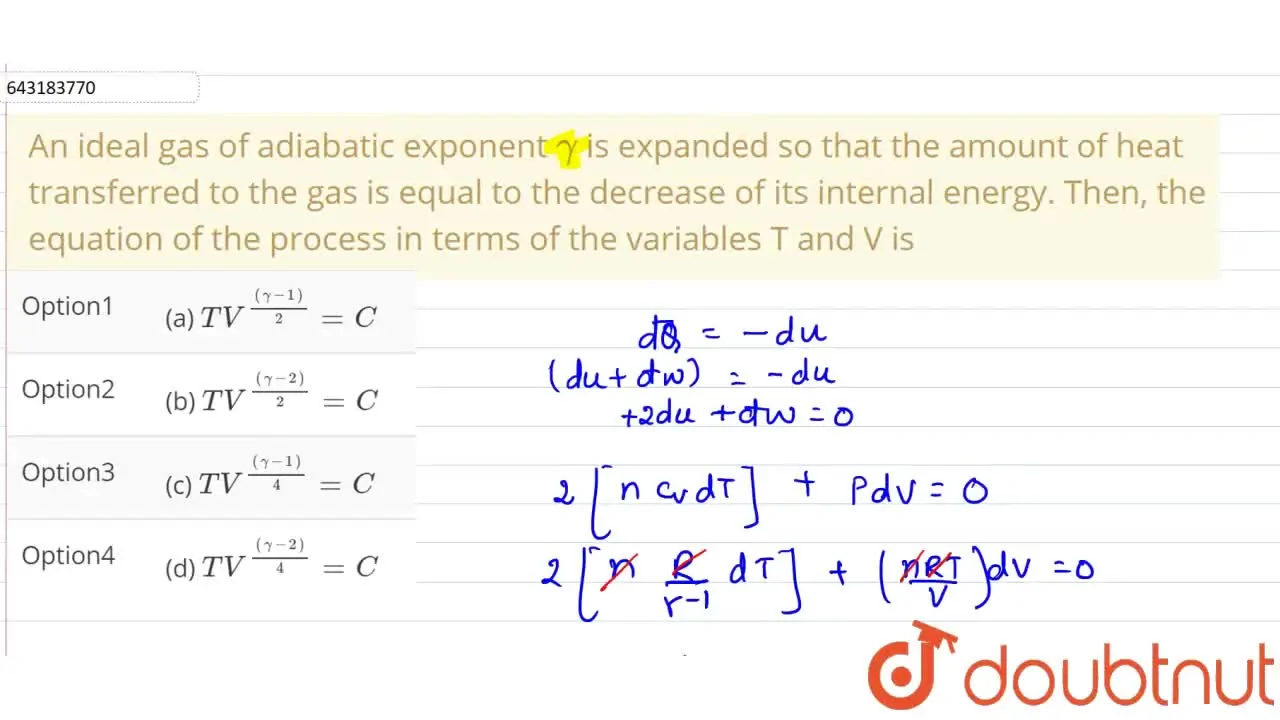
An ideal gas of adiabatic exponent ${\displaystyle \gamma }$ is expanded so that the amount of heat transferred to the gas is equal to the decrease of its internal energy. Then, the equation of the process in terms of the variables T and V is

Options

A. (a) `TV^(((gamma-1))/(2))=C`
B. (b) `TV^(((gamma-2))/(2))=C`
C. (c) `TV^(((gamma-1))/(4))=C`
D. (d) `TV^(((gamma-2))/(4))=C`

Answer

A

Explanation

To solve the problem step by step, we need to derive the equation of the process for an ideal gas under the given conditions. Let's go through the solution systematically.

Step 1: Understand the Given Conditions
We are given that the amount of heat transferred to the gas (dQ) is equal to the decrease in its internal energy (dU). According to the first law of thermodynamics, we have:
\( dQ = dU + dW \)
Since \( dQ = dU \), it implies:
\( dU + dW = 0 \)
This means:
\( dW = -dU \)

Step 2: Express Changes in Internal Energy and Work Done
The change in internal energy (dU) for an ideal gas can be expressed as:
\( dU = n C_v dT \)
where \( n \) is the number of moles, \( C_v \) is the molar specific heat at constant volume, and \( dT \) is the change in temperature.

The work done (dW) during an expansion can be expressed as:
\( dW = P dV \)
where \( P \) is the pressure and \( dV \) is the change in volume.

Step 3: Substitute dW and dU into the Equation
Substituting the expressions for dU and dW into the equation we derived:
\( P dV = -n C_v dT \)

Step 4: Use the Ideal Gas Law
From the ideal gas law, we know:
\( PV = nRT \)
Thus, we can express pressure \( P \) as:
\( P = \frac{nRT}{V} \)
Substituting this into our equation gives:
\( \frac{nRT}{V} dV = -n C_v dT \)

Step 5: Simplify the Equation
We can cancel \( n \) from both sides (assuming \( n \neq 0 \)):
\( \frac{RT}{V} dV = -C_v dT \)
Rearranging gives:
\( \frac{dT}{T} = -\frac{R}{C_v} \frac{dV}{V} \)

Step 6: Integrate Both Sides
Integrating both sides:
\( \int \frac{dT}{T} = -\frac{R}{C_v} \int \frac{dV}{V} \)
This results in:
\( \ln T = -\frac{R}{C_v} \ln V + \ln C \)
where \( C \) is the constant of integration.

Step 7: Exponentiate to Solve for T and V
Exponentiating both sides leads to:
\( T = C V^{-\frac{R}{C_v}} \)
Using the relation \( C_v = \frac{R}{\gamma - 1} \), we can rewrite:
\( T = C V^{-\frac{R}{\frac{R}{\gamma - 1}}} = C V^{-\frac{(\gamma - 1)}{1}} \)
Thus:
\( T V^{\gamma - 1} = C \)

Final Equation
The final equation of the process in terms of T and V is:
\( T V^{\gamma - 1} = C \)

---