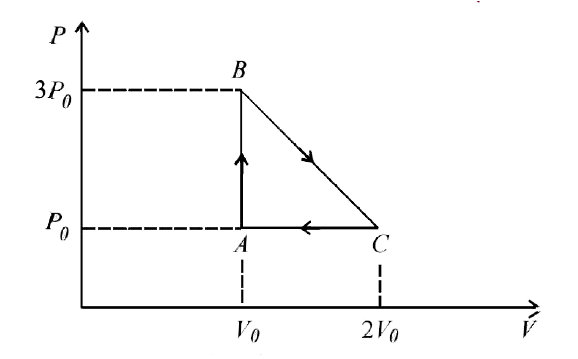
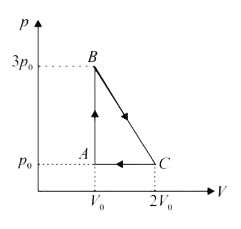
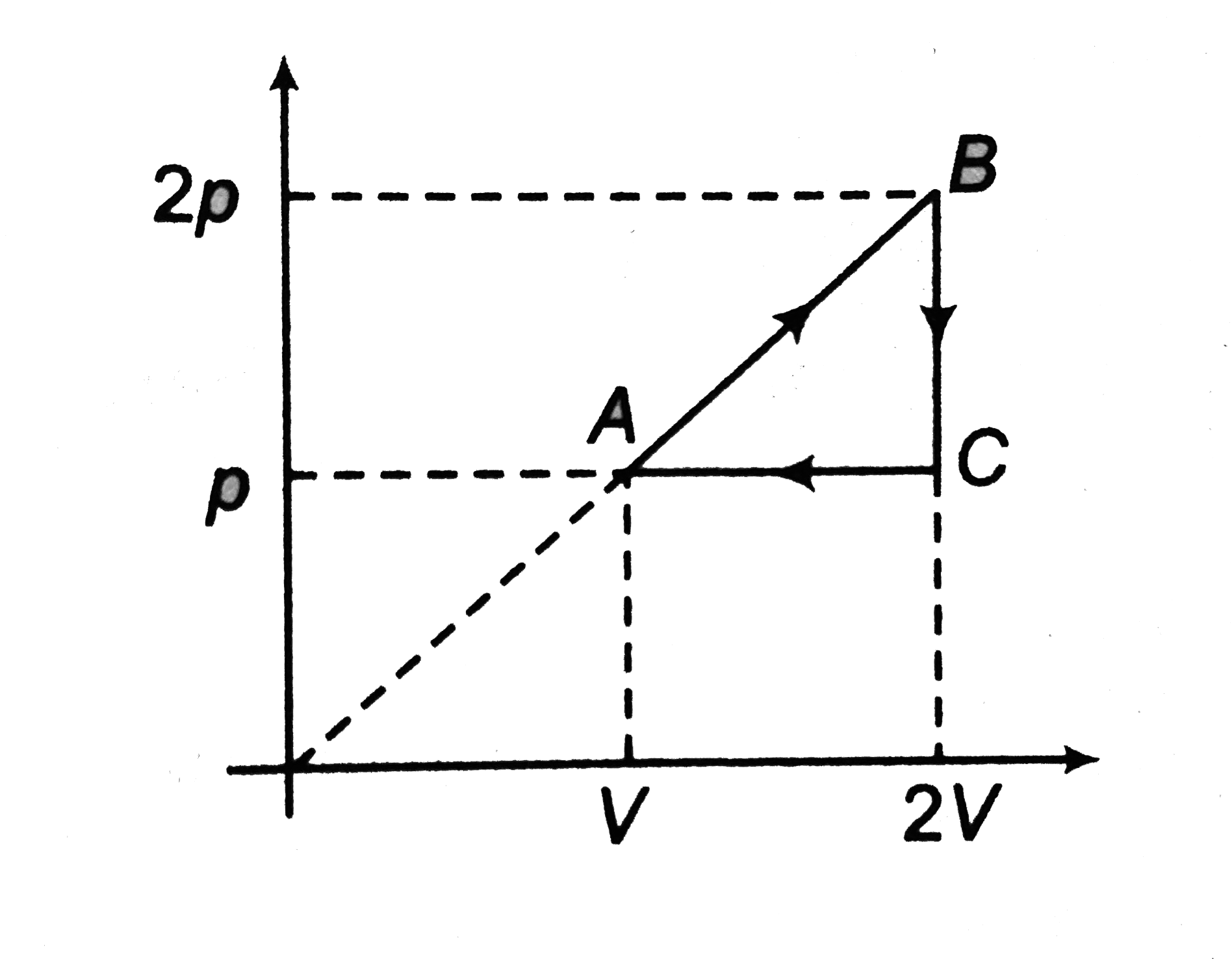
One mole of an ideal monatomic gas is taken round the cyclic process ABCA as shown in figure. Calculate
(a) the work done by the gas.
(b) the heat rejected by the gas in the path CA and the heat absorbed by the gas in the path AB,
(c) the net heat absorbed by the gas in the path BC,
(d) the maximum temperature attained by the gas during the cycle.
The correct Answer is:A, B, C, D
(a) W=+ (Area of the cycle)
=+12(2V0−V0)(3p0−p0)
=p0V0
(b) QCA=nCpΔT
=n(52R)(TA−TC)
=52(pAVA−pCVC)
=52(p0V0−2p0V0)=−52p0V0
QAB=nCVΔT
=n(32R)(TB−TA)
=32(pBVB−pAVA)
=3p0V0
(c) WBC=+ (Area under the graph)
=2p0V0
ΔBC=nCVΔT
=n(32R)(TC−TB)
=32(pCVC−pBVB)=−32p0V0
∴ QBC=WBC+ΔUBC=p0V02
(d) p-V equation along path BC is
p=−(2p0V0)V+5p0
or pV=−(2p0V0)V2+5p0V
or RT=−(2p0V0)V2+5p0V
∴ T=1R[5p0V−2p0V0V2]
For T to be maximum,
dTdV=0
∴ 5p0−4p0V0V=0
∴ V=54V0
So at this volume, temperature is maximum.
Substituting this value of V in Eq. (i), we get
Tmax=25p0V08R
Topper's Solved these Questions
- LAWS OF THERMODYNAMICSBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Single Correct
27 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 More Than One Correct
6 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 1 Objective Questions
1 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Medical entrances gallery
39 Videos
- MAGNETIC EFFECT OF CURRENT AND MAGNETISMBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Integer type Questions
10 Videos
Similar Questions
One mole of an ideal mono-atomic gas is taken round cyclic process ABCD as shown in figure below. Calculate work done by the gas.
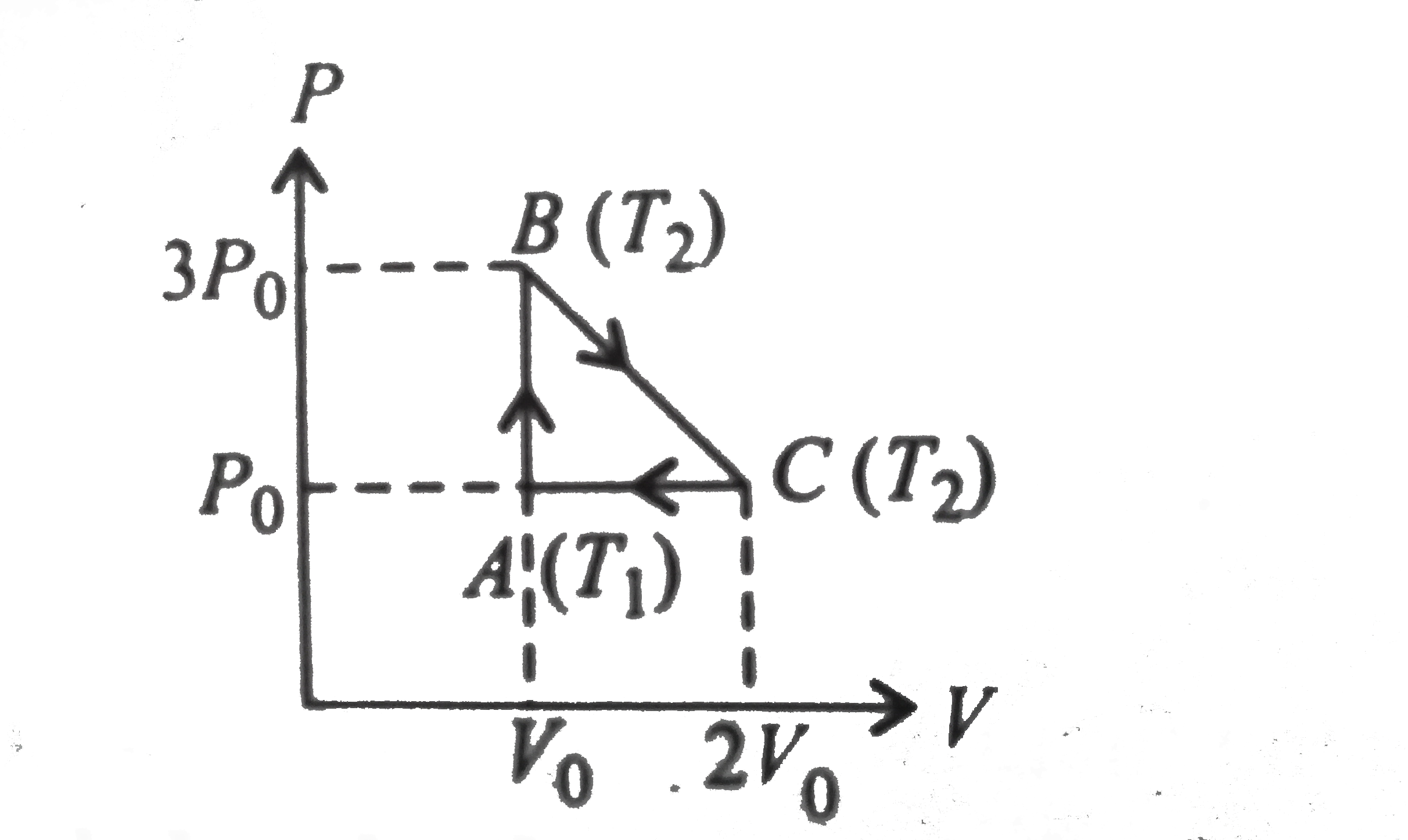
View SolutionOne mole of an ideal mono-atomic gas is taken round cyclic process ABC as shown in figure below. Calculate work done
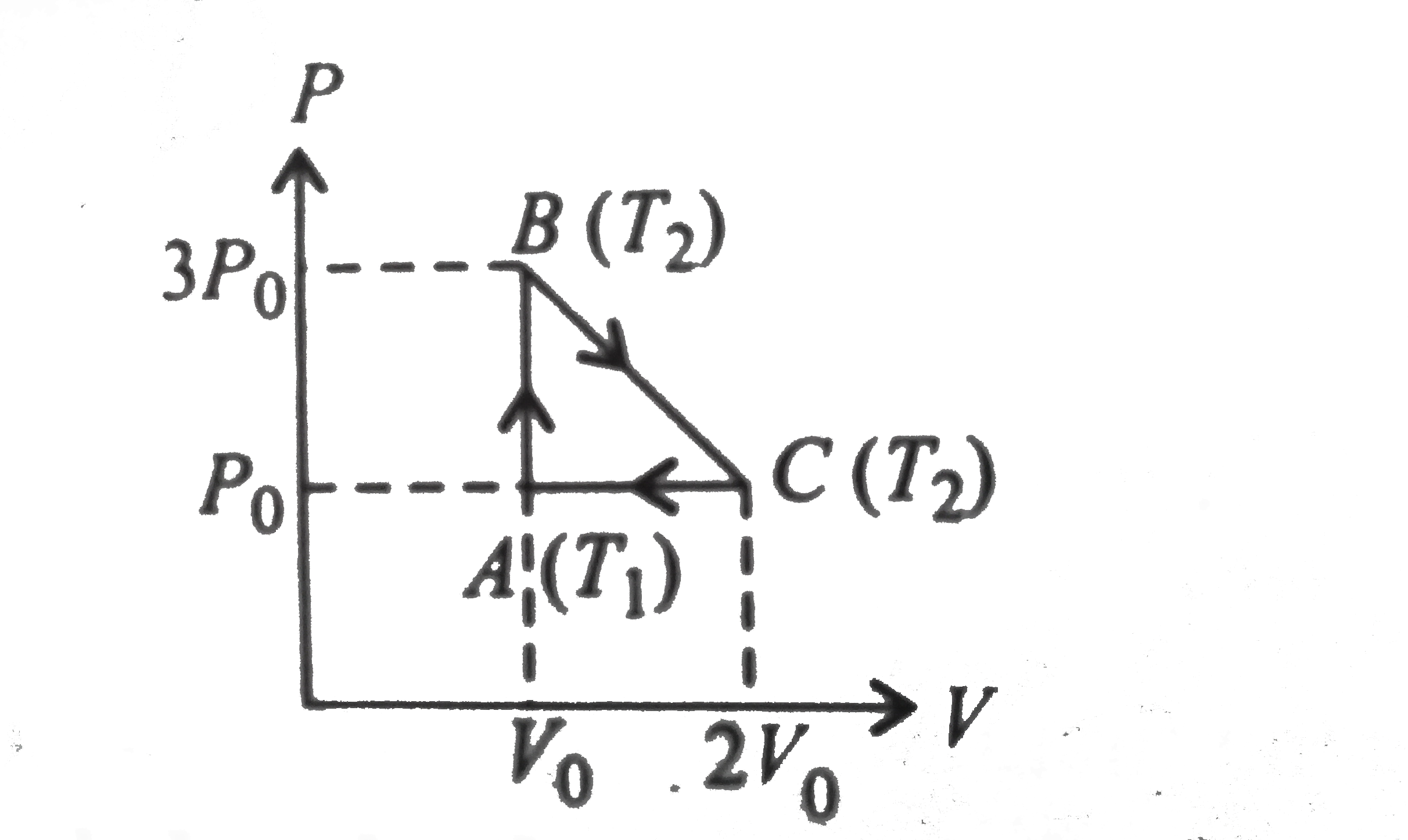
View SolutionOne mole of an ideal mono-atomic gas is taken round cyclic process ABC as shown in figure below. Calculate work done
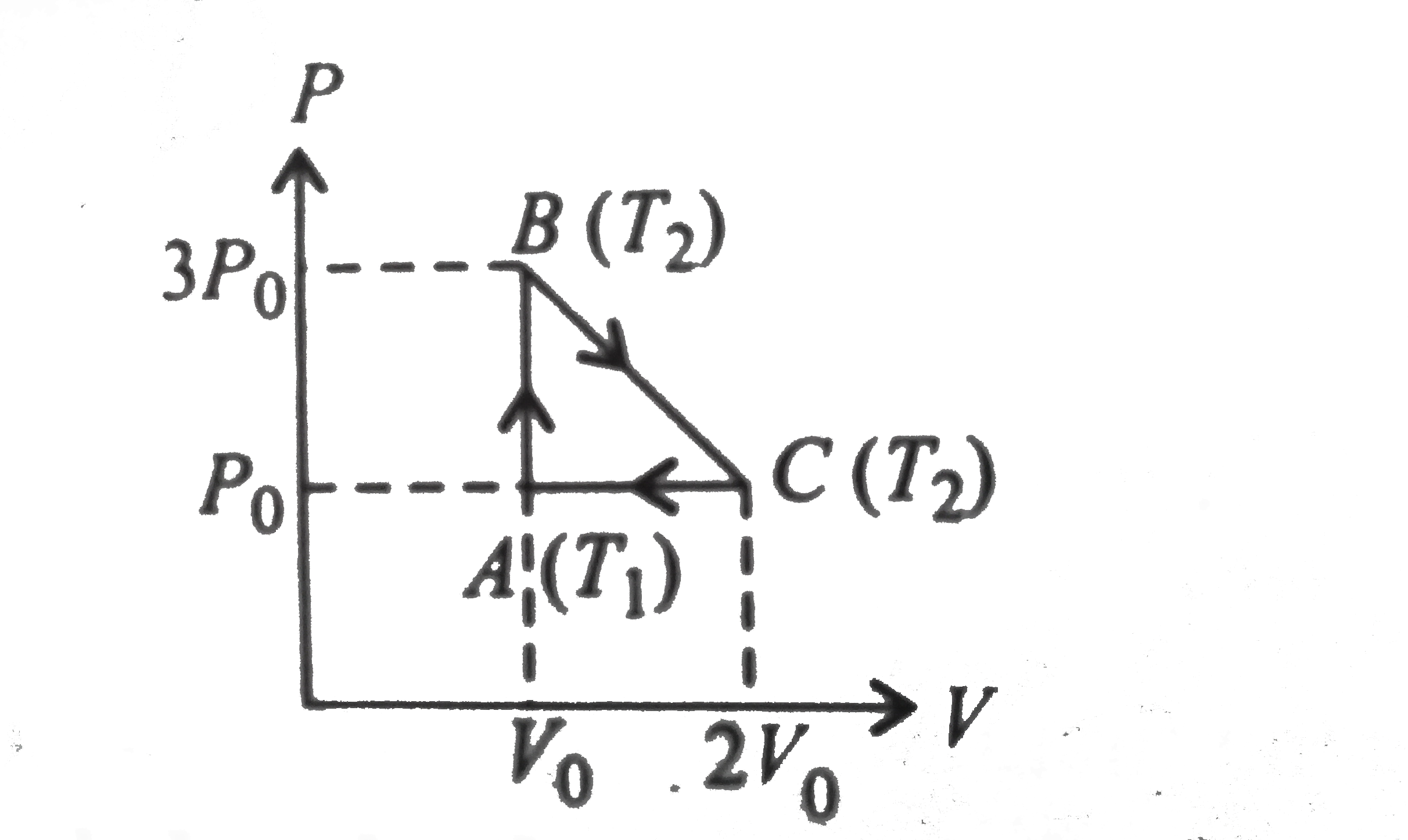
View SolutionOne mole of ideal monoatmic gas is carried throught the reversible cyclic process as shown in figure. Calculate net heat absorbed by the gas in the path BC
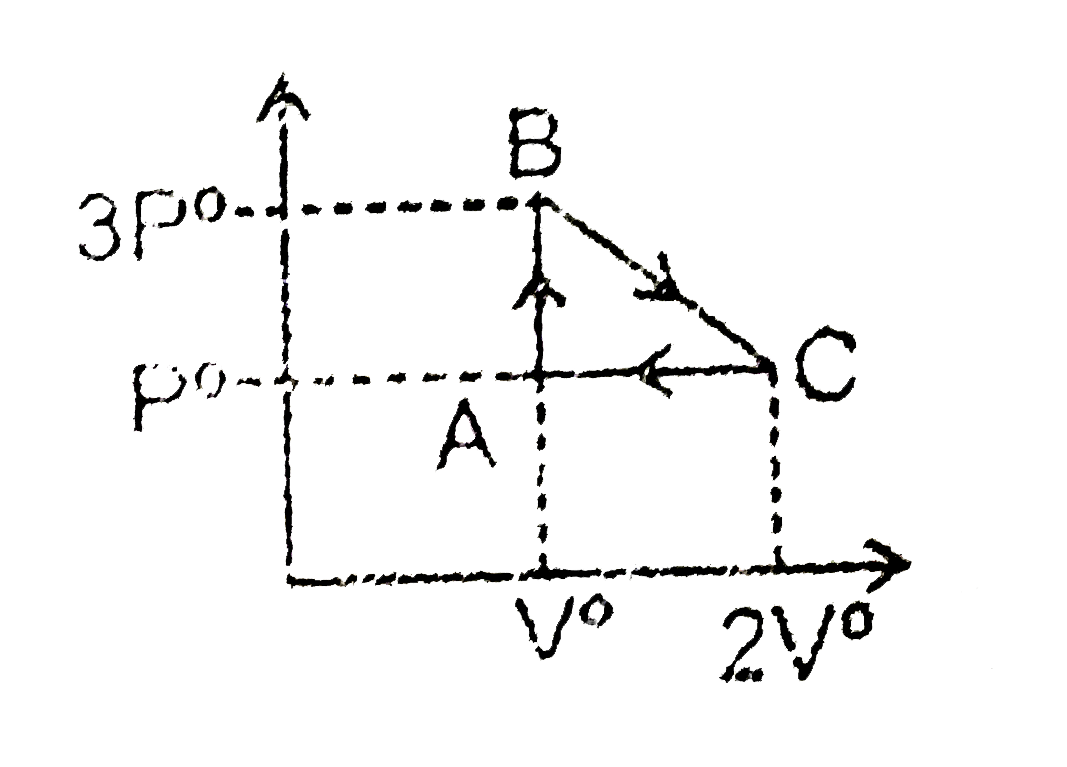
View SolutionOne mole of monoatomic gas is taken round the cyclic process. Heat absorbed by gas in process AB is
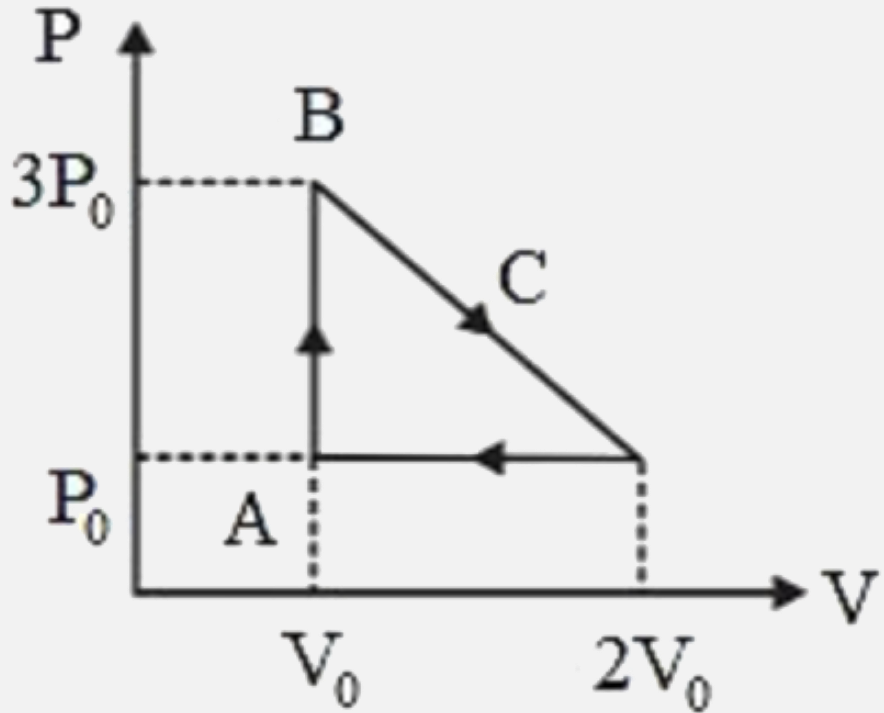
View SolutionOne mole of ideal monoatomic gas is taken round the cyclic process as shown in figure.
The heat rejected by the gas in the path CA is ………...and the heat absorbed by the gas in the path AB is ………...........
View SolutionOne mole of ideal monatomic gas is carried through the reversible cyclic process as shown in figure. Calculate the max temperature attained by the gas during the cycle.
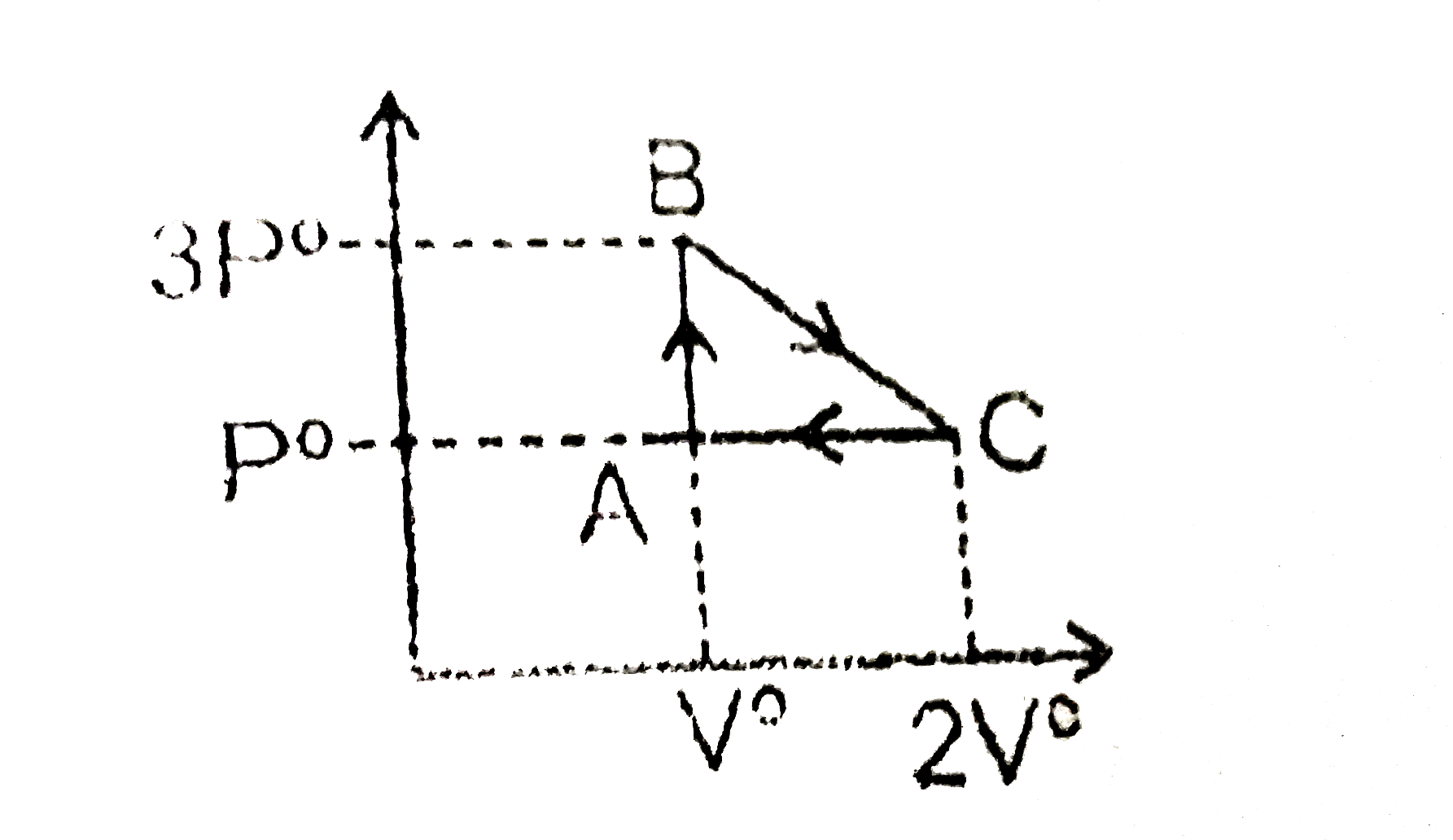
View SolutionOne mole of a monatomic ideal gas is taken along the cycle ABCA as shown in the diagram.
The net heat absorbed by the gas in the given cycle is
View SolutionOne mole of an ideal monoatomic gas is taken along the path ABCA as shown in the PV diagram. The maximum temperature attained by the gas along the path BC is given by :

View SolutionAn ideal monoatomic gas undergoes a cyclic process ABCA as shown in the figure. The ratio of heat absorbed during AB to the work done on the gas during BC id
View Solution
