At what temperature is the "effective" speed of gaseous hydrogen molecules (molecular weight = 2) equal to that of oxygen molecules (molecular weight = 32) at 47∘C?
The correct Answer is:B, C
To solve the problem, we need to find the temperature at which the root mean square speed (VRMS) of hydrogen molecules is equal to that of oxygen molecules at 47°C.
1. Understand the formula for VRMS:
The root mean square speed (VRMS) of a gas is given by the formula:
VRMS=√3RTM
where R is the universal gas constant, T is the absolute temperature in Kelvin, and M is the molar mass of the gas in kg/mol.
2. Set up the equation:
According to the problem, we need to equate the VRMS of hydrogen (H₂) to the VRMS of oxygen (O₂) at 47°C.
- For hydrogen (molecular weight = 2 g/mol = 0.002 kg/mol):
VRMS,H2=√3RTH0.002
- For oxygen (molecular weight = 32 g/mol = 0.032 kg/mol) at 47°C (which is 320 K):
VRMS,O2=√3R⋅3200.032
3. Set the two equations equal:
√3RTH0.002=√3R⋅3200.032
4. Simplify the equation:
Since √3R is common in both sides, we can cancel it out:
√TH0.002=√3200.032
5. Square both sides:
TH0.002=3200.032
6. Cross-multiply to solve for TH:
TH=0.002⋅3200.032
7. Calculate the right-hand side:
TH=0.002⋅10=0.02 K
8. Convert to Celsius:
Since the temperature in Kelvin is 0.02 K, we convert it to Celsius:
TH=0.02−273.15=−273.13°C
Final Answer:
The temperature at which the effective speed of gaseous hydrogen molecules is equal to that of oxygen molecules at 47°C is approximately -273.13 °C.
The root mean square speed (VRMS) of a gas is given by the formula:
VRMS=√3RTM
where R is the universal gas constant, T is the absolute temperature in Kelvin, and M is the molar mass of the gas in kg/mol.
According to the problem, we need to equate the VRMS of hydrogen (H₂) to the VRMS of oxygen (O₂) at 47°C.
- For hydrogen (molecular weight = 2 g/mol = 0.002 kg/mol):
VRMS,H2=√3RTH0.002
- For oxygen (molecular weight = 32 g/mol = 0.032 kg/mol) at 47°C (which is 320 K):
VRMS,O2=√3R⋅3200.032
√3RTH0.002=√3R⋅3200.032
Since √3R is common in both sides, we can cancel it out:
√TH0.002=√3200.032
TH0.002=3200.032
TH=0.002⋅3200.032
TH=0.002⋅10=0.02 K
Since the temperature in Kelvin is 0.02 K, we convert it to Celsius:
TH=0.02−273.15=−273.13°C
The temperature at which the effective speed of gaseous hydrogen molecules is equal to that of oxygen molecules at 47°C is approximately -273.13 °C.
Topper's Solved these Questions
- THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASESBOOK - DC PANDEY ENGLISHCHAPTER - THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASESEXERCISE - Level 2 Single Correct
12 Videos
- THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASESBOOK - DC PANDEY ENGLISHCHAPTER - THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASESEXERCISE - Level 2 More Than One Correct
7 Videos
- THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASESBOOK - DC PANDEY ENGLISHCHAPTER - THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASESEXERCISE - Level 1 Objective
10 Videos
- THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESBOOK - DC PANDEY ENGLISHCHAPTER - THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESEXERCISE - Medical entrance gallary
30 Videos
- UNIT AND DIMENSIONSBOOK - DC PANDEY ENGLISHCHAPTER - UNIT AND DIMENSIONSEXERCISE - Assertion And Reason
2 Videos
Similar Questions
Knowledge Check
At what temperature is the rms velocity of a hydrogen molecule equal to that of an oxygen molecule at 47∘C? a) 10 K b) 20 K c) 30 K d) 40 K
A10 KB20 KC30 KD40 K
At what temperature is the rms velocity of a hydrogen molecule equal to that of an oxygen molecule at 47∘C?
View SolutionAt what temperature the mean speed of the molecules of hydrogen gas equals the escape speed from the earth?
View SolutionWhat do you understand by :
Molecular weight
View Solution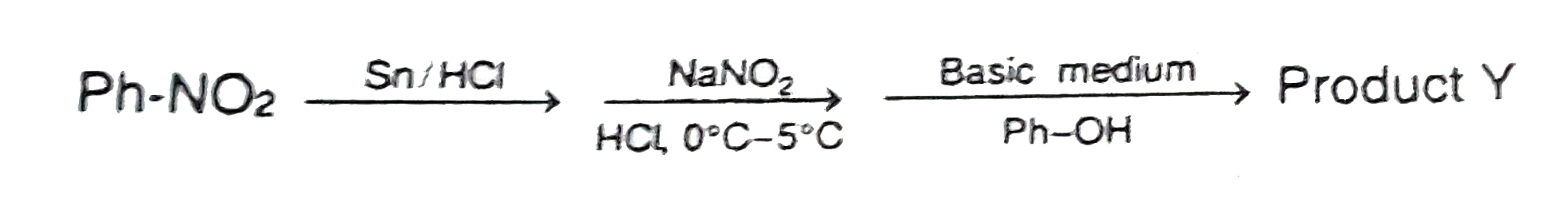
Find the molecular weight of Y report your answer as Molecular weight2
View SolutionAt what temperature is the rms speed of H2 molecules the same as that of oxygen molecules at 1327∘C ?
View SolutionAt what temperature is the rms speed of H2 molecules the same as that of oxygen molecules at 1327∘C ?
View SolutionAt what temperature do the average speed of CH4(g) molecules equal the average speed of O2 molecules at 300 K ?
View Solution
