In the wave equation,
y=Asin(2π)a(x−bt)
speed of wave is a
speed of wave is b
wavelength of wave is a/b
wavelength of wave is a
The correct Answer is:B, D
To solve the given wave equation y=Asin(2πA(x−bt)), we need to find the speed of the wave and its wavelength. Let's break this down step by step.
Step 1: Identify the wave equation format
The general form of a wave equation is:
y=Asin(kx−ωt)
where:
- A is the amplitude,
- k is the wave number,
- ω is the angular frequency.
Step 2: Rewrite the given equation
The given equation is:
y=Asin(2πA(x−bt))
We can rewrite it in the standard form:
y=Asin(2πAx−2πbAt)
Step 3: Identify k and ω
From the rewritten equation:
- The coefficient of x is k=2πA
- The coefficient of t is ω=2πbA
Step 4: Calculate the speed of the wave
The speed of the wave v can be calculated using the formula:
v=ωk
Substituting the values of ω and k:
v=2πbA2πA=b
Step 5: Calculate the wavelength
The wavelength λ is related to the wave number k by the formula:
k=2πλ
From our earlier identification, we have:
k=2πA
Setting these equal gives:
2πλ=2πA
Cancelling 2π from both sides, we find:
λ=A
Final Results
- The speed of the wave v is b.
- The wavelength λ is A.
Summary
- Speed of the wave: v=b
- Wavelength: λ=A
The general form of a wave equation is:
y=Asin(kx−ωt)
where:
- A is the amplitude,
- k is the wave number,
- ω is the angular frequency.
The given equation is:
y=Asin(2πA(x−bt))
We can rewrite it in the standard form:
y=Asin(2πAx−2πbAt)
From the rewritten equation:
- The coefficient of x is k=2πA
- The coefficient of t is ω=2πbA
The speed of the wave v can be calculated using the formula:
v=ωk
Substituting the values of ω and k:
v=2πbA2πA=b
The wavelength λ is related to the wave number k by the formula:
k=2πλ
From our earlier identification, we have:
k=2πA
Setting these equal gives:
2πλ=2πA
Cancelling 2π from both sides, we find:
λ=A
- The speed of the wave v is b.
- The wavelength λ is A.
- Speed of the wave: v=b
- Wavelength: λ=A
Topper's Solved these Questions
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Level 2 Subjective
7 Videos
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Subjective Questions
2 Videos
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Level 2 Single Correct
5 Videos
- VECTORSBOOK - DC PANDEY ENGLISHCHAPTER - VECTORSEXERCISE - Medical enrances gallery
9 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos
Similar Questions
Knowledge Check
A transverse wave is represented by the equation
y=y0sin(2π)λ(vt−x)
For what value of λ is the particle velocity equal to two time the wave velocity ?Aλ=2πy0Bλ=2πy0/3Cλ=πy0/2Dλ=πy0
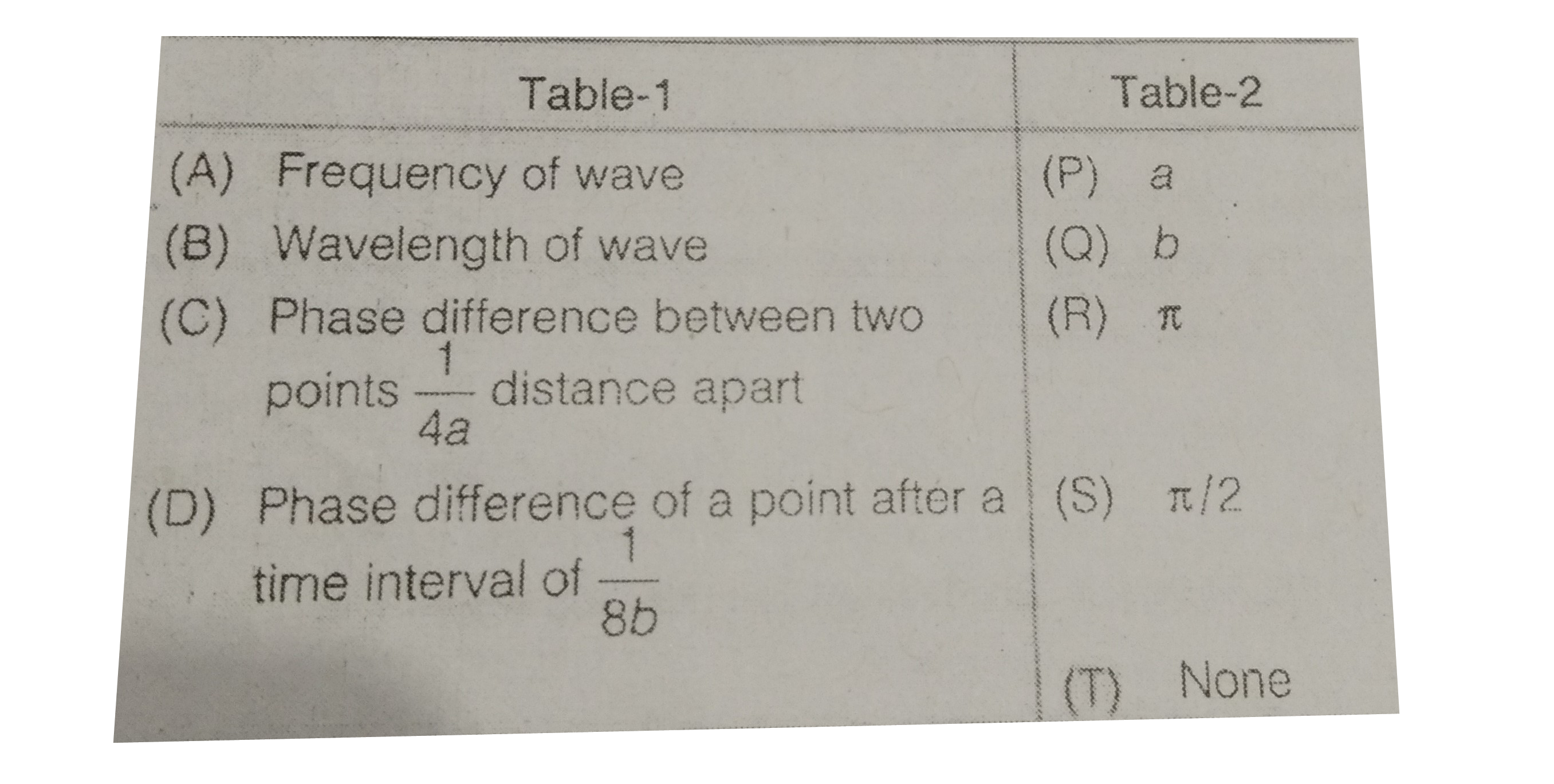
In the equation ,y =A sin 2π(ax+bt+π/4)match the following.
View SolutionThe transverse wave represented by the equation y=4sin(π6)sin(3x−15t) has
View SolutionTwo waves are passing through a region in the same direction at the same time . If the equation of these waves are
y1=asin(2π)λ(vt−x)
and y2=bsin(2π)λ[(vt−x)+x0]
then the amplitude of the resulting wave for x0=(λ/2) is
View SolutionThe equation of a wave is
y=4sin[π2(2t+18x)]
where y and x are in centimeres and t is in seconds.
View SolutionIn the equation y=Asin(ωt+π4) match the following for x=A2.

View SolutionA wave is represented by the equation :y=Asin(10πx+15πt+π/3) where, x is in metre and t is in second. The expression represents.
View SolutionThe equetion of a wave travelling on a string is
y=4sin(π)2(8t−x8)
if x and y are in centimetres, then velocity of waves is
View Solution
