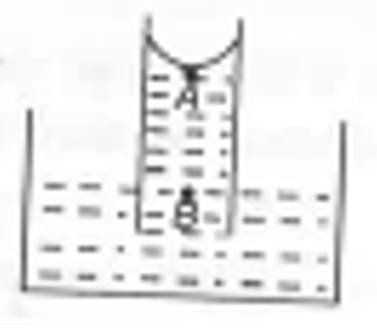
A capillary tube is dipped in a liquid. Let pressure at point A,B and C be pApB and pC respectively, then

1) p
A
=
p
B
=
p
C
2)p
A
=
p
B
<
p
C
3)p
A
=
p
C
<
p
B
4)p
A
=
p
C
>
p
B
pA=pB=pC
pA=pB<pC
pA=pC<pB
pA=pC>pB
The correct Answer is:D
pA=pC=(pB+ρgh).
Topper's Solved these Questions
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Level 1 Assertion And Reason
10 Videos
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Assertion and Reason
1 Videos
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Exercise16.7
4 Videos
- EXPERIMENTSBOOK - DC PANDEY ENGLISHCHAPTER - EXPERIMENTSEXERCISE - Subjective
15 Videos
- GENERAL PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
Knowledge Check
An experiment yields 3 mutually exclusive and exclusive events A, B and C . If P(A) = 2P(B) = 3 P( C ) , then P(A) is equal to
A111B211C311D611
In a triangle ABC , let P and Q be points on AB and AC respectively such that PQ∣∣BC . Prove that the median AD bisects PQ.
View SolutionTriangle ABC has right angle at B and contains a point P for which PA=10,PB=6 and ∠APB=∠BPC=∠CPA, then (PC11) is
View SolutionIn ABC , P and Q are points on sides AB and AC respectively such that PQ∣∣BC . If AP=3cm , PB=5cm and AC=8cm , find AQ .
View SolutionIn a right triangle ABC right-angled at B, if PandQ are points on the sides ABandAC respectively, then (a)AQ2+CP2=2(AC2+PQ2) (b)2(AQ2+CP2)=AC2+PQ2 (c)AQ2+CP2=AC2+PQ2 (d)AQ+CP=12(AC+PQ).
View SolutionP and Q are points on the sides CA and CB respectively of ABC, right-angled at C. Prove that AQ2+BP2=AB2+PQ2.
View SolutionIf a,b,andc are in A.P. p,q,andr are in H.P., and ap,bq,andcr are in G.P., then pr+rp is equal to a/c+c/a
View SolutionThe plots of 1XAvs.1YA (where XA and YA are the mole fraction of liquid A in liquid and vapour phase respectively) is linear with slope and intercept respectively are given as:
(a)P∘AP∘B,P∘B−P∘AP∘B
(b)P∘BP∘A,P∘A−P∘BP∘A
(c)P∘BP∘A,P∘BP∘B−P∘A
(d)P∘A−P∘B,P∘AP∘B
View Solution