A closed tube is filled with

AB=2m
BC=4cm
water (ρ=103kg/m3). It rotating about an axis shown in figure with an angular velocity ω=2rad/s. Find, pA−pC.
The correct Answer is:A, B, C, D
Pressure decreases in moving towards the axis of rotation and increases in moving away from the axis (Δp=±ρω2x22)
∴pA>pB and pB>pC
pA−pC=(pA−pB)+(pB−pC)
=(+ρω2x212)+(−ρω2x222)
Here, x1=AB=2m
and x2=BC=4m
Substituting teh values we have,
pA−pC=(103)(2)2(2)22−(103)(2)2(4)22
=−2.4×103N/m2.
Topper's Solved these Questions
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Solved Examples
21 Videos
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Miscellaneous Examples
10 Videos
- EXPERIMENTSBOOK - DC PANDEY ENGLISHCHAPTER - EXPERIMENTSEXERCISE - Subjective
15 Videos
- GENERAL PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
A closed container shown in figure is filled with water (ρ=103kg/M3)
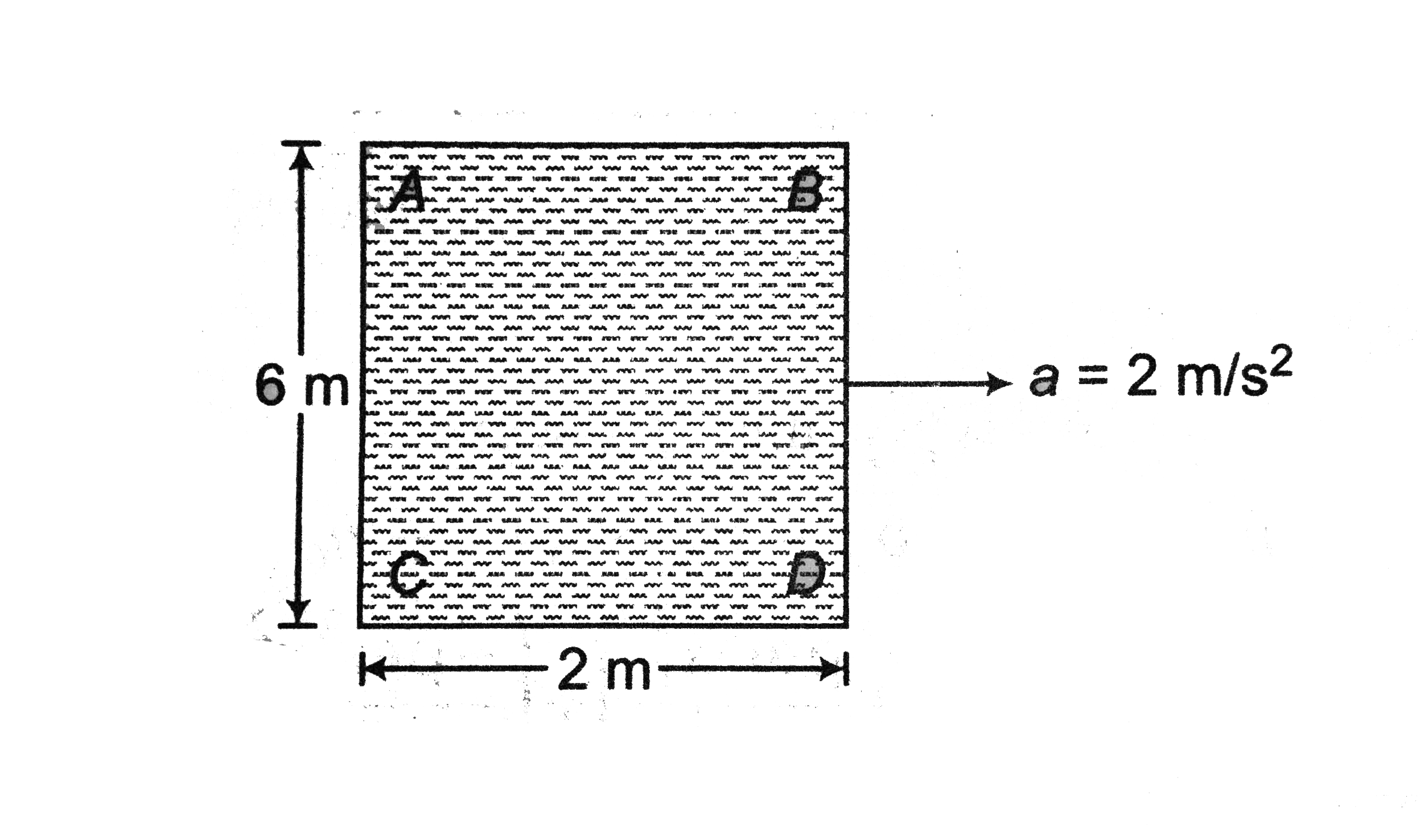
This is accelerated in horizonal direction with an acceleration, a=2m/s2.Find (a)pC−pD and (b) pA−pD.
View Solution
A rod of mass 2 kg ad length 2 m is rotating about its one end O with an angular velocity ω=4rad/s. Find angular momentum of the rod about the axis rotation.
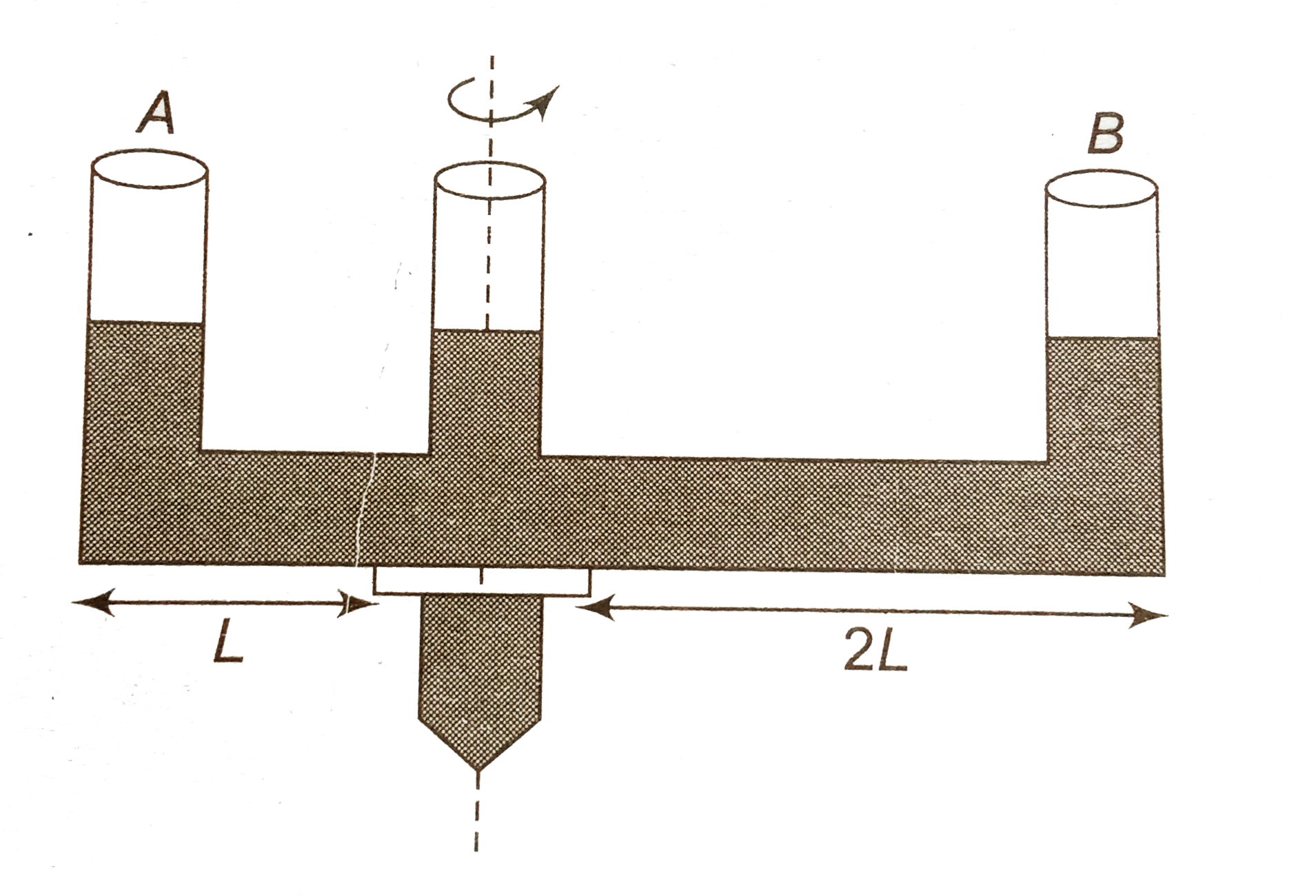
View SolutionA given shaped glass tube having uniform cross-section is filled with water and is mounted on a rotatable shaft as shown in figure. If the tube is rotated with a constant angular velocity ω then :
View SolutionA soild cylinder of mass 2 kg and radius 0.2 m is rotating about its owm axis without friction with an angular velocity of 3 rad s−1 . Angular momentum of the cylinder is
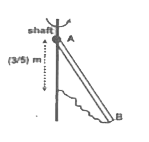
View SolutionA rod AB of length l = 1m and mass m = 2kg is pinned to a vertical shaft and a massless string is tied with the shaft at the lower end of the rod ( length of the string is 45m ) as shown in figure.If the shaft starts rotating with a constant angular velocity ω=5 rad / sec. Then find the tension in the string.
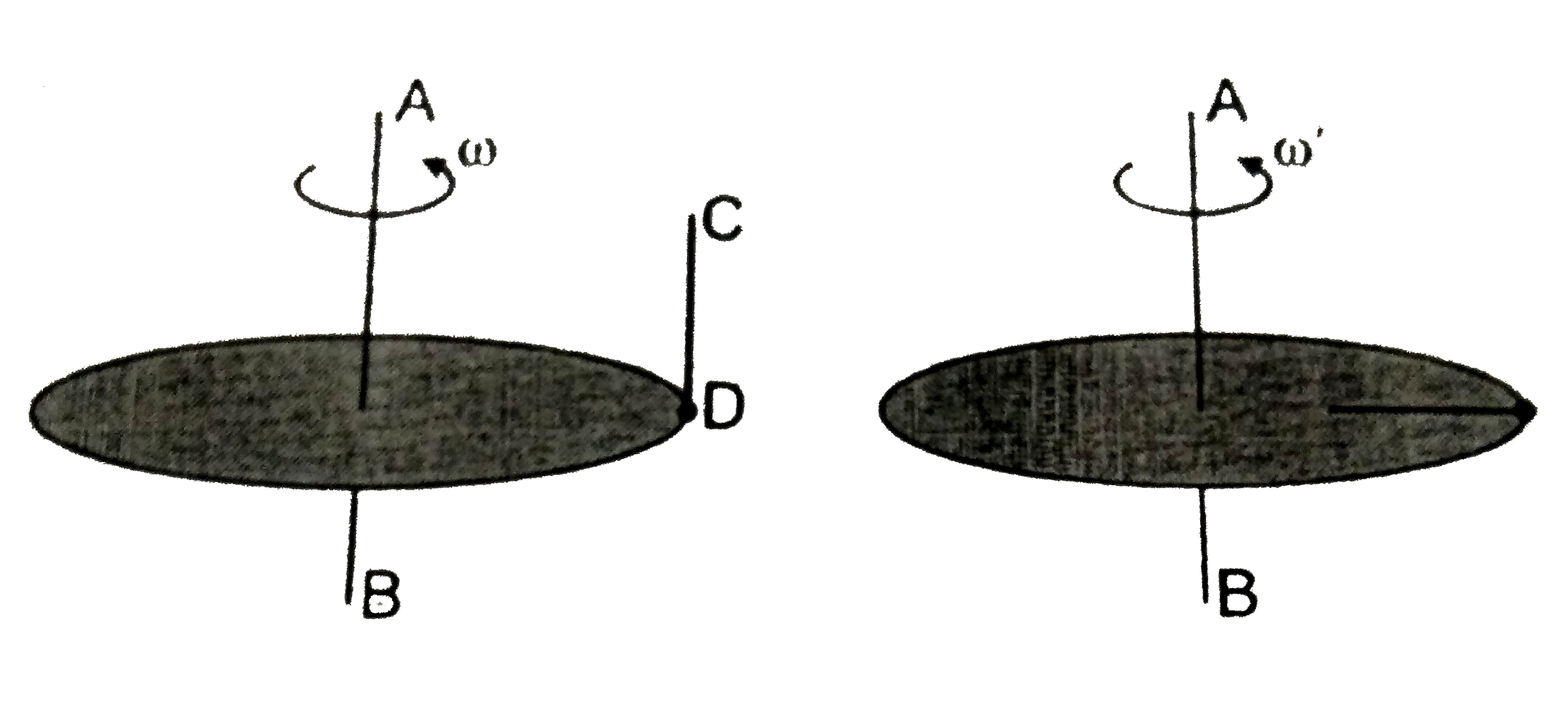
View SolutionIn the figure (i) a disc of mass M(kg) and radius R(m) is rotating smoothly about a fixed vertical axis AB with angular speed 26rad/s A rod rod CD of length R2(m) and mass M(kg) is hinged at one end at point 'D' on the disc. The rod remains in vertical position and rotates along with the disc about axis AB. At some moment the rod CD gets a very smal impulse at point 'C' due to air due to which the rod falls on the disc along one radius and sticks to the disc as shown in figure (ii). Now find the angular velocity of the disc in rad/s.
View SolutionLength of horizontal arm of a uniform cross section U-tube is l=21cm and ends of both of the vertical arms are open to surrounding of pressure 10500N/m2. A liquid of density ρ=103kg/m3 is poured into the tube such that liquid just fills the horizontal part of the tube. Now one of the open ends is sealed and the tube is then rotated about a vertical axis passing through the other vertical arm With angular velocity ω0=10rad/s. If length of each vertical arm is a=6cm, calculate the length of air column in the sealed arm. (in cm)

View Solution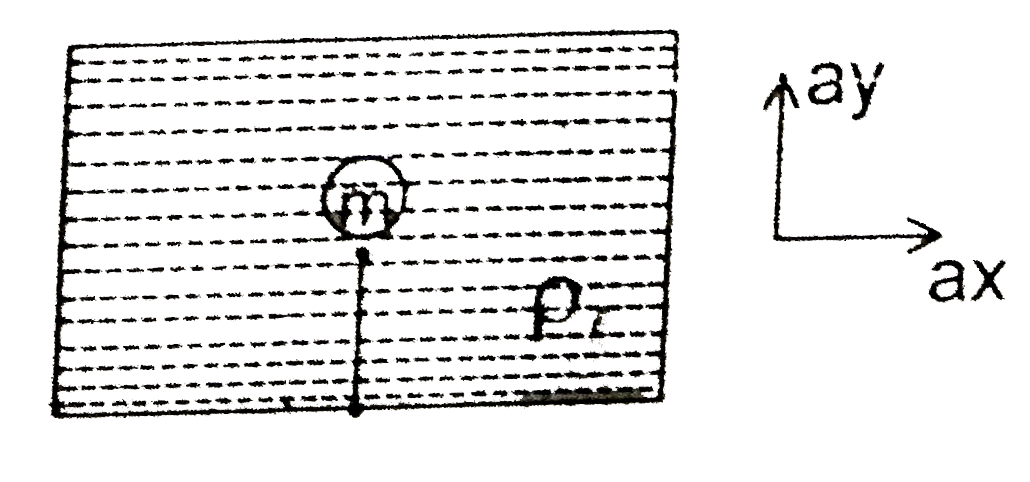
A mass m of volume V=10cm2 is tied with a string to the base of a container filled with a liquid of density (Pl=103kg/m3) as shown in the figure. The container is then accelerated with acceleration (ax=9m/s2) and (ay=2m/s2) a shown in the figure. [g=10(ms2) acceleration due to gravity] the net buoyancy force acting on the mass is
View SolutionA ring of mass m and radius R is given a charge q. It is then rotated about its axis with angular velocity ω.Find
(iii)Magnetic moment of ring.
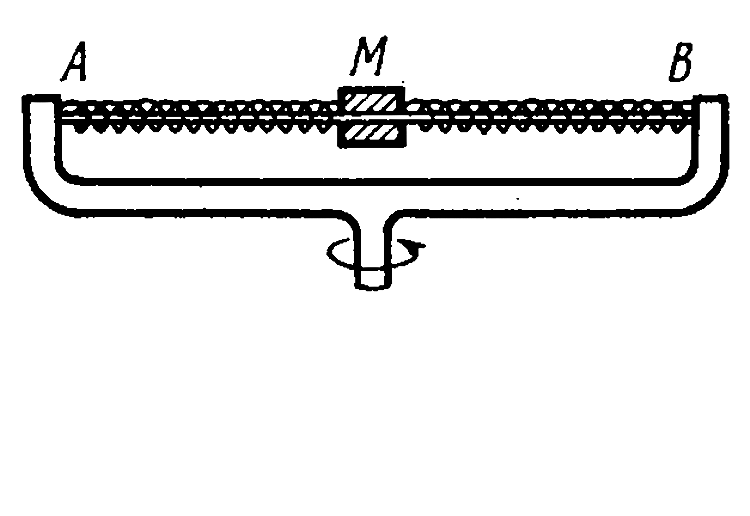
View SolutionIn the arrangement shown in figure the sleeve M of mass m=0.20kg is fixed between two identical springs whose combined stiffness is equal to x=20N/m. The sleeve can slide without friction over a horizontal bar AB. The arrrangement rotates with a constant angular velocity ω=4.4rad/s about a vertical axis passing through the middle of the bar . Find the period of small oscillations of the sleeve. At what values of ω will there be no oscillations of the sleeve ?
View Solution
