A 2.0kg particle undergoes SHM according to x=1.5cos(πt4+π6) (in SI .units)
(a) What is the total mechanical energy of the particle ?
(b) What is the shortest time required for the particle to move from x=0.5m to x=−0.75m ?
The correct Answer is:A, B, C
(a) E=12mv2max=12(m)ω2A2
=12×2.0×(π4)2×(1.5)2
=1.39J
(b) 0.5=1.5sin(πt14+π6)
From here find t.
Then, −0.75=1.5sin(πt24+π6)
From here find t2.
Now, t1~t2 is the required time.
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise 14.2
5 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise 14.3
7 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise 14.1
5 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)
38 Videos
Similar Questions
Knowledge Check
A particle executing SHM according to the equation x=5cos(2πt+π4) in SI units. The displacement and acceleration of the particle at t=1.5 s is
A−3.0m,100ms−2B+2.54m,200ms−2C−3.54m,140ms−2D+3.55m,120ms−2
The potential energy of a particle of mass 2 kg in SHM is (9x2)J. Here x is the displacement from mean position . If total mechanical energy of the particle is 36 J. The maximum speed of the particle is
View SolutionA particle executes SHM according to equation x=10(cm)cos[2πt+π2], where t is in seconds. The magnitude of the velocity of the particle at t=16s will be :-
View SolutionForce acting on a particle is F=−8x in S.H.M. The amplitude of oscillation is 2 (in m) and mass of the particle is 0.5 kg. The total mechanical energy of the particle is 20 J. Find the potential energy of the particle in mean position (in J).
View SolutionA particle executes SHM according to the equation, y=4sin(πt+π3),where y is in m and t is in's '.The phase of particle at time t=0 is
View SolutionA particle moves according to the equation x=acosπt. The distance covered by it in 2.5 s is
View Solutionx−t graph of a particle in SHM is
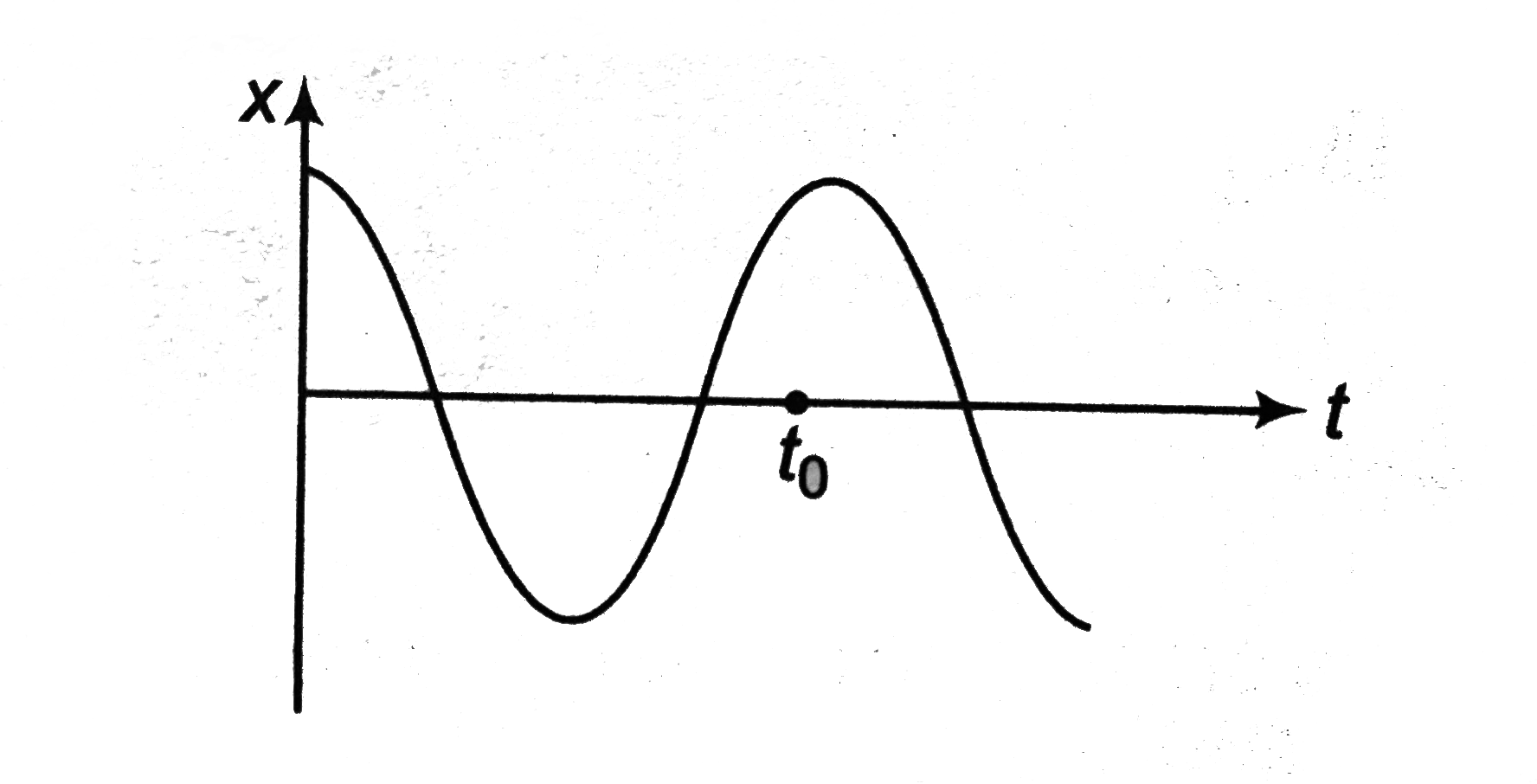
At time t=t0, what are the signs of v and a of the particle?
View SolutionA particle moves according to the law x=rcos(pi)t/(2) The distance covered by it in the time interval between t=0 to t=3s is
View Solution
