Five point masses m each are kept at five vertices of a regular pentagon. Distance of center of pentagon from any one of the vertice is 'a'. Find gravitational potential and filed strength at center.
The correct Answer is:A
To solve the problem of finding the gravitational potential and field strength at the center of a regular pentagon with five point masses m at its vertices, we can follow these steps:
Step 1: Understand the Geometry
We have a regular pentagon with five vertices, each occupied by a mass m. The distance from the center of the pentagon to any vertex is given as a.
Step 2: Calculate the Gravitational Potential
The gravitational potential V due to a single mass m at a distance r is given by the formula:
V=−Gmr
where G is the gravitational constant.
Since there are five identical masses m located at the vertices of the pentagon, the total gravitational potential Vtotal at the center O of the pentagon is the sum of the potentials due to each mass. Since potential is a scalar quantity, we can simply add them:
Vtotal=V1+V2+V3+V4+V5=−Gma−Gma−Gma−Gma−Gma
This simplifies to:
Vtotal=−5Gma
Step 3: Calculate the Gravitational Field Strength
The gravitational field strength E at a point is defined as the force per unit mass experienced by a small test mass placed at that point. The gravitational field E due to a single mass m at a distance r is given by:
E=−Gmr2
However, in this case, we need to consider the contributions from all five masses.
When we place a test mass at the center, the forces due to each of the five masses will act towards their respective vertices. Due to the symmetry of the pentagon, the forces exerted by the masses will cancel each other out. Therefore, the net gravitational field Etotal at the center O will be:
Etotal=0
Final Results
1. The gravitational potential at the center of the pentagon is:
Vtotal=−5Gma
2. The gravitational field strength at the center of the pentagon is:
Etotal=0
We have a regular pentagon with five vertices, each occupied by a mass m. The distance from the center of the pentagon to any vertex is given as a.
The gravitational potential V due to a single mass m at a distance r is given by the formula:
V=−Gmr
where G is the gravitational constant.
Vtotal=V1+V2+V3+V4+V5=−Gma−Gma−Gma−Gma−Gma
This simplifies to:
Vtotal=−5Gma
The gravitational field strength E at a point is defined as the force per unit mass experienced by a small test mass placed at that point. The gravitational field E due to a single mass m at a distance r is given by:
E=−Gmr2
However, in this case, we need to consider the contributions from all five masses.
Etotal=0
1. The gravitational potential at the center of the pentagon is:
Vtotal=−5Gma
2. The gravitational field strength at the center of the pentagon is:
Etotal=0
Topper's Solved these Questions
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - Exercise 13.4
4 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - Exercise 13.5
6 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - Exercise 13.2
9 Videos
- GENERAL PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE
10 Videos
Similar Questions
Knowledge Check
Six point masses of mass m each are at the vertices of a regular hexagon of side l. Calculate the force on any of the masses.
AGm2l2[54+1√3]BGm2l2[34+1√3]CGm2l2[54−1√3]DGm2l2[34−1√3]
Four point masses each of mass m are placed on vertices of a regular tetrahedron. Distance between any two masses is r.
View SolutionFour point masses easch of mass 'm' are placed at four vertieces A, B, C, nd D of a regular hexagon of side 'a' as shown in figure , Find gravitational potential and field strength at the centre O of the hexagon.
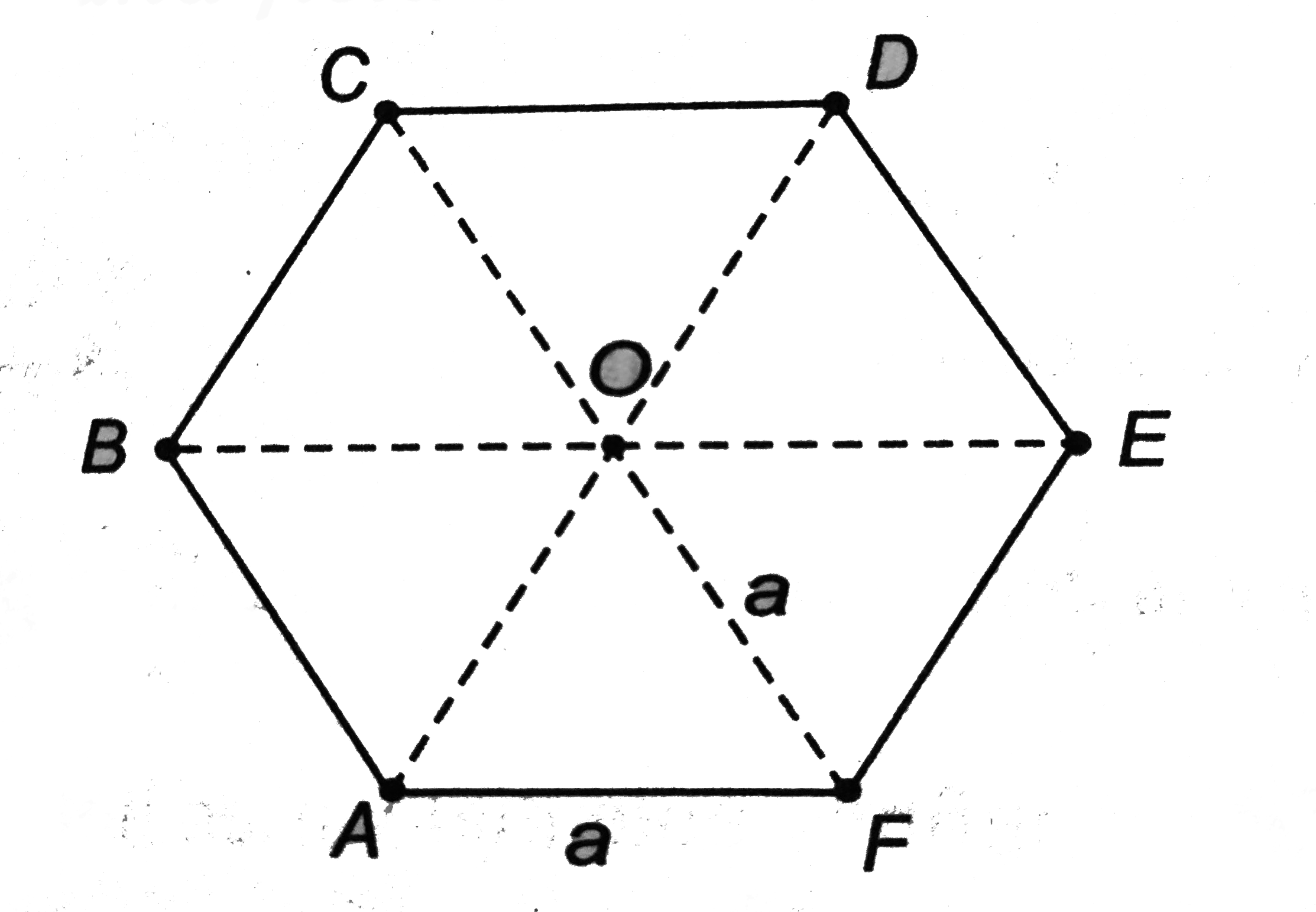 .
.
View SolutionIn the above problem, if any one point mass is removed then what is gravitational potential and magnitude of filed strength at centre?
View SolutionTwo points masses m each are kept at the two verticle of an equilateral triangle of side 'a' as shows in figure.
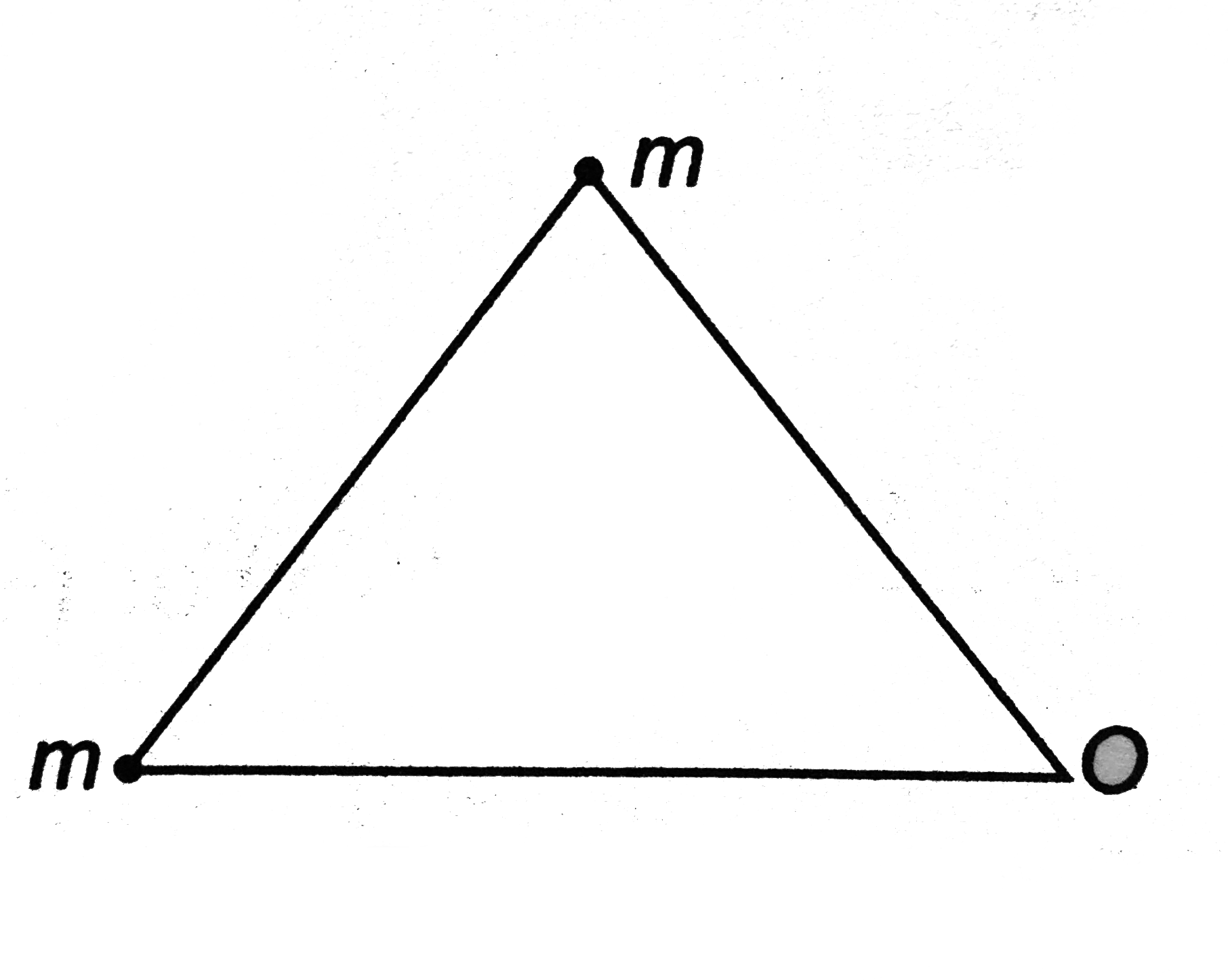
Find gravitational potential and magnitude of field strength at O.
View SolutionFive point charges each of charge +q are placed on five vertices of a regular hexagon of side h as shown in the figure. Then
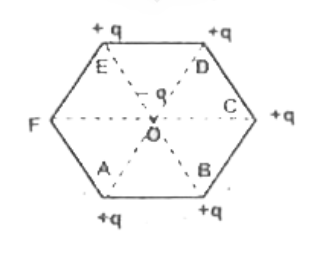
View SolutionFive identical charges are kept at five vertices of a regular hexagon. Match the following two columns at centre of the hexagon. If in the given situation electric field at centre is E, then,

View SolutionShow that any four vertices of a regular pentagon form a cyclic quadrilateral.
View Solution
