What can be said about the centre of mass of a solid hemisphere of radius r without making any calculation. Will its distance from the centre be more than r/2 or less than r/2?
The correct Answer is:A, B
To determine the position of the center of mass of a solid hemisphere of radius r without performing any calculations, we can analyze the distribution of mass within the hemisphere.
1. Visualize the Hemisphere:
- Consider a solid hemisphere placed on a flat surface. The flat circular face is at the bottom, and the curved surface is on top.
2. Understand the Mass Distribution:
- The hemisphere can be divided into two regions: the upper half (the curved part) and the lower half (the flat circular base).
- The mass distribution is not uniform; more mass is concentrated in the upper part of the hemisphere compared to the lower flat circular base.
3. Identify the Center of Mass:
- The center of mass of an object is the point where the mass is evenly distributed in all directions.
- In the case of the hemisphere, since the upper region has more mass, the center of mass will be located closer to this region.
4. Compare Distances:
- The center of mass will be at a distance from the flat circular base. Since the upper region has more mass, the center of mass will be located above the midpoint of the radius.
- The midpoint of the radius is at r2. However, because the upper part has more mass, the center of mass will be positioned at a distance less than r2 from the flat base.
5. Conclusion:
- Therefore, we can conclude that the distance of the center of mass of the solid hemisphere from the center (the flat circular face) is less than r2.
Final Answer:
The distance from the center of a solid hemisphere to its center of mass is less than r2.
- Consider a solid hemisphere placed on a flat surface. The flat circular face is at the bottom, and the curved surface is on top.
- The hemisphere can be divided into two regions: the upper half (the curved part) and the lower half (the flat circular base).
- The mass distribution is not uniform; more mass is concentrated in the upper part of the hemisphere compared to the lower flat circular base.
- The center of mass of an object is the point where the mass is evenly distributed in all directions.
- In the case of the hemisphere, since the upper region has more mass, the center of mass will be located closer to this region.
- The center of mass will be at a distance from the flat circular base. Since the upper region has more mass, the center of mass will be located above the midpoint of the radius.
- The midpoint of the radius is at r2. However, because the upper part has more mass, the center of mass will be positioned at a distance less than r2 from the flat base.
- Therefore, we can conclude that the distance of the center of mass of the solid hemisphere from the center (the flat circular face) is less than r2.
The distance from the center of a solid hemisphere to its center of mass is less than r2.
Topper's Solved these Questions
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBook:DC PANDEY ENGLISHChapter:CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONExercise:Exercise 11.2
Explore 6 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBook:DC PANDEY ENGLISHChapter:CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONExercise:Exercise 11.3
Explore 7 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBook:DC PANDEY ENGLISHChapter:CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONExercise:MiscellaneousExamples
Explore 9 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBook:DC PANDEY ENGLISHChapter:CENTRE OF MASS, IMPULSE AND MOMENTUMExercise:Comprehension type questions
Explore 15 Videos
- CIRCULAR MOTIONBook:DC PANDEY ENGLISHChapter:CIRCULAR MOTIONExercise:Medical entrances s gallery
Explore 19 Videos
Similar Questions
Knowledge Check
The radius of gyration of a solid sphere of radius R about a certain axis is also equal to R. If r is the distance between the axis and the centre of the sphere, then r is equal to
ARB0.5RC√0.6RD√0.3R
Find distance of centre of mass of solid hemisphere of radius 8cm from centre
View SolutionFind distance of centre of mass of solid hemisphere of radius 8cm from centre
View SolutionThe radius of gyration of a solid shapere of radius r about a certain axis is r. The distance of this axis from the centre of the shpere is
View SolutionThe radius of gyration of a uniform solid sphere of radius R., about an axis passing through a point R2 away from the centre of the sphere is:
View SolutionAt what distance 'd' from the surface of a solid sphere of radius 'R',
(a)potential is sme as at a distance R2 from the centre ?
(b) field strengt is ame as at a distance R4 from centre.
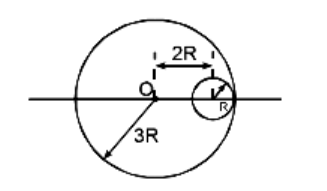
View SolutionIn the figure shown, find out centre of mass of a system of a uniform circular plate of radius 3R from O in which a hole of radius R is cut whose centre is at 2R distance from the centre of large circular plate
View SolutionCalculate the gravitational field intensity and potential at the centre of the base of a solid hemisphere of mass m, radius R
View Solution