A projectile of mass 3kg is projected with velocity 50m/s at 37∘ from horizontal. After 2s, explosion takes place and the projectile breaks into two parts of masses 1kg and 2kg. The first part comes to rest just after explosion.
Find,
(a) the velocity of second part just after explosion.
(b) maximum height attained by this part. Take g=10m/s2
The correct Answer is:A
To solve the problem step by step, we will break it down into two parts as requested: (a) finding the velocity of the second part just after the explosion, and (b) calculating the maximum height attained by this part.
1. Resolve the initial velocity into components:
- Horizontal component (u_x) = u * cos(θ) = 50 * cos(37°) = 50 * (4/5) = 40 m/s
- Vertical component (u_y) = u * sin(θ) = 50 * sin(37°) = 50 * (3/5) = 30 m/s
2. Calculate the velocity after 2 seconds:
- The horizontal velocity remains constant: vx=ux=40m/s
- The vertical velocity after 2 seconds (using vy=uy−g⋅t):
vy=30−10⋅2=30−20=10m/s
- Therefore, the velocity vector just before the explosion is:
→v=40^i+10^jm/s
3. Apply conservation of momentum:
- Before the explosion, the total momentum is:
Total momentum=m⋅→v=3⋅(40^i+10^j)=120^i+30^jkg m/s
- After the explosion, the first part (1 kg) comes to rest, so its momentum is:
Momentum of first part=1⋅0=0kg m/s
- Let the velocity of the second part (2 kg) be →v2. Therefore, we have:
120^i+30^j=0+2⋅→v2
- Solving for →v2:
→v2=120^i+30^j2=60^i+15^jm/s
Answer for Part (a): The velocity of the second part just after the explosion is 60^i+15^jm/s.
Part (b): Maximum height attained by the second part
1. Calculate the height at which the explosion occurs:
- Use the vertical displacement formula:
h=uy⋅t−12gt2
h=30⋅2−12⋅10⋅(22)=60−20=40m
2. Calculate the additional height gained by the second part after the explosion:
- The vertical component of the velocity of the second part is 15m/s.
- Use the formula for maximum height gained after the explosion:
hadditional=v2y2g=1522⋅10=22520=11.25m
3. Total maximum height attained by the second part:
- Total height = height at explosion + additional height:
Total height=40+11.25=51.25m
Answer for Part (b): The maximum height attained by the second part is 51.25m.
- Horizontal component (u_x) = u * cos(θ) = 50 * cos(37°) = 50 * (4/5) = 40 m/s
- Vertical component (u_y) = u * sin(θ) = 50 * sin(37°) = 50 * (3/5) = 30 m/s
- The horizontal velocity remains constant: vx=ux=40m/s
- The vertical velocity after 2 seconds (using vy=uy−g⋅t):
vy=30−10⋅2=30−20=10m/s
- Therefore, the velocity vector just before the explosion is:
→v=40^i+10^jm/s
- Before the explosion, the total momentum is:
Total momentum=m⋅→v=3⋅(40^i+10^j)=120^i+30^jkg m/s
- After the explosion, the first part (1 kg) comes to rest, so its momentum is:
Momentum of first part=1⋅0=0kg m/s
- Let the velocity of the second part (2 kg) be →v2. Therefore, we have:
120^i+30^j=0+2⋅→v2
- Solving for →v2:
→v2=120^i+30^j2=60^i+15^jm/s
- Use the vertical displacement formula:
h=uy⋅t−12gt2
h=30⋅2−12⋅10⋅(22)=60−20=40m
- The vertical component of the velocity of the second part is 15m/s.
- Use the formula for maximum height gained after the explosion:
hadditional=v2y2g=1522⋅10=22520=11.25m
- Total height = height at explosion + additional height:
Total height=40+11.25=51.25m
Topper's Solved these Questions
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - MiscellaneousExamples
9 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Exercise 11.1
13 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Type 1
1 Video
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Medical entrances s gallery
19 Videos
Similar Questions
A particle is projected from ground with velocity 50m/s at 37∘ from horizontal. Find velocity and displacement after 2 s. sin37∘=35.
View SolutionA stationary bomb explode into two parts of masses 3kg and 1kg. The total KE of the two parts after explosioons is 2400J. The KE of the smaller part is
View SolutionThe time of flight of a projectile is 10 s and range is 500m. Maximum height attained by it is [g=10m/s2]
View SolutionThe time of flight of a projectile is 10 s and range is 500m. Maximum height attained by it is [g=10m/s2]
View SolutionA particle of mass 2m is projected at an angle of 45∘ with horizontal with a velocity of 20√2m/s. After 1s explosion takes place and the particle is broken into two equal pieces. As a result of explosion one part comes to rest. Find the maximum height attained by the other part. Take g=10m/s2.
View SolutionA particle of mass 2m is projected at an angle of 45∘ with horizontal with a velocity of 20√2m/s. After 1s explosion takes place and the particle is broken into two equal pieces. As a result of explosion one part comes to rest. Find the maximum height attained by the other part. Take g=10m/s2.
View SolutionA particle of mass 2m is projected at an angle of 45∘ with horizontal with a velocity of 20√2m/s. After 1s explosion takes place and the particle is broken into two equal pieces. As a result of explosion one part comes to rest. Find the maximum height attained by the other part. Take g=10m/s2.
View SolutionA ball of mass 200g is projected with a density of 30m/s at 30∘ from horizontal. Using the concept of impulse, find change in velocity in 2s. Take g=10m/s2.
View SolutionA projectile is fired at a speed of 100 m/s at an angel of 370 above the horizontal. At the highest point, the projectile breaks into two parts of mass ratio 1:3 the smaller coming to rest. Find the distance from the launching point to the where the heavier piece lands.
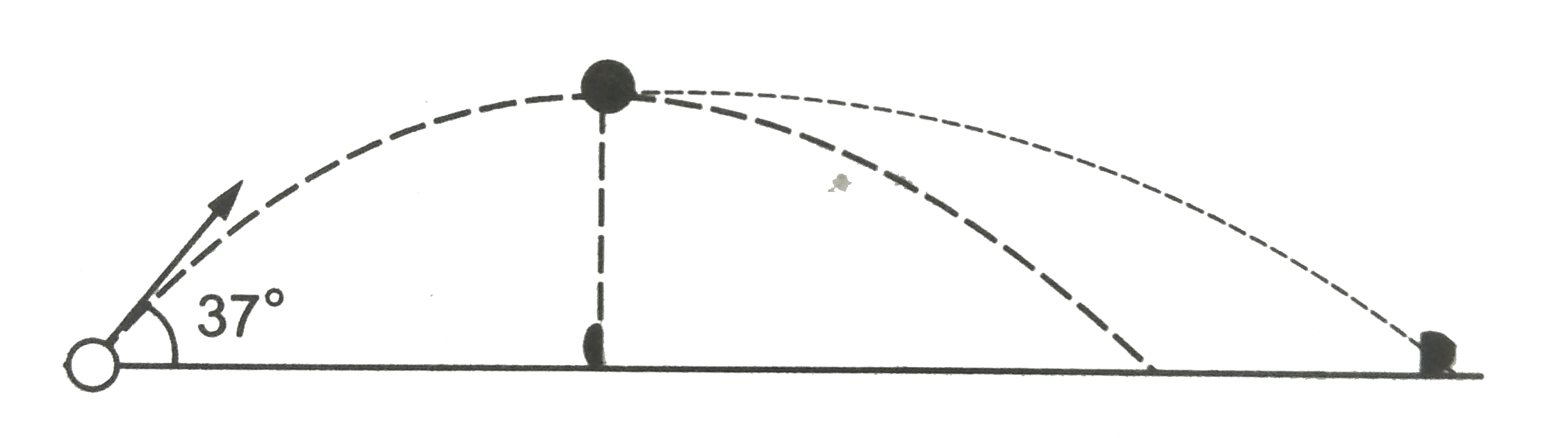
View SolutionA projectile is fired at a spedd of 100 m/s at an angel of 370 above the horizontal. At the highest point, the projectile breaks into two parts of mass ratio 1:3 the smaller coming to rest. Findthe distance from the launching point to the where the heavier piece lands.

View Solution
