A ball of mass 1 kg is dropped from a tower. Find power of gravitational force at time t=2s. Take g=10m/s^(2).
The correct Answer is:B
To solve the problem of finding the power of the gravitational force acting on a ball of mass 1 kg dropped from a tower at time t=2 seconds, we can follow these steps:
Step 1: Identify the given values
- Mass of the ball, m=1kg
- Acceleration due to gravity, g=10m/s2
- Time, t=2s
Step 2: Calculate the velocity of the ball at t=2s
Since the ball is dropped from rest, we can use the equation of motion:
v=u+gt
where u is the initial velocity (which is 0 since the ball is dropped).
Substituting the values:
v=0+(10m/s2)(2s)=20m/s
Step 3: Calculate the gravitational force acting on the ball
The gravitational force F can be calculated using the formula:
F=mg
Substituting the values:
F=(1kg)(10m/s2)=10N
Step 4: Calculate the power of the gravitational force
The power P can be calculated using the formula:
P=F⋅v⋅cos(θ)
Since the force and the velocity are in the same direction (downward), the angle θ=0∘ and cos(0∘)=1.
Thus, the power becomes:
P=F⋅v⋅1=F⋅v
Substituting the values:
P=(10N)(20m/s)=200W
Final Answer
The power of the gravitational force at t=2s is 200W.
---
- Mass of the ball, m=1kg
- Acceleration due to gravity, g=10m/s2
- Time, t=2s
Since the ball is dropped from rest, we can use the equation of motion:
v=u+gt
where u is the initial velocity (which is 0 since the ball is dropped).
v=0+(10m/s2)(2s)=20m/s
The gravitational force F can be calculated using the formula:
F=mg
Substituting the values:
F=(1kg)(10m/s2)=10N
The power P can be calculated using the formula:
P=F⋅v⋅cos(θ)
Since the force and the velocity are in the same direction (downward), the angle θ=0∘ and cos(0∘)=1.
P=F⋅v⋅1=F⋅v
Substituting the values:
P=(10N)(20m/s)=200W
The power of the gravitational force at t=2s is 200W.
Topper's Solved these Questions
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Solved Examples
12 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - TYPE2
1 Video
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Integer Type Question
11 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRACES GALLERY
33 Videos
Similar Questions
A body of mass 10 kg is dropped to the ground from a height of 10 metres. The work done by the gravitational force is (g=9.8m/sec2)
View SolutionA ball of mass 1 kg is dropped from 20 m height on ground and it rebounds to height 5m. Find magnitude of change in momentum during its collision with the ground. (Take g=10m/s2)
View SolutionA ball of mass 50 g is dropped from a height of 20 m. A boy on the ground hits the ball vertically upwards with a bat with an average force of 200 N, so that it attains a vertical height of 45 m. The time for which the ball remains in contact with the bat is [Take g=10m/s2]
View SolutionA ball of mass 1 kg is dropped from height 9.8 m, strikes with ground and rebounds at height 4.9 m, if the time of contact between ball and ground is 0.1 s then find impulse and average force acting on ball.
View SolutionA ball is projected vertically up with speed 20 m/s. Take g=10m/s2
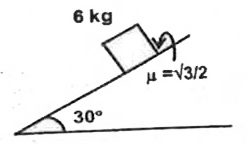
View SolutionA block of mass 6 kg is kept on rough surface as shown in figure. Find acceleration and friction force acting on the block. (Take g=10m/s2)
View SolutionA ball of mass 50 gm is dropped from a height h=10m. It rebounds losing 75 percent of its kinetic energy. If it remains in contanct with the ground for Δt=0.01 sec. the impulse of the impact force is (take g=10m/s2)
View SolutionA ball is dropped from a tower of height h under gravity. If it takes 4s to reach the ground from height h2, then time taken by it to reach from h to h2 is nearly:
View SolutionA stone is dropped freely from the top of a tower and it reaches the ground in 4 s. Taking g = 10 m s−2, calculate the height of the tower.
View SolutionA block of mass 0.2 kg is attached to a mass less spring of force constant 80 N/m as shown in figure. Find the period of oscillation. Take g=10m/s2. Neglect friction

View Solution
