The speed of a train increases at a constant rate α from zero to v and then remains constant for an interval and finally decreases to zero at a constant rate β. The total distance travelled by the train is l. The time taken to complete the journey is t. Then,
t=l(α+β)αβ
t=lv+v2(1α+1β)
t is minimum when v=√2lαβα−β
t is minimum when v=√2lαβα+β
The correct Answer is:B, D
To solve the problem step-by-step, we will analyze the motion of the train in three phases: acceleration, constant speed, and deceleration.
Step 1: Understanding the Phases of Motion
The train's journey can be divided into three distinct phases:
1. Acceleration Phase: The train accelerates from rest (0) to speed v at a constant rate α.
2. Constant Speed Phase: The train moves at a constant speed v for a certain time t2.
3. Deceleration Phase: The train decelerates from speed v to rest (0) at a constant rate β.
Step 2: Calculate Time for Each Phase
1. Acceleration Phase:
- The time taken to accelerate from 0 to v is given by:
t1=vα
2. Constant Speed Phase:
- The time taken at constant speed v is t2.
3. Deceleration Phase:
- The time taken to decelerate from v to 0 is given by:
t3=vβ
Step 3: Total Time
The total time t for the journey is the sum of the times for each phase:
t=t1+t2+t3=vα+t2+vβ
Step 4: Calculate Distances for Each Phase
1. Distance during Acceleration Phase:
- The distance covered while accelerating is:
d1=12vt1=12v(vα)=v22α
2. Distance during Constant Speed Phase:
- The distance covered at constant speed is:
d2=v⋅t2
3. Distance during Deceleration Phase:
- The distance covered while decelerating is:
d3=12vt3=12v(vβ)=v22β
Step 5: Total Distance
The total distance L covered by the train is the sum of the distances for each phase:
L=d1+d2+d3=v22α+vt2+v22β
Step 6: Rearranging the Equations
From the total distance equation, we can express t2:
L=v22α+vt2+v22β
Rearranging gives:
vt2=L−(v22α+v22β)
Thus,
t2=L−(v22α+v22β)v
Step 7: Substitute t2 into Total Time Equation
Substituting t2 back into the total time equation:
t=vα+L−(v22α+v22β)v+vβ
Step 8: Final Relationships
After simplification, we can derive the relationships between t, L, α, β, and v.
The train's journey can be divided into three distinct phases:
1. Acceleration Phase: The train accelerates from rest (0) to speed v at a constant rate α.
2. Constant Speed Phase: The train moves at a constant speed v for a certain time t2.
3. Deceleration Phase: The train decelerates from speed v to rest (0) at a constant rate β.
1. Acceleration Phase:
- The time taken to accelerate from 0 to v is given by:
t1=vα
- The time taken at constant speed v is t2.
- The time taken to decelerate from v to 0 is given by:
t3=vβ
The total time t for the journey is the sum of the times for each phase:
t=t1+t2+t3=vα+t2+vβ
1. Distance during Acceleration Phase:
- The distance covered while accelerating is:
d1=12vt1=12v(vα)=v22α
- The distance covered at constant speed is:
d2=v⋅t2
- The distance covered while decelerating is:
d3=12vt3=12v(vβ)=v22β
The total distance L covered by the train is the sum of the distances for each phase:
L=d1+d2+d3=v22α+vt2+v22β
From the total distance equation, we can express t2:
L=v22α+vt2+v22β
Rearranging gives:
vt2=L−(v22α+v22β)
Thus,
t2=L−(v22α+v22β)v
Substituting t2 back into the total time equation:
t=vα+L−(v22α+v22β)v+vβ
After simplification, we can derive the relationships between t, L, α, β, and v.
Topper's Solved these Questions
- KINEMATICSBook:DC PANDEY ENGLISHChapter:KINEMATICSExercise:Comprehension
Explore 7 Videos
- KINEMATICSBook:DC PANDEY ENGLISHChapter:KINEMATICSExercise:Subjective Questions
Explore 24 Videos
- KINEMATICSBook:DC PANDEY ENGLISHChapter:KINEMATICSExercise:Subjective
Explore 50 Videos
- GRAVITATIONBook:DC PANDEY ENGLISHChapter:GRAVITATIONExercise:(C) Chapter Exercises
Explore 45 Videos
- KINEMATICS 1Book:DC PANDEY ENGLISHChapter:KINEMATICS 1Exercise:INTEGER_TYPE
Explore 15 Videos
Similar Questions
The speed of a car increases uniformly from zero to 10ms−1 in 2s and then remains constant (figure)
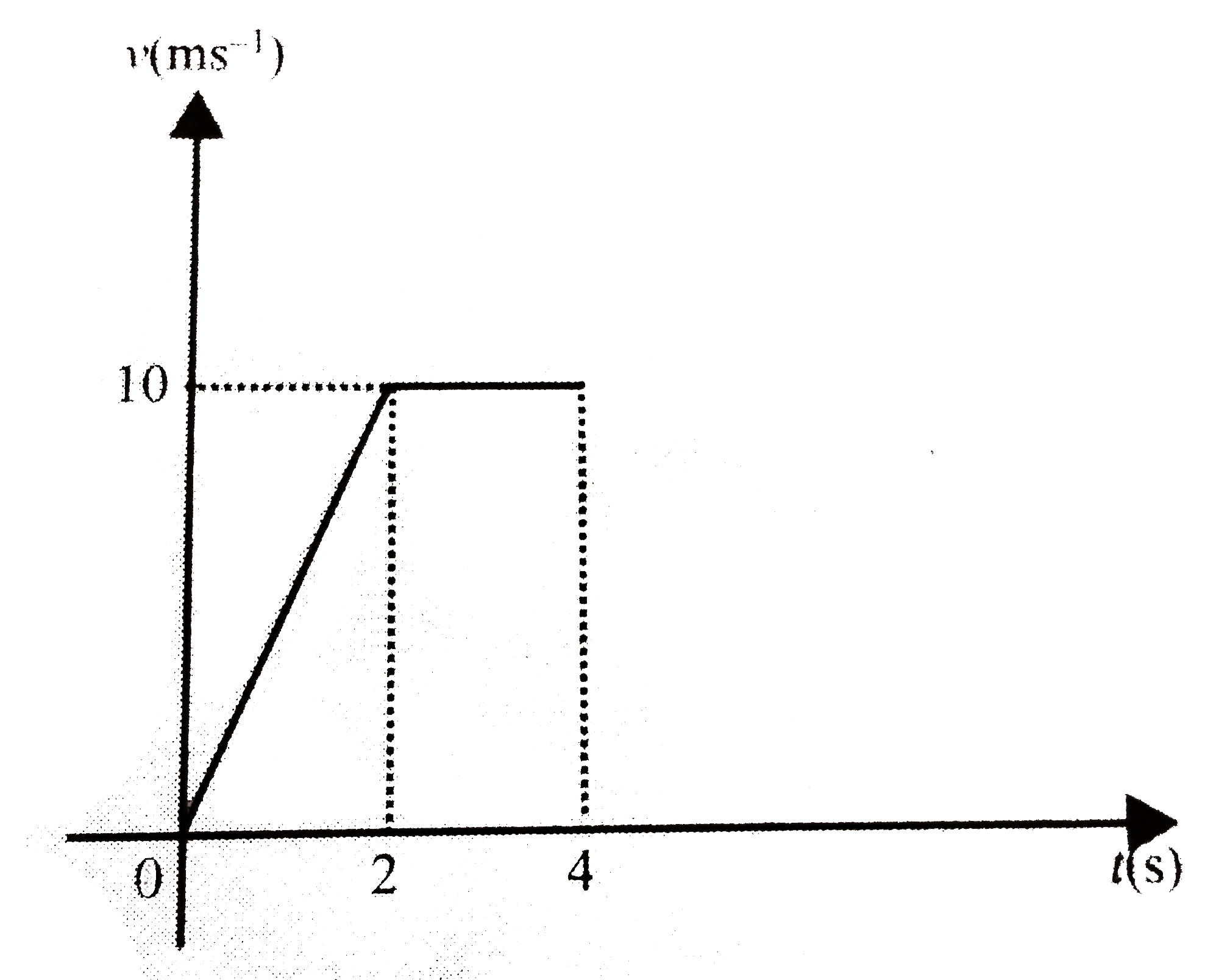
a. Find the distance travelled by the car in the first two seconds.
b. Find the distance travelled by the car in the next two seconds.
c. Find the total distance travelled in 4s.
View SolutionA car, starating from rest, accelerates at the rate f through a distance S, then continoues at constant speed for time t and then decelerates as the rate f/2 to come to rest. If the total distance travelled is 15 S, then
View Solution
Knowledge Check
A train accelerates from rest at a constant rate α for some time and then it retards to rest at the constant rate β. If the total distance covered by the train is s, then the velocity of the train is
A[α+β2αβ×s]1/2B[α−β2αβ×s]1/2C[αβα+β×s]1/2D[αβα−β×s]1/2A train travels at a constant rate. If the train takes 13 minutes to travel m kilometers, how long will the train take to travel n kilometers?
A13mnB13nmC13mnDm13
A bus starting from rest, accelerates at the rate of f through a distance S, then continues at constant speed for time t and declerates at the rate f/2 to come to rest. If the total distance travelled is 15 S then S =
View SolutionA car, starting from rest, accelerates at the rate f through a distance s, then continues at constant speed for time t and then decelerates at the rate f/ 2 to come to rest. If the total distance travelled is 15 s, then
View SolutionA train accelerates from rest at a constant rate a for time t1and then it retards at the constant rate b for time t2 then comes to rest. Find the ratio t1/t2.
View SolutionA car accelerates from rest at a constant rate 'alpha' for some time after which it decelerates at a constant rate β to come to rest. If the total time elapsed is t, the maximum velocity acquired by the car is given by :
View SolutionA lift ascends with constant acceleration a=1ms−2, then with constant velocity and finally, it stops under constant retardation a=1ms−2. If total distance ascended by the lift is 7 m, in a total time of the journey is 8 s. Find the time (in second) for which lift moves with constant velocity.
View SolutionA particle, starting from rest, moves in a straight line with constant acceleration a0 for a time interval t0.Then is accelerates at a constant rate 2a0 for time interval 2t0 .The distance travelled by the particle in time 3t0 from the starts is S . Then Sa0t20=
View Solution