A particle is moving in x-y plane. Its initial velocity and acceleration are u=(4ˆi+8ˆj)m/sanda=(2ˆi−4ˆj)m/s2. Find
(a) the time when the particle will cross the x-axis.
(b) x-coordinate of particle at this instant.
(c) velocity of the particle at this instant.
Initial coordinates of particle are (4m,10m).
The correct Answer is:B, D
To solve the problem step by step, we will break it down into three parts as specified in the question.
Given Data:
- Initial velocity, u = 4^i+8^j m/s
- Acceleration, a = 2^i−4^j m/s²
- Initial coordinates of the particle = (4 m, 10 m)
(a) Time when the particle will cross the x-axis
To find the time when the particle crosses the x-axis, we need to determine when the y-coordinate becomes zero. The equation of motion in the y-direction is given by:
sy=uyt+12ayt2
Where:
- sy = displacement in the y-direction
- uy = initial velocity in the y-direction = 8 m/s
- ay = acceleration in the y-direction = -4 m/s²
The initial y-coordinate is 10 m, and we want to find the time when the particle crosses the x-axis (y = 0). Thus, the displacement sy will be:
sy=0−10=−10 m
Substituting the values into the equation:
−10=8t+12(−4)t2
This simplifies to:
−10=8t−2t2
Rearranging gives:
2t2−8t−10=0
Dividing the entire equation by 2:
t2−4t−5=0
Now, we can factor the quadratic equation:
(t−5)(t+1)=0
This gives us two solutions:
t=5andt=−1
Since time cannot be negative, we have:
t=5 seconds
(b) x-coordinate of the particle at this instant
To find the x-coordinate when t=5 seconds, we use the equation of motion in the x-direction:
sx=uxt+12axt2
Where:
- ux = initial velocity in the x-direction = 4 m/s
- ax = acceleration in the x-direction = 2 m/s²
The initial x-coordinate is 4 m. Thus,
sx=xi+uxt+12axt2
Substituting the values:
x=4+4(5)+12(2)(52)
Calculating:
x=4+20+12(2)(25)
x=4+20+25
x=49 m
(c) Velocity of the particle at this instant
The velocity of the particle at time t is given by:
v=u+at
Substituting the values:
v=(4^i+8^j)+(2^i−4^j)⋅5
Calculating:
v=(4+10)^i+(8−20)^j
v=14^i−12^j m/s
Summary of Results:
- (a) Time when the particle crosses the x-axis: 5 seconds
- (b) x-coordinate at this instant: 49 m
- (c) Velocity at this instant: 14 i - 12 j m/s
- Initial velocity, u = 4^i+8^j m/s
- Acceleration, a = 2^i−4^j m/s²
- Initial coordinates of the particle = (4 m, 10 m)
- sy = displacement in the y-direction
- uy = initial velocity in the y-direction = 8 m/s
- ay = acceleration in the y-direction = -4 m/s²
- ux = initial velocity in the x-direction = 4 m/s
- ax = acceleration in the x-direction = 2 m/s²
x=4+20+25
x=49 m
v=14^i−12^j m/s
- (a) Time when the particle crosses the x-axis: 5 seconds
- (b) x-coordinate at this instant: 49 m
- (c) Velocity at this instant: 14 i - 12 j m/s
Topper's Solved these Questions
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Example Type 5
1 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Example Type 6
1 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Example Type 3
1 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises
45 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
Similar Questions
Velocity and acceleration of a particle are v=(2ˆi−4ˆj)ms and a=(−2ˆi+4ˆj)ms2 Which type of motion is this?
View SolutionVelocity and acceleration of a particle are v=(2ˆi)ms and a=(4tˆi+t2ˆj)ms2 where, t is the time. Which type of motion is this ?
View SolutionVelocity and acceleration of a particle at time t=0are u=(2ˆi+3ˆj)m/sanda=(4ˆi+3ˆj)m/s2 respectively. Find the velocity and displacement if particle at t=2s.
View SolutionA particle has an initial velocity 3ˆi+3ˆj and acceleration of 0.41ˆi+0.3ˆj. Its speed after 10s is
View SolutionA particle is moving in x-y plane. At an instant, it has velocity (4ˆi+4ˆj)m/s and acceleration (3ˆi+5ˆj)m/s2 At that instant, the radius of curvature of its path will be :
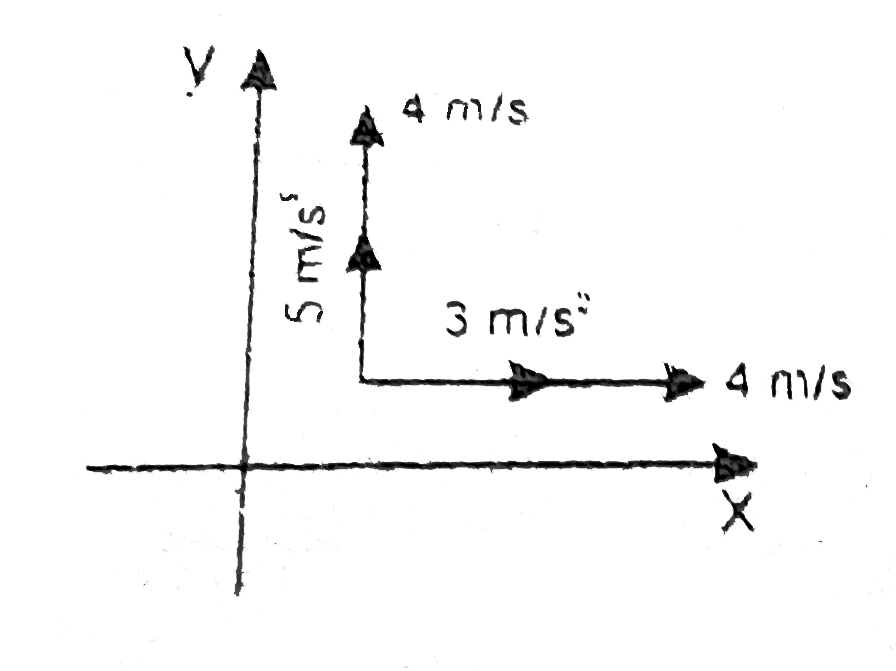
View SolutionA particle has an initial velocity of 4ˆi+3ˆj and an acceleration of 0.4ˆi+0.3ˆj. Its speed after 10s is
View SolutionA particle at origin (0,0) moving with initial velocity u=5msˆj and acceleration 10ˆi+4ˆj. After t time it reaches at position (20,y) then find t & y
View SolutionVelocity of a particle at some instant is v=(3ˆi+4ˆj+5ˆk)m/s. Find speed of the particle at this instant.
View SolutionA particle is moving in such a way that at one instant velocity vector of the particle is 3ˆi+4ˆjm/s and acceleration vector is −25ˆi−25ˆjm/s? The radius of curvature of the trajectory of the particle at that instant is
View SolutionVelocity and acceleration of a particle at some instant of time are v=(3ˆi+4ˆj)ms−1 and a=−(6ˆi+8ˆj)ms−2 respectively. At the same instant particle is at origin. Maximum x-coordinate of particle will be
View Solution
