Question

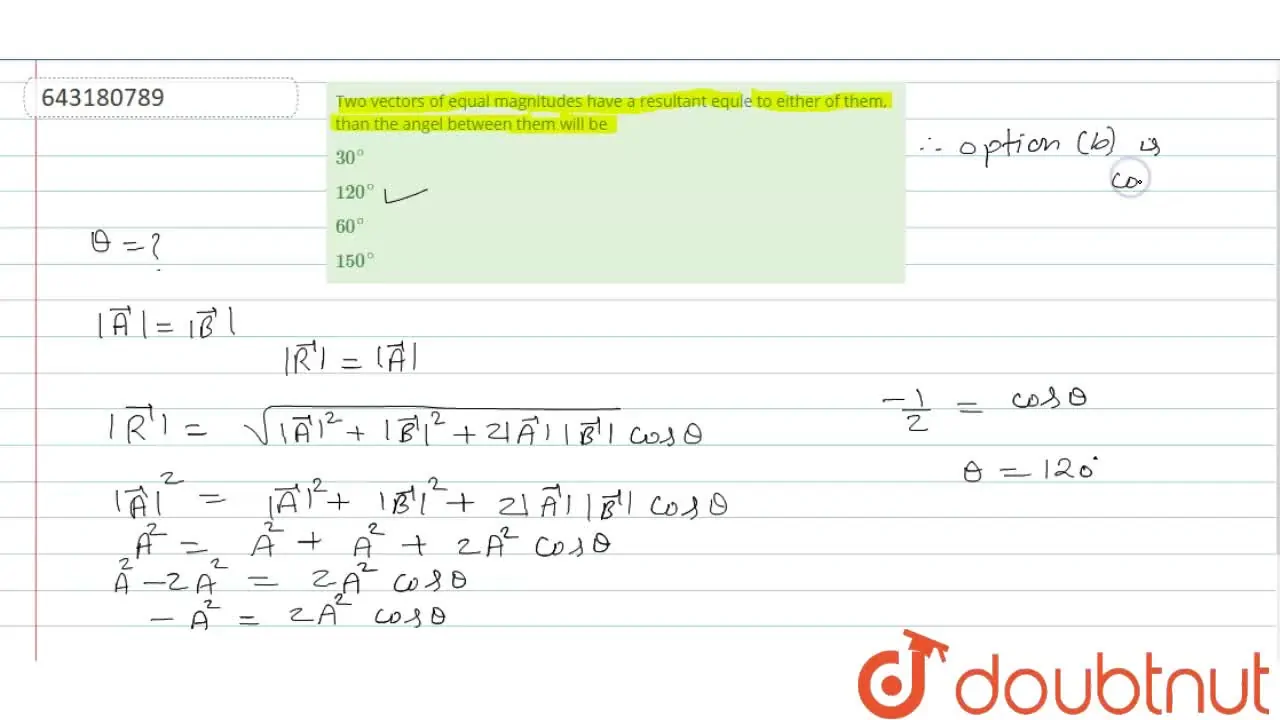
Two vectors of equal magnitudes have a resultant equle to either of them, than the angel between them will be

Options

A. `30^@`
B. `120^@`
C. `60^@`
D. `150^@`

Answer

B

Explanation

To solve the problem, we need to determine the angle between two vectors of equal magnitudes when their resultant is equal to either of them. Let's denote the vectors as A and B, both having the same magnitude A.

1. Understanding the Problem:
- We have two vectors A and B with equal magnitudes: |A| = |B| = A.
- The resultant of these two vectors is equal to the magnitude of either vector.

2. Using the Formula for Resultant:
- The formula for the resultant R of two vectors A and B is given by:
\( R = \sqrt{A^2 + B^2 + 2AB \cos(\theta)} \)
- Since |A| = |B| = A, we can substitute:
\( R = \sqrt{A^2 + A^2 + 2A \cdot A \cos(\theta)} = \sqrt{2A^2 + 2A^2 \cos(\theta)} \)

3. Setting the Resultant Equal to the Magnitude of the Vectors:
- According to the problem, the resultant is equal to the magnitude of either vector:
\( R = A \)
- Therefore, we can set up the equation:
\( A = \sqrt{2A^2 + 2A^2 \cos(\theta)} \)

4. Squaring Both Sides:
- To eliminate the square root, we square both sides:
\( A^2 = 2A^2 + 2A^2 \cos(\theta) \)

5. Rearranging the Equation:
- Rearranging gives:
\( A^2 - 2A^2 = 2A^2 \cos(\theta) \)
- Simplifying this, we have:
\( -A^2 = 2A^2 \cos(\theta) \)

6. Dividing by A^2:
- Dividing both sides by A^2 (assuming A ≠ 0):
\( -1 = 2 \cos(\theta) \)

7. Solving for Cosine:
- Rearranging gives:
\( \cos(\theta) = -\frac{1}{2} \)

8. Finding the Angle:
- The angle whose cosine is -1/2 is:
\( \theta = 120^\circ \)

Conclusion:
The angle between the two vectors is 120 degrees.