Find the domains of the following functions
f(x)=1[x] ( [ ] denotes the GIF) Solution in Telugu
The correct Answer is:R-[0,1)
Step by step video, text & image solution for Find the domains of the following functions f(x) = ( 1)/( [x]) ( [ ] denotes the GIF) by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A stone is dropped into a quiet pond and waves move in circles outward from the place where it strikes, at a speed of 30 cm per second. At the instant when the radius of the wave ring is 50 mt, find the rate of increase in the area and in the circumference of the wave ring.
View SolutionIn a triangle ABC, if A,B, C are in A.P and b:c =√3:√2, then
View SolutionIf the domain of y=f(x)is[−3,2], then find the domain of g(x)=f(|[x]|),wher[] denotes the greatest integer function.
View SolutionFind the domain of each of the following functions: f(x)=ln[x2+x+1], where [.] GIF
View SolutionFind the domain and range of the following function:
f(x)=cos−1√log[x](|x|)x, whre [.] denotes the greatest integer function.
View SolutionFind the domain and range of the following function:
f(x)=log[x−1]sinx, where [ ] denotes greatest integer function.
View SolutionThe domain of function f(x)=cos−1x[x]; [x]=GIF is:
View SolutionThe domain of the function f(x) is denoted by Df
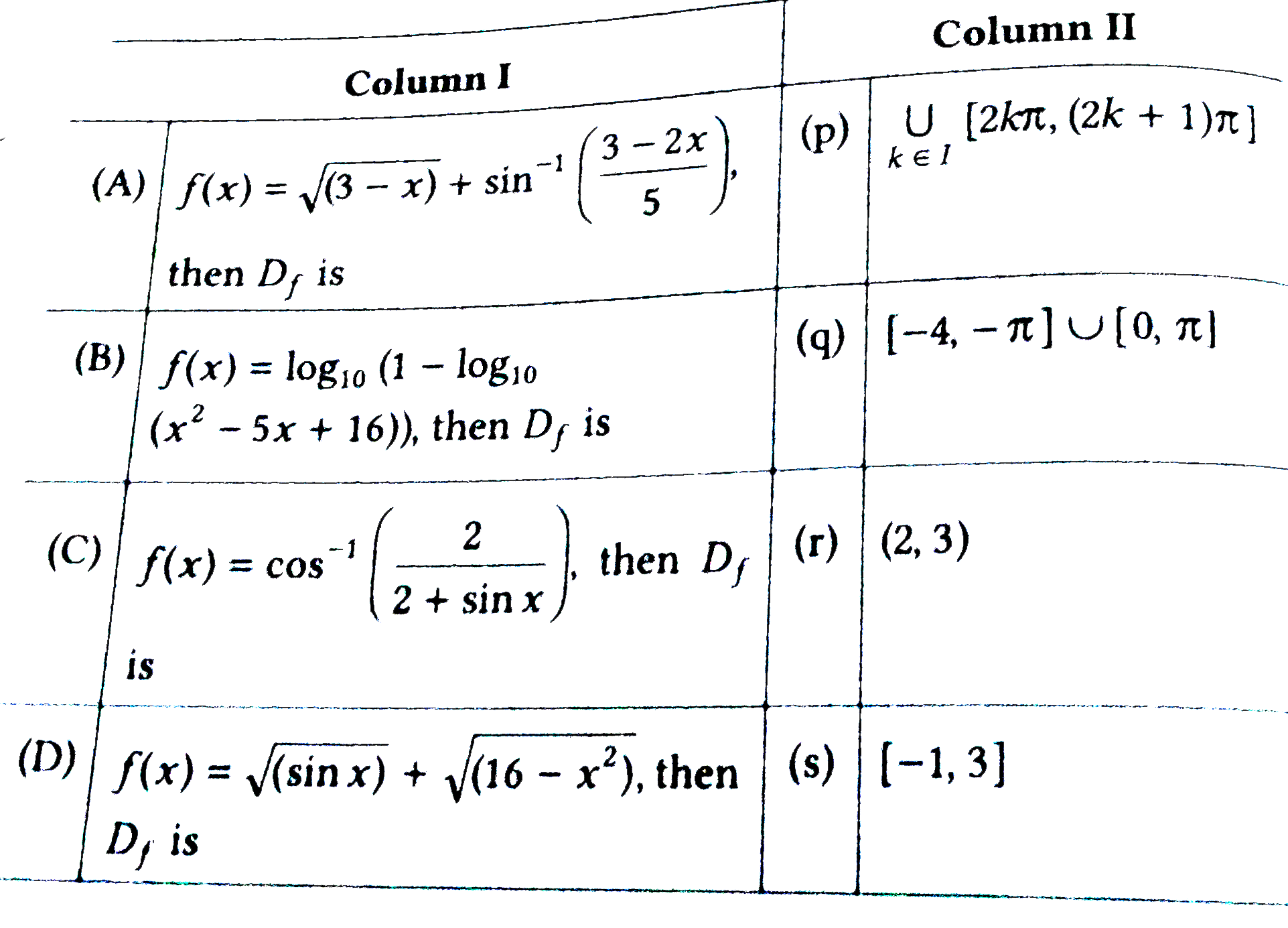
View Solutionf(x)=sin−1[2x2−3], where [⋅] denotes the greatest integer function. Find the domain of f(x).
View SolutionFor the function f(x)=log[x−1]sgn(x) where sgn(x) is the signum function and [x] is GIF, domain of f is.
View SolutionThe domain of the function f(x) is denoted by Df

View SolutionFind the domain of each of the following functions: f(x)=ln[x2+x+1], where [.] GIF
View SolutionFind the domain and range of the following function:
f(x)=log[x−1]sinx, where [ ] denotes greatest integer function.
View SolutionThe domain (प्रांत ) of function f(x)=cos−1x[x]; [x]=GIF is:
View SolutionFind the domain of the following function: f(x)=1[|x−1|]+[|12−x|]−11, where [x] denotes the greatest integer not greater than x.
View Solution
