Assertion : Pressure in bubble ( r ) in the atmosphere of pressure P0isP0+4σr where r is the radius and σ is surface tension .
Reason: 4σr is the excess pressure due to two surface exposed to the atmosphere . Solution in Marathi
Assertion is true , Reason is true ,s2 is correct explanation for Assetion .
Assertion is true , reason Is true Reason is not a correct explanation for Assertion .
Assertion is true , reason is false
Assetion is fales ,reason is true
The correct Answer is:B
Step by step video & image solution for Assertion : Pressure in bubble ( r ) in the atmosphere of pressure P_0 is P_0+(4sigma)/r where r is the radius and sigma is surface tension . Reason: (4sigma)/r is the excess pressure due to two surface exposed to the atmosphere . by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
If the surface tension (T) of a soap bubble depends upon excess of pressure radius 'r' of the bubble, then obtain an equation for the surface tension.
06:21View Solutionसिद्ध कीजिए कि पृष्ठ तनाव के कारण बूँद के अंदर अतिरिक्त दाब 2T/R होता है तथा साबुन के बुलबुले के अंदर अतिरिक्त दाब 4T/R होता है, जहाँ T द्रव का पृष्ठ तनाव तथा R बूँद या बुलबुले की त्रिज्या है |
04:24View SolutionA : Excess pressure inside a soap bubble is 4Tr symbols have their usual meanings).
R : The pressure difference across a curved surface of radius of curvature r is 2Tr). There are two surfaces in a soap bubble.05:04View SolutionFigure shows a capillary tube of radius r dipped into water. If the atmosphere pressure is P0, the pressure at point A is
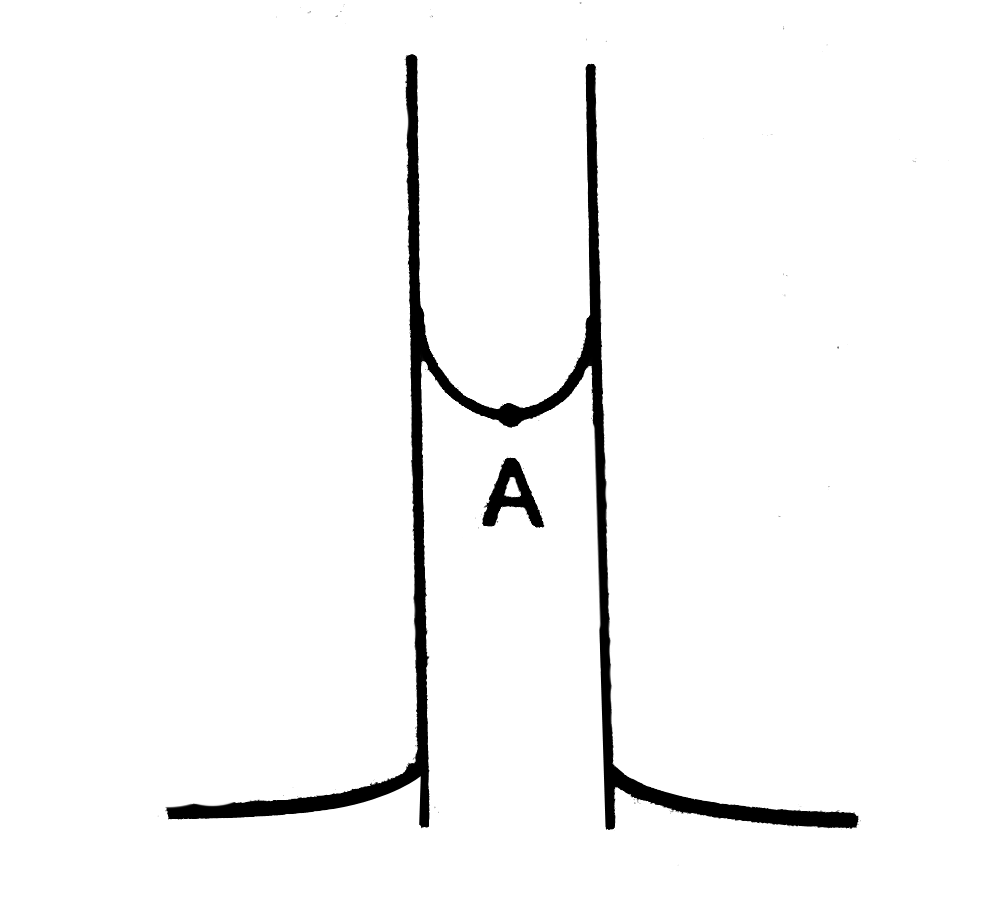 02:02View Solution
02:02View SolutionAn air bubble of radius 'r' is formed at a depth 'h' below the surface of water of density 'd' and if 'p0' is the atm pressure, T is surface tension of water, then the pressure inside the bubble is
View SolutionAn air bubble of radius 'r' is formed at a depth 'h' below the surface of water of density 'd' and if 'p0' is the atm pressure, T is surface tension of water, then the pressure inside the bubble is
View SolutionThe excess pressure inside a soap bubble of radius R is (S is the surface tension)
01:20View Solution

