A person buys a lottery ticket in 50 lotteries, in each of which his chance of winning a prize is 1100. What is the probability that he will win a prize (a) at least once (b) exactly once (c) at least twice?Solution in Gujrati
The correct Answer is:(a)1−(99100)50(b)12(99100)49(c)1−149100(99100)49
Step by step video, text & image solution for A person buys a lottery ticket in 50 lotteries, in each of which his chance of winning a prize is (1)/(100). What is the probability that he will win a prize (a) at least once (b) exactly once (c) at least twice? by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - KUMAR PRAKASHANCHAPTER - PROBABILITYEXERCISE - MISCELLANEOUS EXERCISE 1320videos
PROBABILITY
- BOOK - KUMAR PRAKASHANCHAPTER - PROBABILITYEXERCISE - Textbook Illustrations for Practice Work37videos
PROBABILITY
- BOOK - KUMAR PRAKASHANCHAPTER - PROBABILITYEXERCISE - EXERCISE -13.420videos
PROBABILITY
- BOOK - KUMAR PRAKASHANCHAPTER - MATRICES EXERCISE - Practice Paper - 3 (Section - D)1videos
MATRICES
- BOOK - KUMAR PRAKASHANCHAPTER - RELATIONS AND FUNCTIONS EXERCISE - Practice Paper - 1 (Section - D)2videos
RELATIONS AND FUNCTIONS
Similar Questions
A and B are two events such that P(A)≠0. Find P(B|A), if
(i) A is a subset of B (ii) A∩B=ϕ06:57View SolutionIf P(A)=713,P(B)=913 and P(A∩B)=413 evaluate P(A∣B)
04:52View SolutionState which of the following are not the probability distributions of a random variable. Give reasons for your answer.
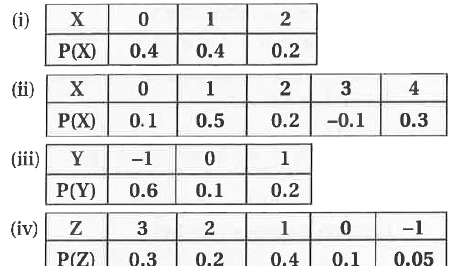 07:28View Solution
07:28View SolutionFor matrix A=⎡⎢⎣11112−32−13⎤⎥⎦ . Prove that , A3−6A2+5A+11I=0. Hence find A−1 using it .
View SolutionLet : f:N→N be defined by f(n)={n+12 if n is oddn2 if n is even for all n∈N .
State whether the function f is bijective . Justify your answer.04:46View SolutionA person buys a lottery ticket in 50 lotteries, in each of which his chance of winning a prize is 1100. What is the probability that he will win a prize(a) at least once (b) exactly once (c) at least twice?
04:52View SolutionA person buys a lottery ticket in 50 lotteries, in each of which his chance of winning and prize is 1100. What is the probability that he will win a prize.
(a) at least once
(b) exactly once04:05View SolutionA person buys a lottery ticket in 50 lotteries, in each of which his chance of winning a prize is 1100 . What is the probability that he will win a prize
a. atleast once
b. exactly once
c. atleast twice?06:57View SolutionA person buys a lottery ticket in 50 lotteries, in each of which his chance of winning a prize is 1100. What is the probability that he will win a prize (a) at least once (b) exactly once (c) at least twice?
06:01View SolutionA person buys a lottery ticket in 50 lotteries, in each of which his chance of winning a prize is 1100. What is the probability that he will win a prize (a) at least once (b) exactly once (c) at least twice?
04:22View SolutionA person buys a lottery ticket in 50 lotteries, in each of which his chance of winning and prize is 1100. What is the probability that he will win a prize.
(a) at least once
(b) exactly once03:27View SolutionA person buys a lottery ticket in 50 lotteries, in each of which his chance of winning a prize is 1100. What is the probability that he will win a prize (a) at least once (b) exactly once (c) at least twice?
04:30View SolutionA person buys a lottery ticket in 50 lotteries, in each of which his chance of winning a prize is 1100. What is the probability that he will win a prize(a) at least once (b) exactly once (c) at least twice?
05:27View SolutionA person buys a lottery ticket in 50 lotteries, in each of which his chance of winning a prize is 1100. What is the probability that he will win a prize(a) at least once (b) exactly once (c) at least twice?
04:17View SolutionA person buys a lottery ticket in 50 lotteries, in each of which his chance of winning a prize is 1100 . What is the probability that he will win a prize at least once.
04:03View SolutionA person buys a lottery ticket in 50 lotteries, in each of which his chance of winning a prize is 1100 . What is the probability that he will win a prize exactly once.
02:45View Solution
