यदि f(x)=4x3−x2−2x+1 तथा g(x)=[Minf{f(t)0≤t≤x}0≤x≤13−x1<x≤2 तब g(14)+g(34)+g(54) का मान है
74
94
134
52
The correct Answer is:D
Step by step video, text & image solution for यदि f(x) = 4x^(3) -x^(2) - 2x +1 तथा g(x) = [ {:("Min"f{f(t)0letlex},,0lexle1),(3-x,,1lt x le 2):} तब g((1)/(4)) + g((3)/(4)) + g((5)/(4)) का मान है by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MAXIMA-MINIMABOOK - ALLEN HINDICHAPTER - MAXIMA-MINIMAEXERCISE - EXERCISE-03 (MISCELLANEOUS TYPE QUESTIONS) (स्तम्भ सुमेलन)
2 Videos
- MAXIMA-MINIMABOOK - ALLEN HINDICHAPTER - MAXIMA-MINIMAEXERCISE - EXERCISE-03 (MISCELLANEOUS TYPE QUESTIONS) (कथन एवं कारण)
5 Videos
- MAXIMA-MINIMABOOK - ALLEN HINDICHAPTER - MAXIMA-MINIMAEXERCISE - EXERCISE-01 (CHECK YOUR GRAPS) (सही विकल्पों को चुनिए (एक या एक से अधिक सही उत्तर हैं))
4 Videos
- LIMIT BOOK - ALLEN HINDICHAPTER - LIMIT EXERCISE - Do yourself
29 Videos
- METHODS OF DIFFERENTIATIONBOOK - ALLEN HINDICHAPTER - METHODS OF DIFFERENTIATIONEXERCISE - EXERCISE - 05 [B]
12 Videos
Similar Questions
चार बिन्दु A, B, C तथा D परवलय y=ax2+bx+c पर क्रम से स्थित है तथा A, B व D के निर्देशांक A(-2.3), B(-1, 1), D(2,7) है।
उपरोक्त जानकारी के आधार पर निम्न को सुमेलित कीजिए
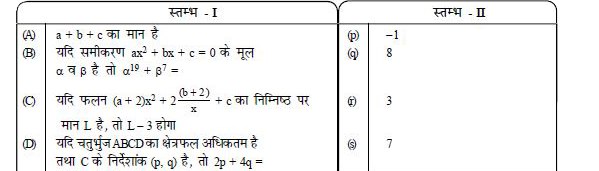
View Solutionकथन-I : माना फलन f(x)={−x2,x≤07x+8π≥0
क्योकिं
कथन-II : यदि f(a)<f(a−h) तथा f(a)<f(a+h) जहाँ 'h' पर्याप्त रूप से छोटा है, तब x) का स्थानीय निम्निष्ठ x=a पर होगा।
View Solutionयदि y=aln|x|+bx2+x का चरम मान x=−1 तथा x = 2 पर है, तो :
View Solutionफलन y = f (x) के आरेख के सापेक्ष निम्न में से कौन से कथन सत्य या असत्य होंगे :
limn→−1+f(x)=1

View Solutionयदि ln (x+y) = 2xy हो , तो y'(0) =
View SolutionIf f(x)=ax^(2)+bx+c and g(x)+px^(2)+qx with g(1)=f(1)g(2)-f(2)=1g(3)-f(3)=4 then g(4)-f(4) is
View Solutionif f(x)=4x^(3)-x^(2)-2x+1 and g(x)={min{f(t):0<=t<=x;0<=x<=1,3-x:1} then g(1/4)+g(3/4)+g(5/4) is equal to
View SolutionLet f(x)=1+x^(2) and f(g(x))=1+x^(2)-2x^(3)+x^(4). A possible value of g(3) is
View SolutionIf f(x)=4x3−x2−2x+1 and g(x)=min{f(t):0≤t≤x}:0≤x≤1 and g(x)=3−x:1≤x≤2 then g(14)+g(34)+g(54) is (A) 74 (B) 94 (C) 134 (D) 52
View SolutionSuppose F(x)=f(g(x)) and g(3)=5,g'(3)=3,f'(3)=1,f'(5)=4 Then F'(3)=
View SolutionLet f(x)=x3−x2+x+1 and g(x)={max f(t)0≤t≤x0≤x≤13−x1<x≤2 then
View SolutionIf g(x)=12[f(x)−f(−x)] defined over −3≤x≤3 and f(x)=2x2−4x+1, then the value of ∫3−3g(x)dx is -
View SolutionIf f(x)=4x3−x2−2x+1andg(x)={min{f(t):0≤t≤x}0≤x≤13−x1<x≤2 and if λ=g(14)+g(34)+g(54), then 2λ=
View SolutionGiven functions f(x)=2x+1andg(x)=x2−4, what is the value of f(g(−3))?
View Solutionif f(x)=4x3−x2−2x+1 and g(x)={minf(t):0≤t≤x;0≤x≤1,3−x:1} then g(14)+g(34)+g(54) is equal to
View SolutionThe two functions f (x) and g (x) are distinguishable in the interval [0,2], such that f(x)-g(x)=0,f(1)=2,g'(1)=4,f(2)=3,g(2)=9 , Then x=3//2 At f (x) -g (x) का मान है
View Solution
