For which of the following curves, the line x+√3y=2√3 is the tangent at the point (3√32,12) ?
x2+y2=7
y2=16√3x
2x2−18y2=9
x2+9y2=9
The correct Answer is:D
To determine which of the given curves has the line x+√3y=2√3 as a tangent at the point (3√32,12), we will analyze each option step by step.
Step 1: Identify the Tangent Line
The equation of the tangent line is given as:
x+√3y=2√3
We can rewrite it in slope-intercept form:
√3y=−x+2√3⟹y=−1√3x+2√3
The slope of the tangent line is −1√3.
Step 2: Check Each Curve Option
We will check each option to see if the tangent line is valid at the given point.
Option A: x2+9y2=9
1. Differentiate the equation implicitly:
2x+18ydydx=0⟹dydx=−2x18y=−x9y
2. Substitute the point (3√32,12):
dydx=−3√329⋅12=−3√39=−√33
This slope matches −1√3 (as −√33=−1√3), so this option is valid.
Option B: 2x2+18y2=9
1. Differentiate:
4x+36ydydx=0⟹dydx=−4x36y=−x9y
2. Substitute the point:
dydx=−3√329⋅12=−3√39=−√33
This slope also matches −1√3, so this option is valid.
Option C: y2=6√3x
1. Differentiate:
2ydydx=6√3⟹dydx=6√32y=3√3y
2. Substitute the point:
dydx=3√312=6√3
This slope does not match −1√3, so this option is invalid.
Option D: x2+y2=7
1. Differentiate:
2x+2ydydx=0⟹dydx=−xy
2. Substitute the point:
dydx=−3√3212=−3√3
This slope does not match −1√3, so this option is invalid.
Conclusion
The curves for which the line x+√3y=2√3 is the tangent at the point (3√32,12) are:
- Option A: x2+9y2=9
- Option B: 2x2+18y2=9
The equation of the tangent line is given as:
x+√3y=2√3
We can rewrite it in slope-intercept form:
√3y=−x+2√3⟹y=−1√3x+2√3
The slope of the tangent line is −1√3.
We will check each option to see if the tangent line is valid at the given point.
1. Differentiate the equation implicitly:
2x+18ydydx=0⟹dydx=−2x18y=−x9y
2. Substitute the point (3√32,12):
dydx=−3√329⋅12=−3√39=−√33
This slope matches −1√3 (as −√33=−1√3), so this option is valid.
1. Differentiate:
4x+36ydydx=0⟹dydx=−4x36y=−x9y
2. Substitute the point:
dydx=−3√329⋅12=−3√39=−√33
This slope also matches −1√3, so this option is valid.
1. Differentiate:
2ydydx=6√3⟹dydx=6√32y=3√3y
2. Substitute the point:
dydx=3√312=6√3
This slope does not match −1√3, so this option is invalid.
1. Differentiate:
2x+2ydydx=0⟹dydx=−xy
2. Substitute the point:
dydx=−3√3212=−3√3
This slope does not match −1√3, so this option is invalid.
The curves for which the line x+√3y=2√3 is the tangent at the point (3√32,12) are:
- Option A: x2+9y2=9
- Option B: 2x2+18y2=9
Topper's Solved these Questions
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-B
50 Videos
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - (SECTION - A)
20 Videos
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - Mathematic section B
10 Videos
- JEE MAINBOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINEXERCISE - QUESTION
1 Video
- JEE MAIN 2022BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2022EXERCISE - Question
454 Videos
Similar Questions
Knowledge Check
To which of the following circles, the line y−x+3=0 is normal at the point (3+3/√2,3/√2)?
A(x−3−3/√2)2+(y−3/√2)2=9B(x−3/√2)2+(y−3/√2)2=9Cx2+(y−3)2=9D(x−3)2+y2=9The graph of straight line y=√3x+2√3 is :
A B
B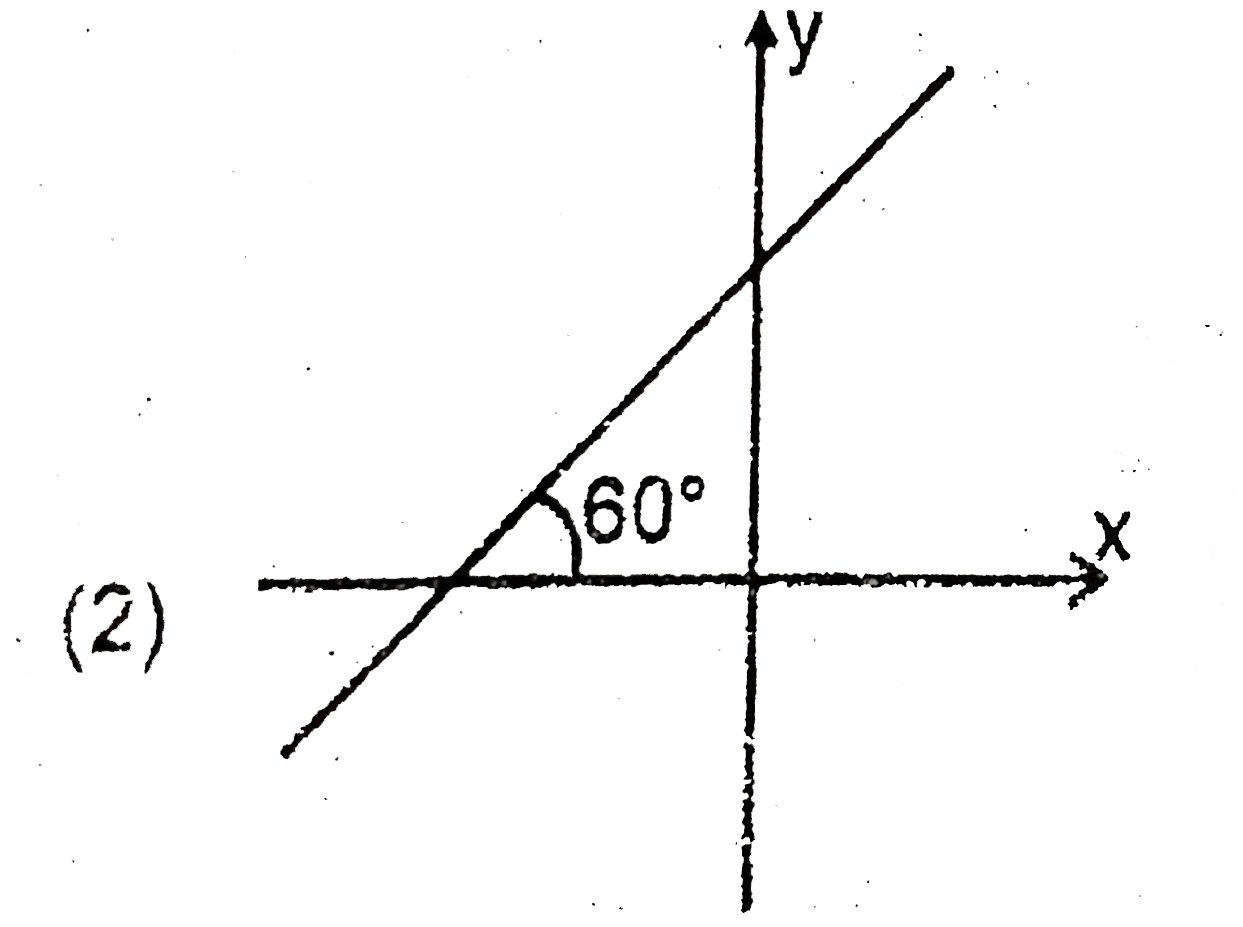 C
C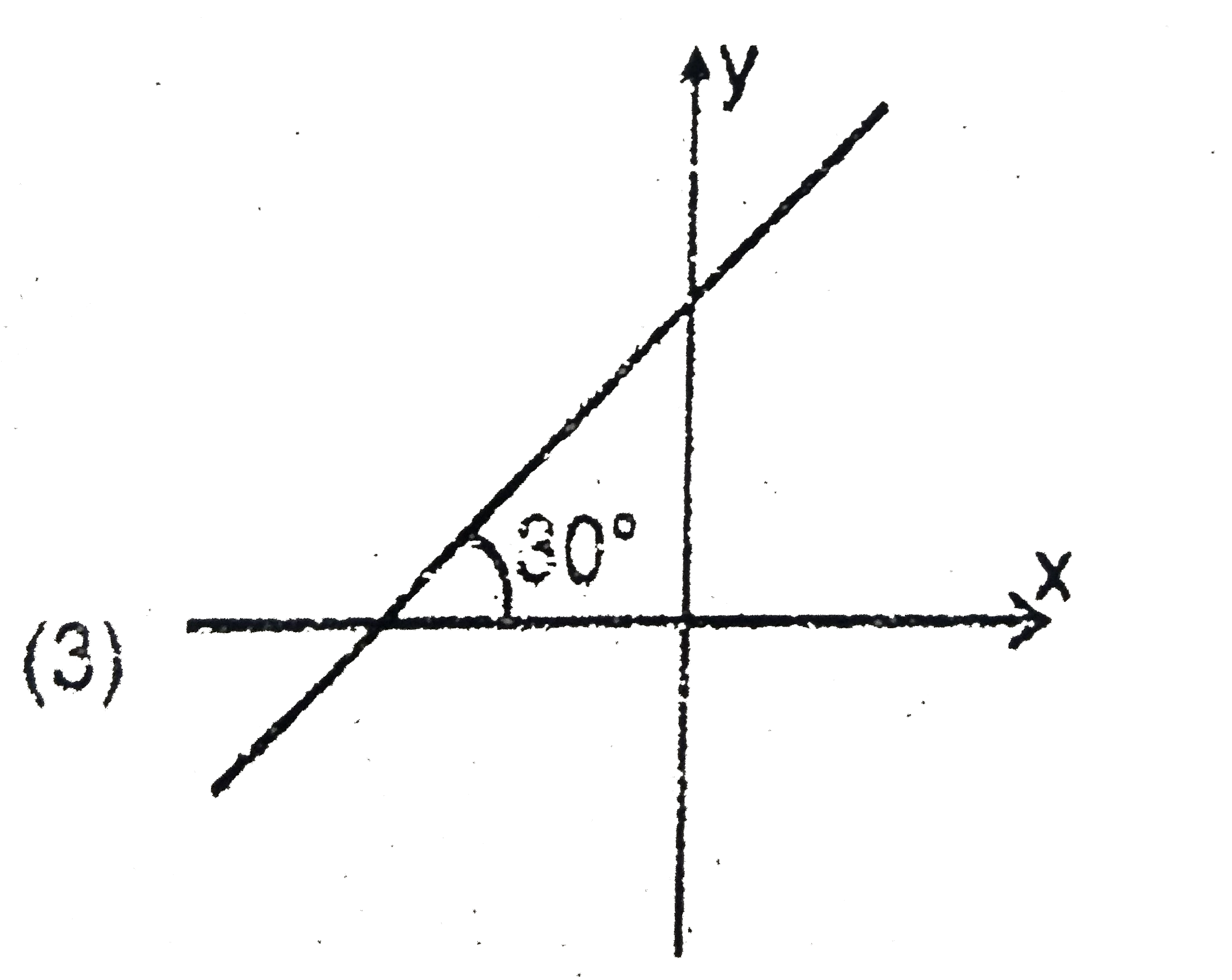 D
D
If x=2+√3,y=2−√3 then the value x2+y2x3+y3 is
A738B740C7/18D726
To which of the circles, the line y−x+3=0 is normal at the point (3+3√2,3√2) is
View SolutionThe coordinates (2, 3) and (1, 5) are the foci of an ellipse which passes through the origin. Then the equation of the (a)tangent at the origin is (3√2−5)x+(1−2√2)y=0 (b)tangent at the origin is (3√2+5)x+(1+2√2y)=0 (c)tangent at the origin is (3√2+5)x−(2√2+1)y=0 (d)tangent at the origin is (3√2−5)−y(1−2√2)=0
View SolutionFind the angle which the line joining the points (1,√3)and(√2,√6) makes with the x-axis.
View SolutionThe point at which the tangent to the curve y=√4x−3−10 has slope 23 is
View SolutionThe angle between the lines √3x−y−2=0andx−√3y+1=0 is
View Solution
