Question

Obtain the equation of speed of transverse wave on tensed (stretched) string.

Explanation

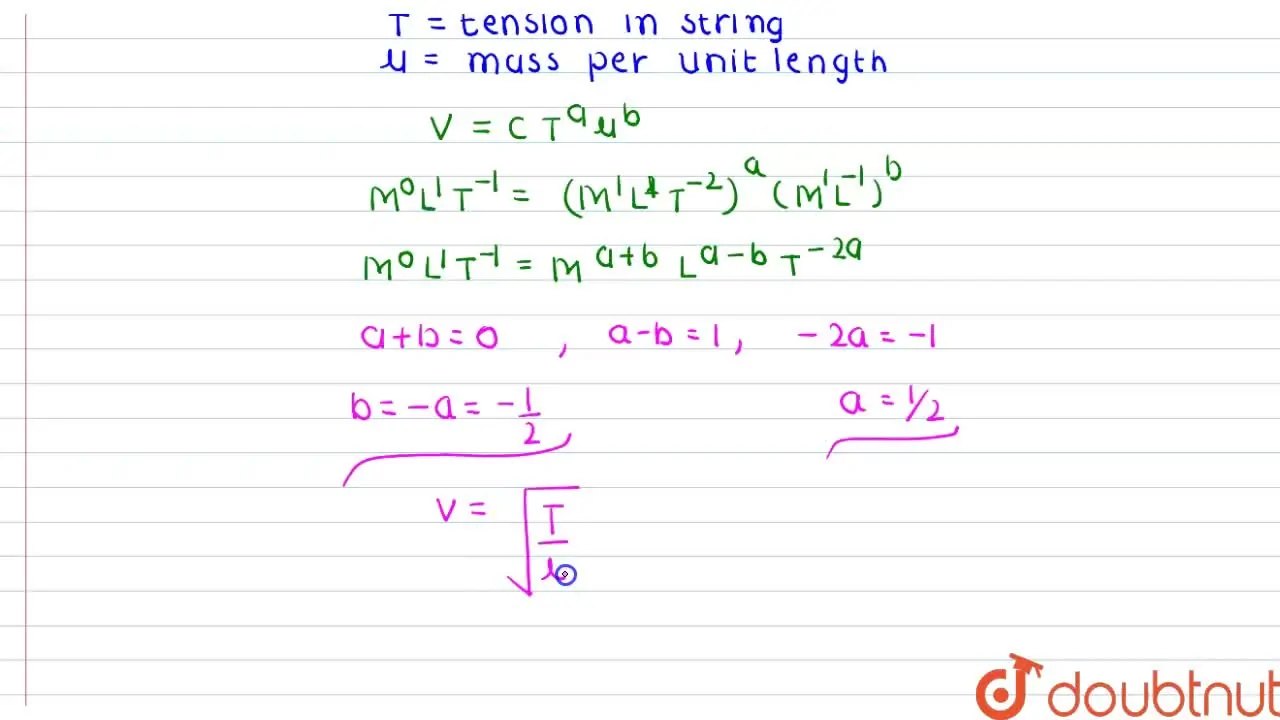
The speed of transverse waves on a string is determined by two factors, (i) the linear mass density or mass per unit length `mu` and (ii) the tension T.
The linear mass density, `mu` of a string is the mass m of the string divided by its length l. Therefore its dimension is `[M^(1) L ^(-1)].` The tension T has the dimension of force - namely `[M^(1) L ^(1) T ^(2)].`
We have to combine `mu` and T in such a way as to generate v [dimension `(LT ^(-1) )].`
It can be seen that tghe ratio `T/mu` has the dimension `[ L ^(2) T ^(-2)].`
`[(T)/(mu) ] = ([ M^(2) L ^(1) T ^(-2)])/( [ M ^(1) L ^(-1)]) = [L^(2) T ^(-2)]`
Therefore, if v depends only on T anad `mu,` the relation between them must be,
`v = C sqrt ((T)/(mu))`
Here C is a dimensionless constant and constnat C is indeed equal to unity.
The speed of transverse waves on the stretched string is therefore given by.
`v = sqrt ((T)/(mu))`
The speed of a wave along a stretched ideal string depends only on the tension and the linear mass density of the streing and does not depend on the frequency of the wave.