In a triangle ABC , if A is (2,−1),and7x−10y+1=0 and 3x−2y+5=0 are the equations of an altitude and an angle bisector, respectively, drawn from B , then the equation of BC is a+y+1=0 5x+y+17=0 4x+9y+30=0 (d) x−5y−7=0
The correct Answer is:4
Co-ordinate of B will be point of intersection of 7x−10y+1=0,3x−2y+5=0
(-3,-2)
Image of A in bisector of B lies on line BC.
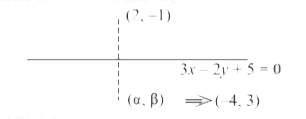
Similar Questions
In a triangle ABC , if A is (2,−1),and7x−10y+1=0 and 3x−2y+5=0 are the equations of an altitude and an angle bisector, respectively, drawn from B , then the equation of BC is (a) a+y+1=0 (b)5x+y+17=0 (c)4x+9y+30=0 (d) x−5y−7=0
View SolutionIn a triangle ABC , the bisectors of angles BandC lies along the lines x=yandy=0. If A is (1,2) , then the equation of line BC is (a) 2x+y=1 (b) 3x−y=5 (c) x−2y=3 (d) x+3y=5
View Solution
Knowledge Check
The straight lines 3x−4y+7=0 and 12x+5y−2=0 has equation of the acute angle bisector as:
A99x−27y−81=0B11x−3y+9=0C21x+77y−101=0D21x+77y+101=0x2+y2−4x−4y+7=0 and x2+y24x+4y+7=0 are equation of excircle opposite to B and C respectively in a ΔABC, then
AA=(0,0)BAB=ACCΔABCcan be equilateralDΔABCcan not be equilateralIn a triangle ABC , if the equation of sides AB,BC and CA are 2x−y+4=0,x−2y−1=0andx+3y−3=0 respectively ,
The equation of external bisector of angle B isAx−y−1=0Bx−y+1=0Cx+y−5=0Dx+y+5=0
The equation of the line AB is y=x. If A and B lie on the same side of the line mirror 2x−y=1, then the equation of the image of AB is (a) x+y−2=0 (b) 8x+y−9=0 (c) 7x−y−6=0 (d) None of these
View Solutionthe equation of perpendicular bisectors of side AB,BC of triangle ABC are x−y=5,x+2y=0 respectively and A(1,−2) then coordinate of C
View SolutionIn ΔABC equation of the right bisectors of the sides Ab and AC are x+y=0andx−y=0 respectively .If A = (5,7) then equation of side BC is
View SolutionGiven the four lines with the equations
x+2y−3=0, 3x+4y−7=0,
2x+3y−4=0, 4x+5y−6=0, then
View SolutionThe equations of the sides of a triangle are x+y-5=0, x-y+1=0, and y-1=0. Then the coordinates of the circumcenter are
View Solution
