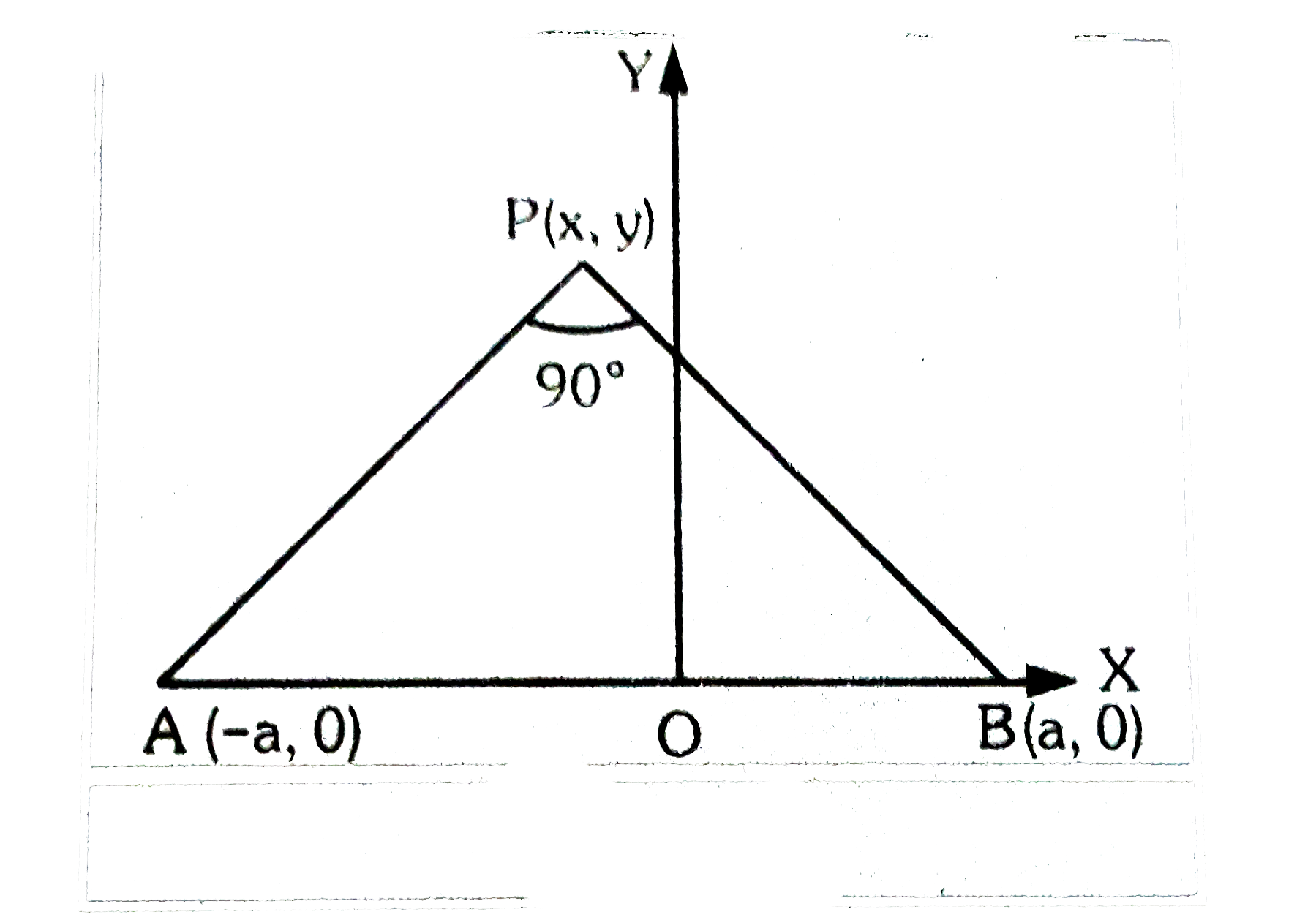
If A(a,0),B(−a,0) and ∠APB=45∘, then the locus of P is Solution in Telugu
x2+y2+2ax+a2=0
x2+y2−2ax−a2=0
x2+y2+2yx+a2=0
x2+y2−2ay−a2=0
The correct Answer is:4
Step by step video, text & image solution for If A(a,0), B(-a,0) and angleAPB=45^(@), then the locus of P is by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- LOCUSBOOK - AAKASH SERIESCHAPTER - LOCUSEXERCISE - PRACTICE EXERCISE
37 Videos
- LOCUSBOOK - AAKASH SERIESCHAPTER - LOCUSEXERCISE - ADDITIONAL EXERCISE
8 Videos
- LIMITSBOOK - AAKASH SERIESCHAPTER - LIMITSEXERCISE - PRACTICE EXERCISE
143 Videos
- MATHEMATICAL INDUCTIONBOOK - AAKASH SERIESCHAPTER - MATHEMATICAL INDUCTIONEXERCISE - PRACTICE SHEET (EXERCISE-I) LEVEL-I (Principle of Mathematical Induction) (Straight Objective Type Questions)
55 Videos
Similar Questions
The locus of the point which is equidistant to the coordinate axes is
View SolutionFind the locus of the point (x,y) where
x=a+bcosθ,y=b+asinθ
View SolutionLtx→0x2−5x+72x2+3x−1=
View SolutionLet P(n) denote the statement that n2+n is odd. It is seen that P(n)⇒P(n+1),P(n) is true for all
View SolutionIf A(a,0),B(-a,0) and /_APB=45^(@) then the locus of P is
View Solutionमान लीजिए A(0,a) तथा B(0,b) दो स्थिर बिंदु है तथा P(x,0) एक चर बिंदु है । तब ∠APB अधिकतम होगा जबकि x2 बराबर होगा -
View Solutionमाना A तथा B दो स्थिर बिन्दु है | ऐसे बिन्दु P का बिन्दुपथ ज्ञात कीजिए जिससे ∠APB समकोण बनता है |
View SolutionA(−9,0),B(−1,0) are two points. If P is a point such that PA:PB=3:1 , then the locus of P is
View SolutionO(0,0),A(6,0),B(0,4) are three points. If P is a point such that the area of the quadrilateral PABC is 10 sq. Unit, then the locus of P is
View SolutionA and B are two fixed points. The locus of a point P such that ∠APB is a right angle, is:

View SolutionIf A and B are mutually exclusive events such that P(A)=0.35 and P(B)=0.45 , find P(A∪B)
View SolutionA (a,0) , B (-a,0) are two points . If a point P moves such that ∠PAB−∠PBA=π/2 then find the locus of P.
View SolutionA(a,0) , B(-a,0) are two point . If a point P moves such that ∠PAB−∠PBA=2α then find the locus of P .
View SolutionA=(a,0),B=(−a,0), P is a moving point such that ∠PAB−∠PBA=π2. The locus of P is
View SolutionA(−9,0) and B(−1,0) are two points. If P(x,y) is a point such that 3PB=PA, then the locus of P is
View Solution
