माना हो, तो -
h वर्धमान होगा यदि वर्धमान हो।
h वर्धमान होगा, यदि ओसमान हो।
h ओसमान है, यदि ओसमान हो।
सामान्य रूप से कुछ भी नहीं कहा सकता है।
The correct Answer is:A, C
Step by step video, text & image solution for माना h(x) = f(x) - (f(x))^(2) + (f(x))^(3) , AA x in R हो, तो - by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MONOTONOCITYBook:ALLEN HINDIChapter:MONOTONOCITYExercise:EXERCISE - 02 (सही विकल्पों को चुनिए (एक या एक से अधिक सही उत्तर हैं))
Explore 16 Videos
- MONOTONOCITYBook:ALLEN HINDIChapter:MONOTONOCITYExercise:EXERCISE - 03 MISCELLANEOUS TYPE QUESTIONS ( स्तम्भ सुमेलन (Match the column) )
Explore 1 Video
- MONOTONOCITYBook:ALLEN HINDIChapter:MONOTONOCITYExercise:EXERCISE - 01 (सही विकल्प चुनिए (केवल एक सही उत्तर है))
Explore 20 Videos
- METHODS OF DIFFERENTIATIONBook:ALLEN HINDIChapter:METHODS OF DIFFERENTIATIONExercise:EXERCISE - 05 [B]
Explore 12 Videos
- PARABOLA Book:ALLEN HINDIChapter:PARABOLA Exercise:DO YOURSELF - 7
Explore 2 Videos
Similar Questions
एक अवकलनीय फलन f(x),∀x∈R, एकदिष्ट वर्धमान है, तब -
View Solutionनिम्न प्रश्न में दो स्तम्भ में वक्तव्य (statements) दिये हुए हैं जिनका सुमेल (match) करना है।स्तम्भ-I (Column-I) के वक्तव्यों को A,B,C तथा D नामित किया गया है जबकि स्तम्भ-II (Column-II) के वक्तव्यों को p,q,r तथा s नामित किया गया है। स्तम्भ-I (Column-I) में दिए गए कोई एक वक्तव्यस्तम्भ-II (Column-II) के एक या एक से अधिक वक्तव्य (वक्तव्यों) से सही सुमेल करता है।
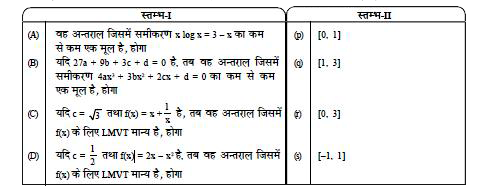
View Solutionफलन f(x)=x2(x−2)2 है I
View Solutionयदि ln (x+y) = 2xy हो , तो y'(0) =
View Solutionरेखाओं x=4,y=4 तथा निर्देशी अक्षो से बने वर्गीय क्षेत्र को परवलय y2=4x तथा x2=4y विभाजित करते है। यदि S1,S2,S3 क्रमशः इन भागो के क्षेत्रफल ऊपर से नीचे की ओर अंकित है, तो S1:S2:S3 ज्ञात कीजिए।
View SolutionF(x)=min{x,x^(2)},AA in R then f(x) is
View SolutionSuppose f'(x) exists for each x and h(x)=f(x)−(f(x))2+(f(x))3∀x∈R, then
View SolutionLet f(x) be a continuous function, ∀x∈R,f(0)=1andf(x)≠x for any x∈R, then show f(f(x))>x,∀x∈R+
View SolutionIf f(x) is a real valued function such that
f(x+6)−f(x+3)+f(x)=0,∀x∈R, then period of f(x) is
View Solutionयदि f:R→R तथा f(x)=x2+2,∀x∈R तो सिद्ध कीजिये कि f बहु - एक अन्तःक्षेपी है।
View SolutionConsider the function f(x)=(x3−x)∣∣x2−6x+5∣∣,∀x∈R, then f(x) is
View Solutionमाना कि f:R→R फलन f(x)=√x−3,∀x∈R द्वारा परिभाषित है, तब f−1(x)⇒
View Solutionमाना f(x+y)+f(x-y)=2f (x)f(y) , AA x , y in R And f(0) ne 0 , Then the function is f (x)
View Solutionमाना f(x+y)+f(x-y)=2f (x)f(y) , AA x , y in R And f(0) ne 0 , Then the value of f (2) is if f (1) = k
View Solutionमाना f(x+y)+f(x-y)=2f (x)f(y) , AA x , y in R And f(0) ne 0 , Then if f (1) = k then f (3) - 2kf (2) is the value of
View Solution
