Question

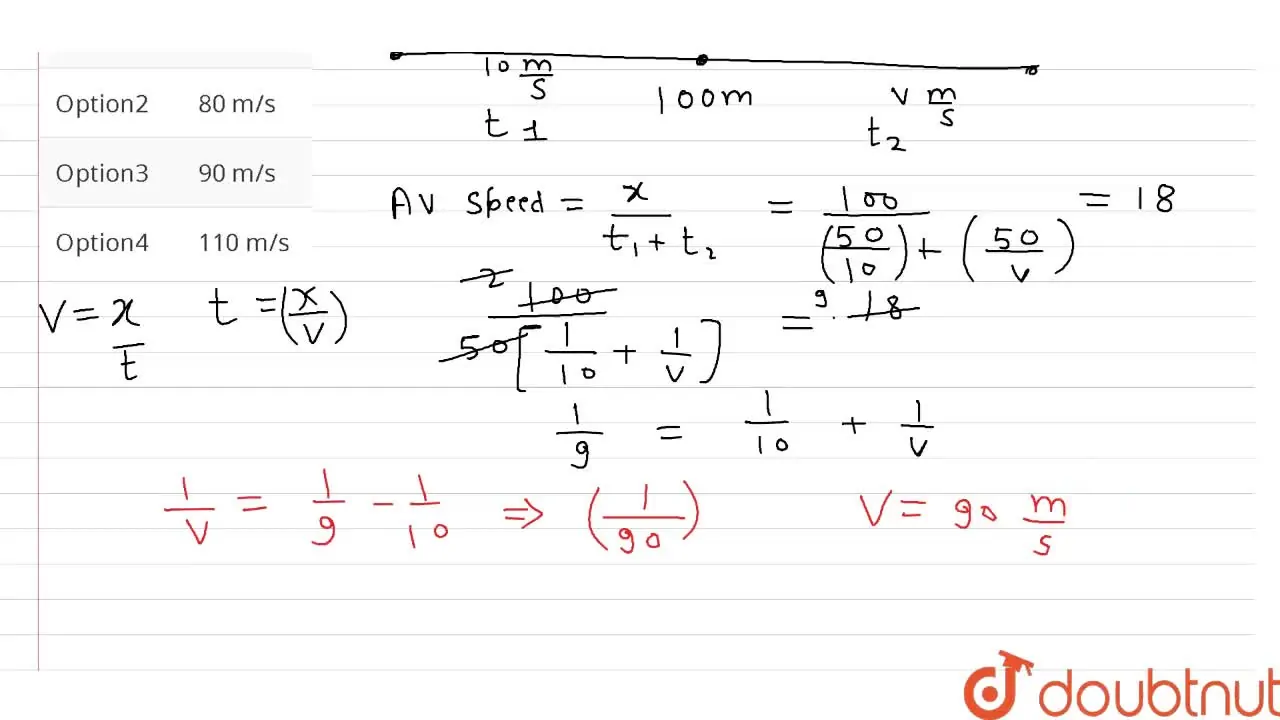
A car moves a distance of 100 m. It coveres first half with speed of 10 m/s and another half with speed of v m/s. If the average speed of car is 18 m/s, then value of v is

Options

A. 60 m/s
B. 80 m/s
C. 90 m/s
D. 110 m/s

Answer

C

Explanation

To solve the problem step by step, we need to find the value of $$v$$ given the conditions of the car's movement.

Step 1: Understand the problem
The car travels a total distance of 100 m. It covers the first half (50 m) at a speed of 10 m/s and the second half (50 m) at an unknown speed \( v \) m/s. The average speed of the car is given as 18 m/s.

Step 2: Calculate the time taken for each half
1. Time for the first half (t1):
\( t_1 = \frac{\text{Distance}}{\text{Speed}} = \frac{50 \text{ m}}{10 \text{ m/s}} = 5 \text{ s} \)

2. Time for the second half (t2):
\( t_2 = \frac{\text{Distance}}{\text{Speed}} = \frac{50 \text{ m}}{v \text{ m/s}} = \frac{50}{v} \text{ s} \)

Step 3: Write the equation for average speed
The average speed (\( v_{avg} \)) is defined as the total distance divided by the total time:
\( v_{avg} = \frac{\text{Total Distance}}{\text{Total Time}} = \frac{100 \text{ m}}{t_1 + t_2} \)
Given that the average speed is 18 m/s, we can write:
\( 18 = \frac{100}{t_1 + t_2} \)

Step 4: Substitute the time values into the average speed equation
Substituting \( t_1 \) and \( t_2 \):
\( 18 = \frac{100}{5 + \frac{50}{v}} \)

Step 5: Solve for \( v \)
1. Rearranging the equation gives:
\( 5 + \frac{50}{v} = \frac{100}{18} \)
Simplifying \( \frac{100}{18} \):
\( \frac{100}{18} = \frac{50}{9} \)
Thus:
\( 5 + \frac{50}{v} = \frac{50}{9} \)

2. Subtract 5 from both sides:
\( \frac{50}{v} = \frac{50}{9} - 5 \)
Converting 5 to a fraction with a denominator of 9:
\( 5 = \frac{45}{9} \)
Therefore:
\( \frac{50}{v} = \frac{50}{9} - \frac{45}{9} = \frac{5}{9} \)

3. Cross-multiplying gives:
\( 50 \cdot 9 = 5v \)
Simplifying:
\( 450 = 5v \)
Dividing by 5:
\( v = \frac{450}{5} = 90 \text{ m/s} \)

Final Answer
The value of \( v \) is 90 m/s.

---