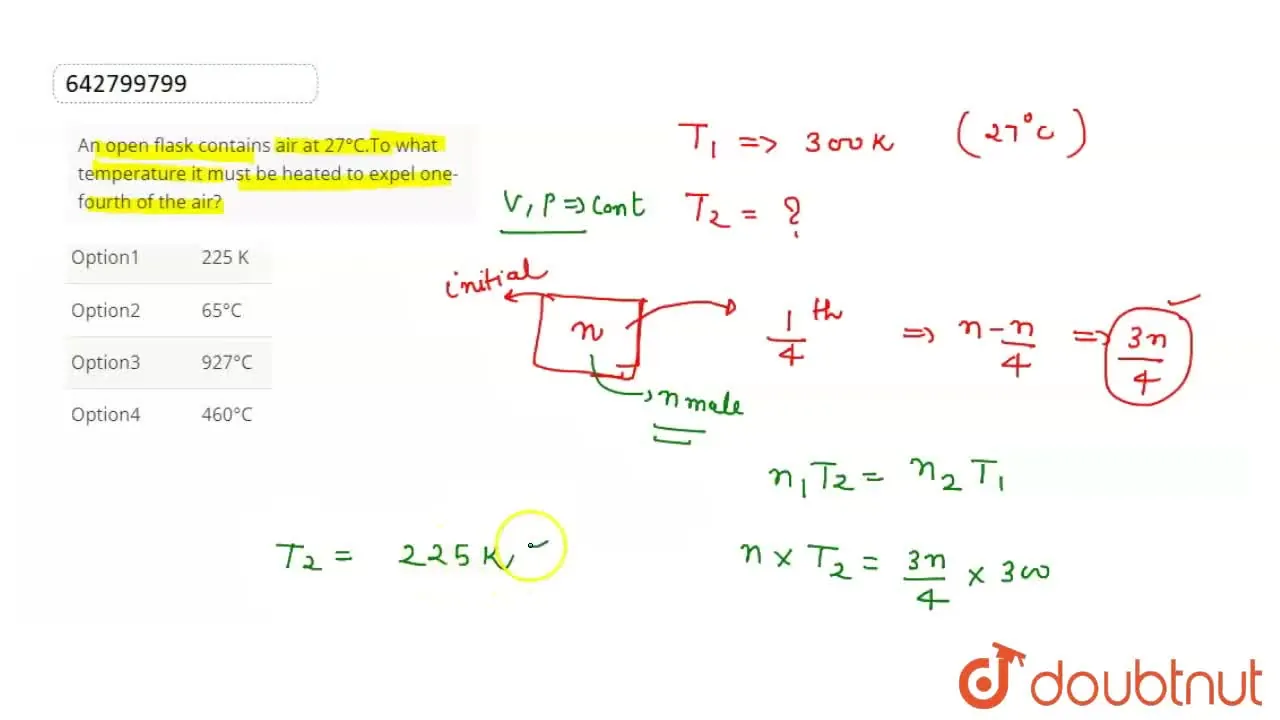
An open flask contains air at 27°C.To what temperature it must be heated to expel one-fourth of the air?
225 K
65°C
927°C
460°C
The correct Answer is:A
Step by step video, text & image solution for An open flask contains air at 27°C.To what temperature it must be heated to expel one-fourth of the air? by Chemistry experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
Similar Questions
An open flask contains air at 27∘C Calculate the temperature at which it should be heated so that
(a) 13 rd of air measured at 27∘C escapes out
(b) 13 rd of air measured at final temperature escapes out .View SolutionAn open flask contains air at 27∘C, calculate the temperature to which it should be heated such that one third of air measured at final temperature escapes out.
View SolutionA vessel with open mouth contains air at 60∘C. When the vessel is heated upto temperature T, one fourth of the air goes out. The value of T is
View SolutionAn open flask contains air at 27∘C. Calculate the fraction of air that would be expelled out, at 477∘C.
View SolutionAn open flask contains air at 27∘ C and one atm. Pressure. The flask is heated to 127∘ C at the same pressure . The fraction of original air remaining in the flask will be
View SolutionAn open vessel contains200 mg of air at 17∘C. What weight percent of air would be expelled if the vessel is heated to 117∘C ?
View SolutionA 1 L flask contains 32 g O2 gas at 27∘C. What mass of O2 must be released to reduce the pressure in the flask to 12.315 atm?
View SolutionA steel tank containing air at 15 pressure at 15∘C is provided with a safety valve that will yield at a pressure of 30 atm. To what minimum temperature must the air be heated to blow the safety valve ?
View SolutionAn open bulb containing air at 19∘C was cooled to a certain temperature at which the number of moles of the gaseous molecules increased by 25%. What is the final temperature ?
View SolutionA two litre glass flask contains some mercury. It is found that at all temperatures the volume of the air inside the flask remains the same. The volume of the mercury inside the flask is (αfor glass =9×10−6/∘C,γ for mercury 1.8×10−4/∘C)
View Solution