The resultant of the three vectors $- - \to O A, - - \to O B$ and $- - \to O C$ shown in figure is

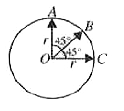
$r (1 + \sqrt{s})$

$r (\sqrt{2} - 1)$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

Updated on:21/07/2023

What is the angle between the vector $\to A$ and $\to B$ as shown in figure ?

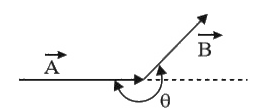A $θ$
B $π - θ$
C $θ - π$
D $2 π - θ$
The resultant of two vectors $\to P$ and $\to Q$ is $\to R$ . If the magnitude of $\to Q$ is doubled, the new resultant vector becomes perpendicular to $\to P$ . Then, the magnitude of $\to R$ is equal to
A $P + Q$
BP
C $P - Q$
DQ
The resultant of two vectors $\to A$ and $\to B$ is perpendicular to the vector $\to A$ and its magnitudes is equal to half of the magnitudes of vector $\to B$ (figure). The angle between $\to A$ and $\to B$ is

A $120^{\circ}$
B $150^{\circ}$
C $135^{\circ}$
DNone of these