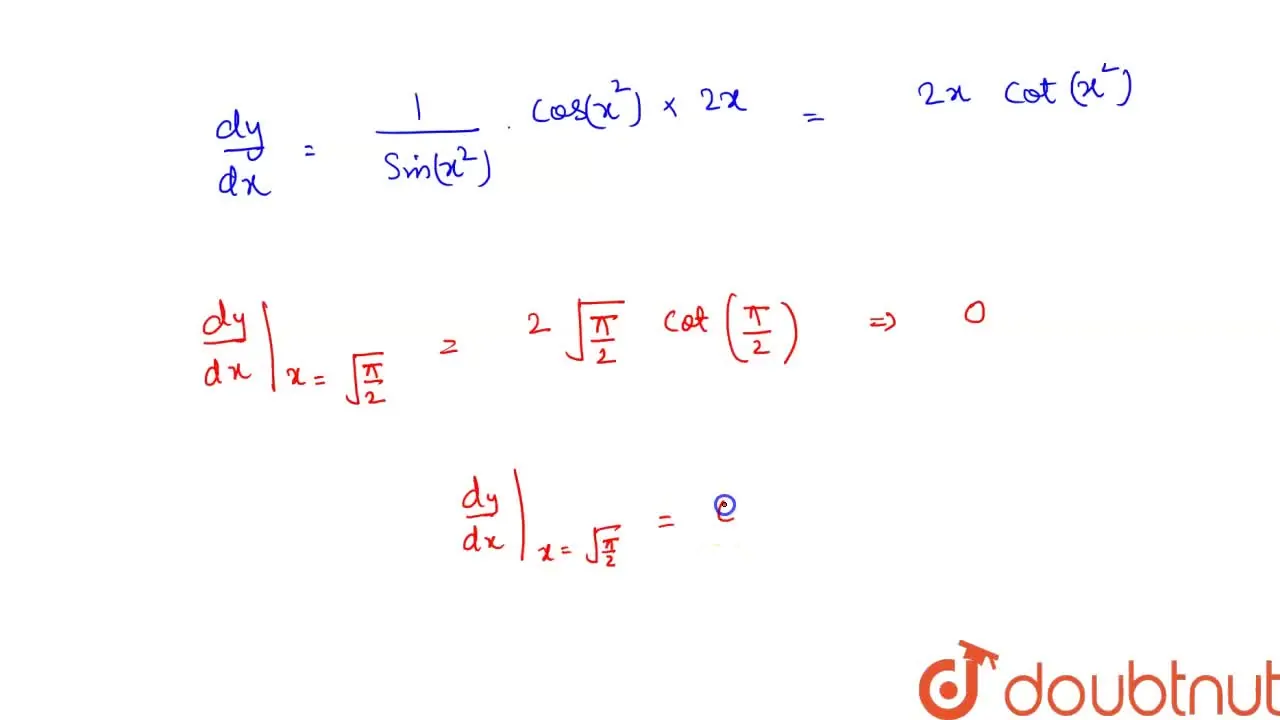
Let y=logesin(x2),0<x≤π2. The value of dydx at x=√π2 is
0
1
π4
√π
The correct Answer is:A
Step by step video & image solution for Let y =log_(e)sin(x^2),0ltxlepi/2. The value of dy/dx at x= sqrt(pi/2) is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - A DAS GUPTACHAPTER - DifferentiationEXERCISE - Exercise98videos
Differentiation
- BOOK - A DAS GUPTACHAPTER - Differential Equation of the First OrderEXERCISE - Exercise64videos
Differential Equation of the First Order
- BOOK - A DAS GUPTACHAPTER - Elementary ProbabilityEXERCISE - Exercise137videos
Elementary Probability
Similar Questions
If y=esinx ,the value of dydx at x=π2 is
View SolutionIf y=log(sin(x2)),0<x<π2,then dydxat x =√π2 is
View SolutionIf y=∫x0√sinxdx the value of dydx at x=π2 is :
View SolutionIf y=log√tanx , then the value of dydx at x=π4 is given by (a)∞ (b) 1 (c) 0 (d) 12
View SolutionIf y=tan−1√1−sinx1+sinx, then the vluae of dydx at x=π2 is
View SolutionIf y =sin^(-1) ((sqrt(1+x)+sqrt(1-x))/2),Thenthevalueof(dy)/(dx) at x=0 is
View SolutionIf y=sin−1(√1+x+√1−x2), Then the value of dydx at x=0 is
View SolutionIf y =sin^(-1) ((sqrt(1+x)+sqrt(1-x))/2),Thenthevalueof(dy)/(dx) at x=0 is
View SolutionIf y=sin−1(√1+x+√1−x2), Then the value of dydx at x=0 is
View SolutionIf y=log(ex2), then dydx=?
View Solution