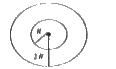
A soap bubble of radius R is surrounded by another soap bubble of radius 2R, as shown. Take surface tension = S.
 Solution in Telugu
Solution in Telugu
Then the pressure inside the smaller soap bubble in excess of the atmospheric pressure is zero
Then the pressure inside the smaller soap bubble in excess of the atmospheric pressure is 6S/R
Then the pressure inside the larger soap bubble in excess of the atmospheric pressure is 4S/2R
Then the pressure inside the larger soap bubble in excess of the atmospheric pressure is 4S/R
The correct Answer is:B, C
Step by step video, text & image solution for A soap bubble of radius R is surrounded by another soap bubble of radius 2R, as shown. Take surface tension = S. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SURFACE TENSIONBOOK - AAKASH SERIESCHAPTER - SURFACE TENSIONEXERCISE - LECTURE SHEET (EXERCISE-II ( LINKED COMPREHENSION TYPE QUESTIONS))
3 Videos
- SURFACE TENSIONBOOK - AAKASH SERIESCHAPTER - SURFACE TENSIONEXERCISE - LECTURE SHEET (EXERCISE-II ( MATRIX MATCHING TYPE QUESTIONS))
1 Videos
- SURFACE TENSIONBOOK - AAKASH SERIESCHAPTER - SURFACE TENSIONEXERCISE - LECTURE SHEET (EXERCISE-II (STRAIGHT OBJECTIVE TYPE QUESTIONS))
8 Videos
- SIMPLE HARMONIC MOTION BOOK - AAKASH SERIESCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL - II)(PRACTICE SHEET (ADVANCED)) (INTEGER TYPE QUESTIONS)
3 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH SERIESCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - PRACTICE EXERCISE
99 Videos
Similar Questions
When small droplets floating in atmosphere merge into each other in clouds, they become heavy and cannot float in air and fall down as rain drops. In this process of formation of bigger drops if 'n' small drops each of radius 'r', surface tension “T” coalesce. Then
If P is the excess pressure in a smaller drop, the excess pressure in bigger drop is
View SolutionMatch the following

View SolutionA liquid rises to a height of 50 cm in a capillary tube of diameter 0.04mm. If density of liquid is 0.08×103kgm−3 angle of contact is 20°, the surface tension of the liquid is (cos20∘=0.94)
View SolutionConsider the motion of a ball in a tunnel through the earth (radius of the earth is R and mass of the earth is M), the tunnel is along a chord and the ball is released from the surface. The time period of the ball is √x (approx), find x.
View SolutionThe centre of mass of two particles with masses 2kg and 6 kg located at (1,1,1), (2,2,1) respectively has the coordinates
View SolutionA soap bubble of raidus R is surrounded by another soap bubble of radius 2R , as shown. Take surface tension =S . Then the pressure inside the smaller soap bubble, in excess of the atmosphere presure will be
View SolutionThe radius of a soap bubble is r. the surface tension of soap solution is T, keeping temperature constant, the radius of the soap bubble is doubled the energy necessary for this will be-
View SolutionA soap bubble of radius r is placed on another bubble of radius 2r . The radius of the surface common to both the bubbles is
View Solutionसाबुन के एक बुलबुले में हवा डालकर इसकी त्रिज्या r से बढ़ाकर 2r कर दी जाती है | यदि पृष्ठ-तनाव S हो तो इस प्रक्रिया में किया गया कार्य होगा
View SolutionWork done in forming a soap bubble of radius R is W. Then workdone is forming a soap bubble of radius '2R' will be :
View SolutionWhat will be the work done in increasing the radius of soap bubble from r/2 to 2r, if surface tension of soap solution is T?
View SolutionThe excess pressure inside a soap bubble of radius R is (S is the surface tension)
View SolutionRadius of soap bubble is r. The surface tension of soap solution is T. Keeping temperature constant, the radius of soap bubble is doubled. The energy necessary for this will be....
View SolutionThe radius of soap bubble is 'r' and the surface tension of soap solution is S, the energy required to double the radius is
View SolutionA soap bubble of radius r is placed on another bubble of radius 2r . The radius of the surface common to both the bubbles is
View Solution
