The reaction ,2AB(g)+2C(g)→A2(g)+2BC(g) proceeds according to the mechanism .
I. 2AB⇔A2B2 (fast)
II. A2B2+C→A2B+BC (slow )
III. A2B+C→A2+BC (fast)
what will be the initial rate taking [AB] = 0.2 M and [C] = 0.5 M ? The Kc for the step I is 102M−1 and rate constant for the step II is 3.0×10−3mol−1−1min
0.0716M−1min
0.0891M−1min
0.006M−1min
0.0257M−1min
The correct Answer is:C
To solve the given problem, we will follow these steps:
Step 1: Identify the rate-determining step
The rate of a reaction mechanism is determined by the slowest step, which is step II in this case. Therefore, we will focus on this step to find the rate of the reaction.
Step 2: Write the rate law for the slow step
The rate law for step II can be expressed as:
Rate=k⋅(A2B2)⋅(C)
where k is the rate constant for step II.
Step 3: Determine the concentration of A2B2
Since step I is an equilibrium step, we can use the equilibrium constant Kc to relate the concentrations of the species involved. The equilibrium constant for step I is given by:
Kc=(A2B2)(AB)2
From this, we can express the concentration of A2B2:
(A2B2)=Kc⋅(AB)2
Step 4: Substitute the values into the equation
Now we can substitute the values provided in the question:
- Kc=102M−1
- (AB)=0.2M
- (C)=0.5M
- k=3.0×10−3mol−1min−1
Calculating (A2B2):
(A2B2)=102⋅(0.2)2=100⋅0.04=4M
Step 5: Calculate the rate of the reaction
Now we can substitute (A2B2) into the rate law:
Rate=k⋅(A2B2)⋅(C)
Rate=(3.0×10−3)⋅(4)⋅(0.5)
Rate=(3.0×10−3)⋅2=6.0×10−3molmin−1
Final Answer
The initial rate of the reaction is:
Rate=0.006molmin−1
The rate of a reaction mechanism is determined by the slowest step, which is step II in this case. Therefore, we will focus on this step to find the rate of the reaction.
The rate law for step II can be expressed as:
Rate=k⋅(A2B2)⋅(C)
where k is the rate constant for step II.
Since step I is an equilibrium step, we can use the equilibrium constant Kc to relate the concentrations of the species involved. The equilibrium constant for step I is given by:
Kc=(A2B2)(AB)2
From this, we can express the concentration of A2B2:
(A2B2)=Kc⋅(AB)2
Now we can substitute the values provided in the question:
- Kc=102M−1
- (AB)=0.2M
- (C)=0.5M
- k=3.0×10−3mol−1min−1
(A2B2)=102⋅(0.2)2=100⋅0.04=4M
Now we can substitute (A2B2) into the rate law:
Rate=k⋅(A2B2)⋅(C)
Rate=(3.0×10−3)⋅(4)⋅(0.5)
Rate=(3.0×10−3)⋅2=6.0×10−3molmin−1
The initial rate of the reaction is:
Rate=0.006molmin−1
Topper's Solved these Questions
- PRACTICE PAPER -2BOOK - NCERT FINGERTPS ENGLISHCHAPTER - PRACTICE PAPER -2EXERCISE - Practice Paper 2
50 Videos
- PRACTICE PAPER -1BOOK - NCERT FINGERTPS ENGLISHCHAPTER - PRACTICE PAPER -1EXERCISE - Practice Paper 1
50 Videos
- PRACTICE PAPER -3BOOK - NCERT FINGERTPS ENGLISHCHAPTER - PRACTICE PAPER -3EXERCISE - Practice Paper 3
50 Videos
Similar Questions
Knowledge Check
A hypothetical reaction A2+B2→2AB follows the mechanism as given below:
A2⇔A+A(fast)
A+B2→AB+B (slow)
A+B→AB (fast)
The order of the overall reaction isA2B1C1.5Dzero
The yield of product in the reaction,
A2(g)+2B(g)⇔C(g)+QKJ
would be higher at:
View SolutionIf 50 ml of A2B3 reacts completely with 200 ml of C2 in closed vessel according to the equation , 2A2B3(g)+5C2(g)→3C3B2(g)+CA4(g) . The composition of the mixture of gases is :
View SolutionThe equilibrium constant for the reaction
A2(g)+B2(g)⇔2AB(g)
is 20 at 500K. The equilibrium constant for the reaction 2AB(g)⇔A2(g)+B2(g) would be
View SolutionThe mechanism for the overall reaction is
A2+B→C
A2→2A (slow)
2A+B→X (fast)
If a catalyst D changes the mechanism to
A2+D→A2D (slow)
A2D⇒2A+D (fast)
2A+B→C (fast)
Which is the rate expresison for the reaction with and without a catalyst ?
View SolutionLet A={a1,b1,c1} and B={a2,b2}
(i) A×B
(ii) B×B
View Solution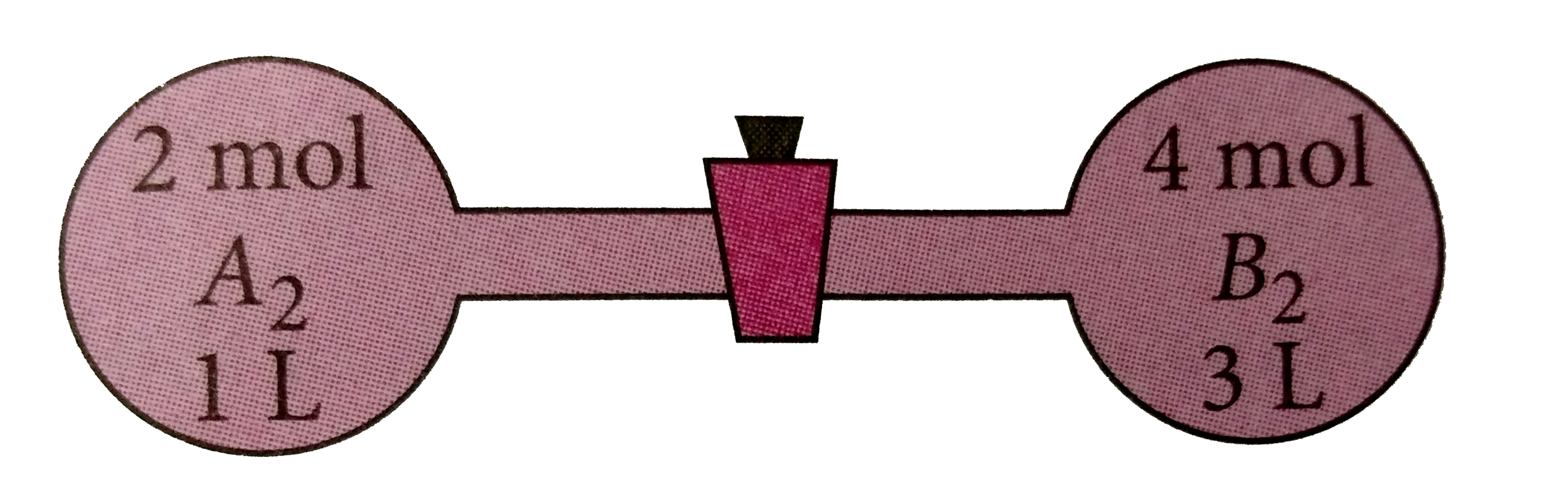
When A2andB2 are allowed to react, the equilibrium constant of the reaction at 27∘C is found (Kc=4).
A2(g)+B2(g)⇔2AB(g)
what will be the equilibrium concentration of AB?
View SolutionMechanism of the reaction is:
A2k2⇐⇒2A
A+Bk2−−→C
A2+Ck−−→D+A
What is d[D]dt ?
View Solution
